10 Is What Percent Of 60
Kalali
Apr 17, 2025 · 5 min read
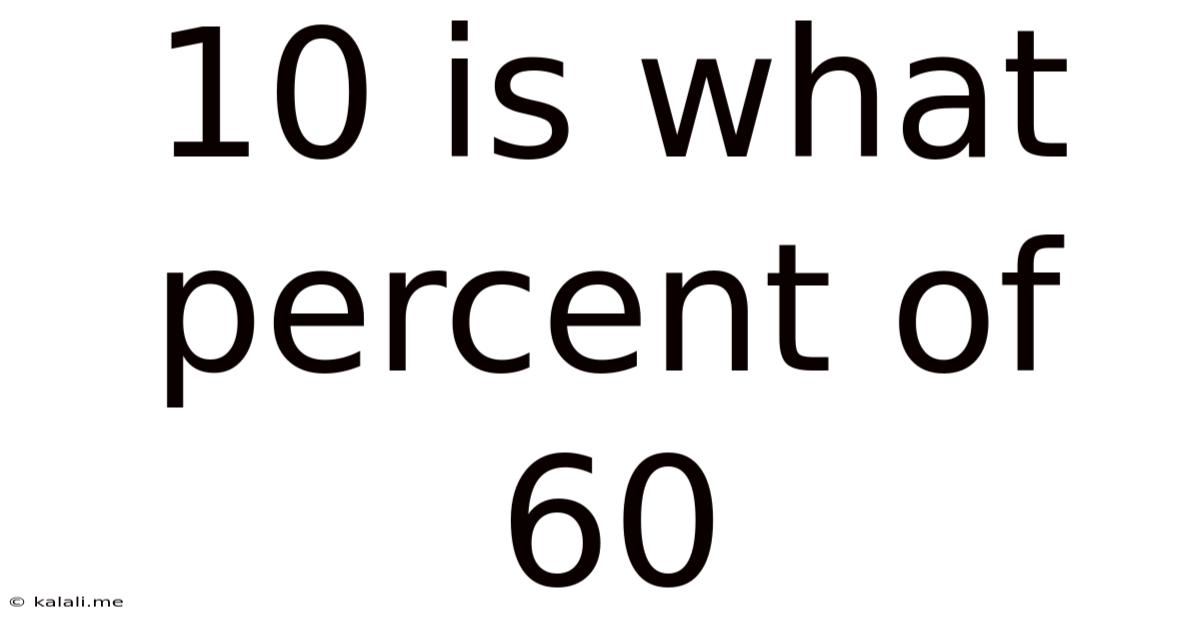
Table of Contents
10 is What Percent of 60? A Deep Dive into Percentages and Their Applications
This seemingly simple question, "10 is what percent of 60?", opens the door to a vast world of percentage calculations, their practical applications, and the underlying mathematical principles. While the answer itself is straightforward, understanding the process and its broader implications is crucial for various aspects of life, from everyday budgeting to complex financial analysis. This article will not only provide the solution but also delve into the mechanics of percentage calculations, explore different methods for solving similar problems, and demonstrate its relevance across diverse fields.
Meta Description: Learn how to calculate percentages with a comprehensive guide solving "10 is what percent of 60?". Discover various methods, real-world applications, and master percentage calculations for everyday use.
Understanding Percentages: The Foundation
A percentage is a way of expressing a number as a fraction of 100. The term "percent" literally means "per hundred" (from the Latin per centum). Therefore, 10% means 10 out of 100, or 10/100, which simplifies to 1/10. Understanding this fundamental concept is essential for all percentage-related calculations.
Solving "10 is What Percent of 60?"
There are several methods to determine what percentage 10 represents of 60. Let's explore the most common approaches:
Method 1: Using the Formula
The most direct method involves using the basic percentage formula:
(Part / Whole) x 100 = Percentage
In this case:
- Part: 10
- Whole: 60
Therefore:
(10 / 60) x 100 = 16.67% (approximately)
Therefore, 10 is approximately 16.67% of 60.
Method 2: Finding the Equivalent Fraction
We can express the relationship between 10 and 60 as a fraction: 10/60. To convert this fraction into a percentage, we simplify the fraction and then multiply by 100:
10/60 simplifies to 1/6.
To convert 1/6 to a percentage:
(1/6) x 100 ≈ 16.67%
Method 3: Using Proportions
Proportions offer another effective way to solve this problem. We can set up a proportion:
10/60 = x/100
Where 'x' represents the percentage we're trying to find. Solving for 'x':
60x = 1000 x = 1000/60 x ≈ 16.67
Practical Applications of Percentage Calculations
The ability to calculate percentages is invaluable in numerous real-world scenarios:
-
Finance: Calculating interest rates, discounts, tax rates, profit margins, and investment returns all rely heavily on percentage calculations. For example, understanding the percentage increase or decrease in your investment portfolio is crucial for effective financial planning. Similarly, calculating the interest on a loan or the discount on a sale requires proficiency in percentage calculations.
-
Sales and Marketing: Businesses use percentages extensively for sales analysis, tracking conversion rates, measuring marketing campaign effectiveness, and setting pricing strategies. Understanding market share and customer acquisition costs often involves percentage calculations. Analyzing the percentage change in sales figures from one period to the next is vital for business decision-making. Discount percentages are crucial in attracting customers and boosting sales.
-
Science and Statistics: Percentages are fundamental in scientific research and statistical analysis. Expressing experimental results, calculating statistical significance, and representing data visually often involves the use of percentages. For instance, scientists might express the percentage of a population affected by a disease or the percentage of successful trials in an experiment.
-
Everyday Life: Calculating tips in restaurants, understanding sales tax, figuring out discounts on purchases, and even determining the percentage of a recipe ingredient all involve percentage calculations. These skills are valuable in managing personal finances and making informed decisions in daily life. For example, accurately calculating the tip amount at a restaurant requires a quick understanding of percentages.
Advanced Percentage Calculations: Going Beyond the Basics
While the example of "10 is what percent of 60?" is relatively simple, percentage calculations can become more complex. Here are some advanced scenarios:
- Percentage Increase/Decrease: Calculating the percentage change between two values requires understanding the formula:
[(New Value - Old Value) / Old Value] x 100 = Percentage Change
For example, if a product's price increased from $50 to $60, the percentage increase is:
[(60 - 50) / 50] x 100 = 20%
-
Compound Interest: This involves calculating interest on both the principal amount and accumulated interest. Compound interest calculations are essential for understanding long-term investments and loan repayments.
-
Percentage Points vs. Percentages: It’s crucial to differentiate between percentage points and percentages. A change of 10 percentage points is different from a 10% increase. For example, if interest rates rise from 5% to 15%, that’s a 10-percentage point increase, but a 200% percentage increase.
-
Working with Multiple Percentages: Many real-world scenarios involve applying multiple percentages successively, such as discounts combined with taxes. These calculations require careful attention to the order of operations and understanding how percentages interact.
Mastering Percentage Calculations: Tips and Tricks
Here are some tips for improving your proficiency in percentage calculations:
-
Practice Regularly: The more you practice, the more comfortable you'll become with the different methods and formulas.
-
Use a Calculator: While mental calculations are beneficial, a calculator can be invaluable, especially for more complex problems.
-
Understand the Concepts: Focus on understanding the underlying principles of percentages rather than simply memorizing formulas.
-
Break Down Complex Problems: Divide complex problems into smaller, manageable steps.
-
Check Your Work: Always double-check your calculations to ensure accuracy.
Conclusion
The seemingly simple question, "10 is what percent of 60?", opens a gateway to a world of valuable mathematical applications. Understanding percentages is essential for various aspects of life, from personal finance to professional success. Mastering percentage calculations empowers you to make informed decisions, analyze data effectively, and navigate the complexities of the modern world. By understanding the fundamental principles, practicing different calculation methods, and applying this knowledge to real-world scenarios, you can confidently tackle a wide range of percentage-related problems. Remember, consistent practice and a solid grasp of the underlying concepts are key to mastering this important skill.
Latest Posts
Latest Posts
-
How Many Cups Are In 20oz
Apr 19, 2025
-
How Many Diagonals Has A Hexagon
Apr 19, 2025
-
9 As A Percentage Of 25
Apr 19, 2025
-
What Is 1 12 In A Decimal
Apr 19, 2025
-
Cuanto Es 39 Fahrenheit En Celsius
Apr 19, 2025
Related Post
Thank you for visiting our website which covers about 10 Is What Percent Of 60 . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.