1000 Is 2 Percent Of What
Kalali
Apr 16, 2025 · 5 min read
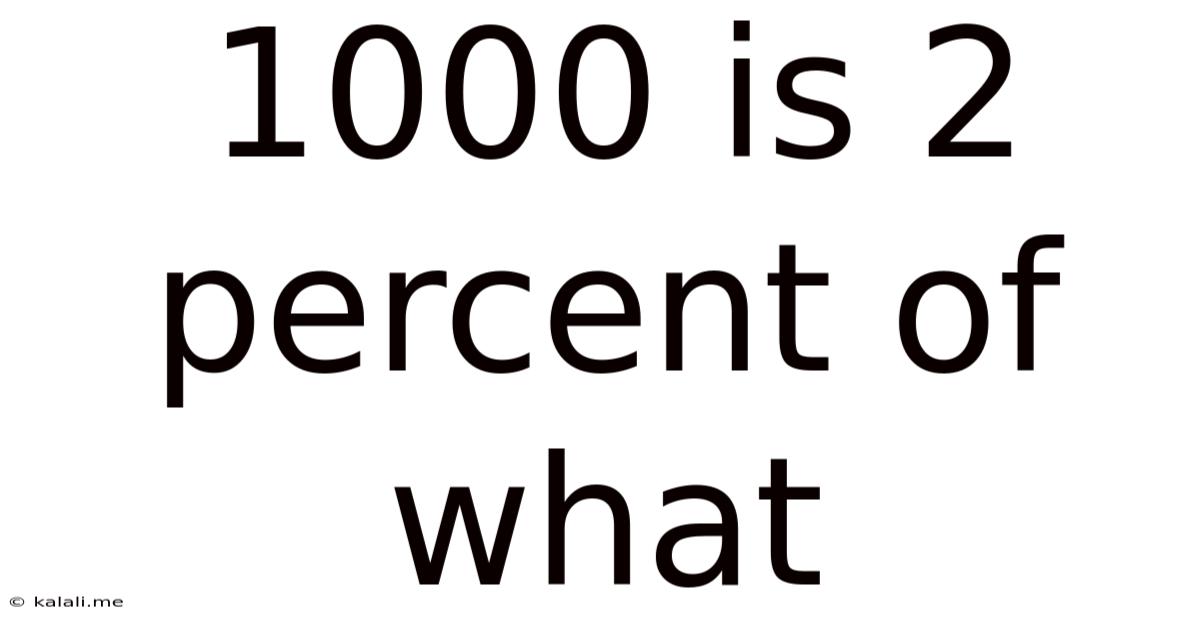
Table of Contents
1000 is 2 Percent of What: A Comprehensive Guide to Percentage Calculations
Finding a number where 2% represents 1000 might seem like a simple math problem, but it touches upon fundamental concepts in percentages, proportions, and algebraic problem-solving. This article will not only solve this specific problem but also delve into the underlying principles, providing you with a comprehensive understanding of percentage calculations and their application in various real-world scenarios. We'll explore different methods for solving this type of problem, making it accessible to everyone, regardless of their mathematical background. Understanding percentage calculations is crucial for budgeting, finance, data analysis, and many other fields.
What is a Percentage?
Before diving into the solution, let's refresh our understanding of percentages. A percentage is a fraction or ratio expressed as a number out of 100. For example, 2% means 2 out of 100, or 2/100, which simplifies to 1/50. Percentages are used to express proportions, often representing a part of a whole.
Understanding the Problem: 1000 is 2% of What?
The question "1000 is 2% of what?" asks us to find the whole number (the total) where 2% of that number equals 1000. We can represent this problem algebraically. Let's use 'x' to represent the unknown whole number:
2% of x = 1000
Method 1: Using the Equation
This method directly tackles the algebraic representation of the problem. We can rewrite the equation as follows:
(2/100) * x = 1000
To solve for x, we need to isolate it. We can do this by multiplying both sides of the equation by 100/2 (or 50):
x = 1000 * (100/2)
x = 1000 * 50
x = 50000
Therefore, 1000 is 2% of 50000.
Method 2: Using Proportions
Proportions offer another elegant way to solve this problem. We can set up a proportion:
2/100 = 1000/x
This proportion states that the ratio of 2 to 100 is equal to the ratio of 1000 to the unknown number (x). To solve for x, we can cross-multiply:
2 * x = 100 * 1000
2x = 100000
x = 100000 / 2
x = 50000
Again, we find that 1000 is 2% of 50000.
Method 3: Working with Decimals
Percentages can easily be converted to decimals. 2% is equivalent to 0.02 (2 divided by 100). We can rewrite the problem as:
0.02 * x = 1000
To solve for x, divide both sides by 0.02:
x = 1000 / 0.02
x = 50000
This method provides the same solution, demonstrating the flexibility of using decimals in percentage calculations.
Real-World Applications of Percentage Calculations
Understanding how to solve percentage problems like "1000 is 2% of what?" has numerous practical applications. Here are some examples:
-
Finance: Calculating interest earned or owed, determining profit margins, analyzing investment returns, and understanding loan repayments all rely heavily on percentage calculations. For example, if you earned $1000 in interest and that represents 2% of your investment, you can determine the total investment amount using the methods described above.
-
Sales and Marketing: Tracking conversion rates, calculating sales growth, analyzing discount offers, and understanding market share all involve percentage calculations. If a company had 1000 sales, and this represents 2% of the total market sales, calculating the total market size is directly applicable.
-
Data Analysis: Percentages are essential for presenting data effectively. Understanding the proportion of different data points, such as customer demographics or product performance, is crucial for informed decision-making. Analyzing survey results often relies on percentages to represent the distribution of responses.
-
Everyday Life: Calculating tips at restaurants, understanding sales tax, figuring out discounts, and even comparing prices often require basic percentage calculations.
Beyond the Basics: More Complex Percentage Problems
While "1000 is 2% of what?" is a straightforward problem, understanding the core concepts allows you to tackle more complex scenarios. Consider these examples:
-
Calculating percentage increase or decrease: If a value increases from 1000 to 1200, what is the percentage increase? This requires understanding the difference between the new and old values and expressing that difference as a percentage of the original value.
-
Compounding percentages: This deals with applying percentages repeatedly over time, often seen in financial calculations involving interest. For instance, calculating the future value of an investment with a compound interest rate requires understanding how percentages build upon each other over time.
-
Finding the original value after a percentage change: If a price has been reduced by 10% to 900, what was the original price? This requires working backwards from the reduced price to the original value.
Mastering Percentage Calculations: Tips and Tricks
Here are some tips to improve your ability to solve percentage problems:
-
Practice regularly: The more you practice, the more comfortable you’ll become with percentage calculations.
-
Master the different methods: Understanding the equation, proportion, and decimal methods allows you to choose the most efficient approach for different problems.
-
Use online resources: There are numerous online calculators and tutorials available to help you learn and practice percentage calculations.
-
Break down complex problems: If you encounter a complex percentage problem, break it down into smaller, more manageable steps.
-
Visualize the problem: Sometimes, visualizing the problem using diagrams or charts can make it easier to understand.
Conclusion:
The seemingly simple question "1000 is 2% of what?" provides a gateway to understanding the fundamental concepts of percentages and their wide-ranging applications. By mastering the techniques outlined in this article, you’ll equip yourself with valuable skills applicable to various aspects of life, from personal finance to professional endeavors. Remember to practice regularly and explore more complex problems to solidify your understanding and become proficient in percentage calculations. The ability to solve these problems confidently is a valuable asset in many aspects of life.
Latest Posts
Latest Posts
-
What Has 4 Letters Sometimes Has 9
Jul 02, 2025
-
How Many Milliliters In A Half Gallon
Jul 02, 2025
-
How Much Can I Sell Bro For
Jul 02, 2025
-
How Many Bottles Is 64 Oz Of Water
Jul 02, 2025
-
How Many Ounces In Pound Of Meat
Jul 02, 2025
Related Post
Thank you for visiting our website which covers about 1000 Is 2 Percent Of What . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.