108 Is What Percent Of 200
Kalali
Apr 18, 2025 · 5 min read
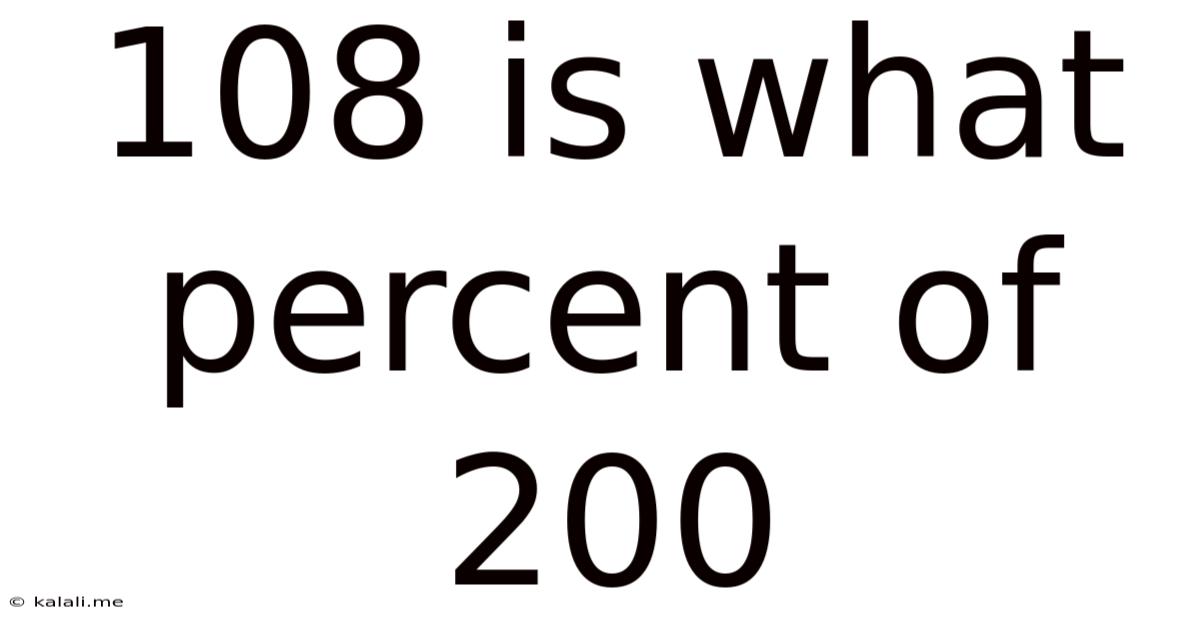
Table of Contents
108 is What Percent of 200? A Comprehensive Guide to Percentage Calculations
Finding out what percentage 108 represents of 200 might seem like a simple math problem. However, understanding the underlying principles behind percentage calculations is crucial not only for solving this specific problem but also for a wide range of applications in everyday life, from calculating discounts and tax rates to analyzing data and understanding financial reports. This comprehensive guide will walk you through solving this problem, explain the methodology, and delve into the broader context of percentage calculations.
Meta Description: Learn how to calculate percentages with a detailed explanation of how to determine what percentage 108 represents of 200. This guide covers the basic formula, practical applications, and advanced percentage concepts.
Understanding the Fundamentals of Percentages
A percentage is simply a fraction or ratio expressed as a number out of 100. The term "percent" comes from the Latin "per centum," meaning "out of a hundred." Therefore, 50% means 50 out of 100, which is equivalent to ½ or 0.5. Percentages are a useful way to represent proportions and compare different quantities.
This understanding is key to solving the problem: "108 is what percent of 200?" We're essentially asking: what fraction of 200 is 108, expressed as a number out of 100?
Calculating the Percentage: The Step-by-Step Method
The basic formula for calculating percentages is:
(Part / Whole) * 100% = Percentage
In our case:
- Part: 108
- Whole: 200
Let's substitute these values into the formula:
(108 / 200) * 100% = 0.54 * 100% = 54%
Therefore, 108 is 54% of 200.
Different Approaches to Solving Percentage Problems
While the above method is the most straightforward, there are other ways to approach this type of problem, particularly helpful depending on the context and the numbers involved.
-
Using Proportions: You can set up a proportion: 108/200 = x/100. Solving for x will give you the percentage. Cross-multiplying gives 10800 = 200x. Dividing both sides by 200 yields x = 54.
-
Decimal Conversion: First, divide 108 by 200 to get 0.54. Then, multiply the decimal by 100 to convert it to a percentage: 0.54 * 100% = 54%. This method is particularly useful when working with calculators.
-
Mental Math (for simpler problems): For easier percentage calculations, you can sometimes use mental shortcuts. For instance, knowing that 50% of 200 is 100, you can deduce that 54% is slightly more. This approach is less precise but offers a quick estimation.
Practical Applications of Percentage Calculations
Understanding percentage calculations is vital in many real-world scenarios:
-
Financial Calculations: Calculating interest rates, discounts, taxes, profits, and losses all involve percentages. For example, figuring out the sale price after a 20% discount or the total cost including a 6% sales tax. Understanding percentage changes (increases or decreases) is also essential in financial analysis.
-
Data Analysis: Percentages are widely used to represent proportions in data sets. For example, analyzing survey results, market share, or the success rate of a particular process. Presenting data as percentages often makes it easier to understand and compare.
-
Scientific Calculations: Percentages are used extensively in scientific fields, such as expressing concentrations (e.g., percentage of a substance in a solution), error rates, and statistical probabilities.
-
Everyday Life: From calculating tips in restaurants to understanding nutritional information on food labels, percentages are encountered frequently in our daily lives. Even simple things like figuring out the percentage of a task completed or the percentage of time spent on different activities involve percentage calculations.
Advanced Percentage Concepts and Problem Solving
Beyond the basic calculations, a deeper understanding of percentages involves several advanced concepts:
-
Percentage Change: This measures the relative change between two values. The formula is: [(New Value - Old Value) / Old Value] * 100%. For example, if a product's price increases from $100 to $120, the percentage increase is [(120 - 100) / 100] * 100% = 20%.
-
Percentage Point Difference: This is a crucial concept often misunderstood. It refers to the arithmetic difference between two percentages, not a percentage change. For example, if the unemployment rate rises from 5% to 7%, the increase is 2 percentage points, not a 40% increase.
-
Compound Interest: This is interest calculated on the initial principal and also on the accumulated interest from previous periods. Understanding compound interest is critical for long-term financial planning and investment strategies.
-
Percentage Increase/Decrease Problems: These problems often involve finding the original value given a percentage change and the new value, or vice-versa. Solving these problems requires careful application of the percentage change formula and algebraic manipulation.
-
Complex Percentage Calculations: Some problems may involve multiple percentage calculations, such as calculating discounts and taxes sequentially or dealing with compound percentage changes. These problems demand a methodical approach, breaking them down into smaller, manageable steps.
Practical Examples and Exercises
Let's explore a few more examples to solidify our understanding:
Example 1: A store offers a 30% discount on an item originally priced at $250. What is the sale price?
- Solution: The discount amount is 30% of $250, which is (0.30 * $250) = $75. The sale price is $250 - $75 = $175.
Example 2: A student scored 85 out of 100 on a test. What is their percentage score?
- Solution: (85 / 100) * 100% = 85%
Example 3: A company's profit increased from $50,000 to $60,000. What is the percentage increase?
- Solution: [(60,000 - 50,000) / 50,000] * 100% = 20%
Conclusion: Mastering Percentage Calculations
Mastering percentage calculations is a valuable skill with far-reaching applications. By understanding the fundamental formula, exploring different approaches, and familiarizing yourself with advanced concepts, you'll be well-equipped to tackle a wide range of percentage-related problems. Remember that practice is key; the more you work with percentage calculations, the more comfortable and efficient you'll become. From everyday finances to complex data analysis, your ability to confidently calculate and interpret percentages will significantly enhance your problem-solving skills and decision-making abilities. So, next time you encounter a percentage problem, you'll be well-prepared to solve it efficiently and accurately.
Latest Posts
Latest Posts
-
2 2 3 As An Improper Fraction
Apr 19, 2025
-
Cuanto Es 8 Pies A Metros
Apr 19, 2025
-
What Is The Mixed Number For 7 4
Apr 19, 2025
-
How Much Is 1 5 Fluid Ounces
Apr 19, 2025
-
What Is Equivalent To 2 Quarts
Apr 19, 2025
Related Post
Thank you for visiting our website which covers about 108 Is What Percent Of 200 . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.