11 Is What Percent Of 12
Kalali
Mar 08, 2025 · 5 min read
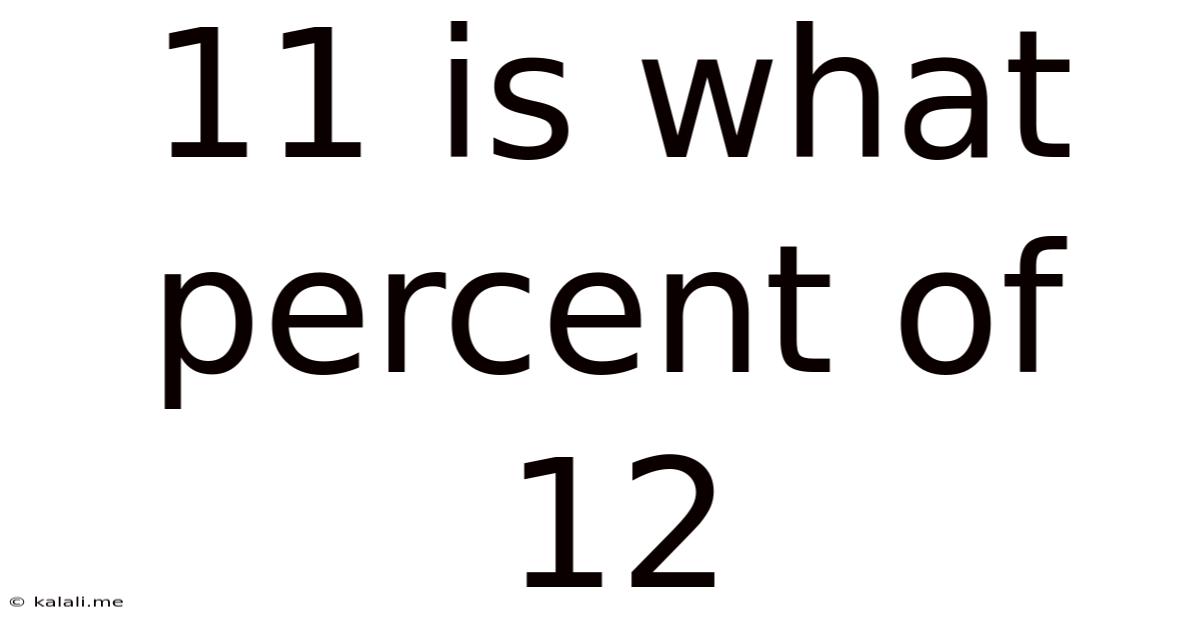
Table of Contents
11 is What Percent of 12? A Deep Dive into Percentage Calculations
Calculating percentages is a fundamental skill in various aspects of life, from understanding sales discounts to analyzing financial reports. This seemingly simple question – "11 is what percent of 12?" – offers a perfect opportunity to explore the mechanics of percentage calculations and delve into practical applications. We'll not only solve this specific problem but also equip you with the knowledge to tackle similar percentage problems with confidence.
Understanding Percentages: The Foundation
Before diving into the calculation, let's solidify our understanding of percentages. A percentage is simply a fraction expressed as a part of 100. The symbol "%" represents "per hundred" or "out of 100." For instance, 50% means 50 out of 100, which is equivalent to the fraction 50/100 or the decimal 0.5.
Calculating "11 is What Percent of 12?"
The question asks us to find the percentage that 11 represents when compared to 12. We can solve this using the following formula:
(Part / Whole) x 100% = Percentage
In this case:
- Part: 11 (the value we're comparing)
- Whole: 12 (the total value)
Substituting these values into the formula:
(11 / 12) x 100% = 0.916666... x 100% ≈ 91.67%
Therefore, 11 is approximately 91.67% of 12.
Different Approaches to Percentage Calculations
While the above method is straightforward, there are other ways to approach this problem. Let's explore some alternative methods:
Method 2: Using Proportions
We can set up a proportion to solve this:
11/12 = x/100
Where 'x' represents the percentage we're trying to find. To solve for x, we cross-multiply:
11 * 100 = 12 * x
1100 = 12x
x = 1100 / 12 ≈ 91.67%
This method provides an alternative pathway to the same solution.
Method 3: Decimal Conversion
Another approach involves converting the fraction into a decimal first and then multiplying by 100%:
11/12 ≈ 0.916666...
0.916666... x 100% ≈ 91.67%
This method showcases the relationship between fractions, decimals, and percentages.
Practical Applications of Percentage Calculations
The ability to calculate percentages is crucial in a wide range of situations. Here are some examples:
1. Sales and Discounts:
Imagine a store offering a 20% discount on an item priced at $100. To calculate the discount amount, you'd find 20% of $100:
(20/100) x $100 = $20
The discounted price would then be $100 - $20 = $80.
Understanding percentage discounts is essential for making informed purchasing decisions. Calculating the final price after a discount is a common application of percentage calculations. This skill enables consumers to compare prices effectively and save money.
2. Financial Analysis:
Percentage calculations are fundamental in financial analysis. Investors use percentages to analyze things like:
- Return on Investment (ROI): Measuring the profitability of an investment.
- Profit Margins: Determining the percentage of revenue remaining after deducting costs.
- Growth Rates: Assessing the increase or decrease in revenue or profits over time.
Understanding these financial metrics helps investors make informed decisions about investments. Fluctuations in percentage changes can signal significant trends in financial performance.
3. Statistical Analysis:
In statistics, percentages are frequently used to represent data proportions. For example, in surveys or polls, results are often presented as percentages to show the proportion of respondents who chose a particular option. Accurate percentage calculations are vital for drawing meaningful conclusions from statistical data.
4. Scientific Calculations:
Many scientific calculations involve percentages. For example, calculating concentrations of solutions or determining the percentage of a particular element in a compound relies heavily on accurate percentage computations. This application extends to diverse scientific fields, including chemistry, biology, and environmental science.
5. Everyday Life:
Beyond formal applications, percentage calculations are part of our daily lives. We use them to:
- Tip Calculations: Determining the appropriate tip amount in a restaurant.
- Tax Calculations: Calculating sales tax or income tax.
- Grade Calculations: Understanding your performance in school based on percentages.
These everyday applications highlight the pervasiveness of percentages in our daily lives and the importance of understanding how to calculate them accurately.
Rounding and Accuracy
In our example, we rounded the answer to 91.67%. The exact value is a repeating decimal. The level of accuracy needed depends on the context. For everyday calculations, rounding to two decimal places is often sufficient. However, in situations requiring higher precision, such as scientific or financial applications, more decimal places might be necessary. Always consider the context and the required level of accuracy when rounding your results.
Avoiding Common Mistakes
Common mistakes in percentage calculations include:
- Incorrectly placing the decimal point: Be mindful when converting fractions or decimals to percentages and vice versa.
- Using the wrong formula: Double-check that you're using the appropriate formula for the specific problem.
- Rounding errors: Be aware of potential rounding errors, especially when performing multiple calculations.
Always double-check your work and use a calculator if necessary to minimize these errors.
Expanding Your Knowledge
Understanding percentages is a stepping stone to more advanced mathematical concepts. Exploring related topics like ratios, proportions, and fractions will further strengthen your quantitative skills. Practicing various percentage problems will build your confidence and proficiency.
Conclusion
The question "11 is what percent of 12?" serves as a practical illustration of percentage calculations. We've explored different methods for solving this problem and highlighted the widespread applications of percentage calculations in various fields. Mastering these calculations is not just a mathematical skill but a valuable life skill that enhances your ability to analyze data, make informed decisions, and navigate the quantitative aspects of daily life. Remember to practice regularly to hone your skills and overcome any challenges you might encounter. The more you practice, the more confident and proficient you'll become in tackling percentage problems of any complexity.
Latest Posts
Latest Posts
-
How Long Does It Take To Drive 2000 Miles
Jul 18, 2025
-
How Many Grams In A Teaspoon Of Cinnamon
Jul 18, 2025
-
How Long To Heat Water In Microwave
Jul 18, 2025
-
40 Oz Of Water Is How Many Cups
Jul 18, 2025
-
How Many Eighths In A Quarter Pound
Jul 18, 2025
Related Post
Thank you for visiting our website which covers about 11 Is What Percent Of 12 . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.