12 Is What Percent Of 15
Kalali
Apr 04, 2025 · 4 min read
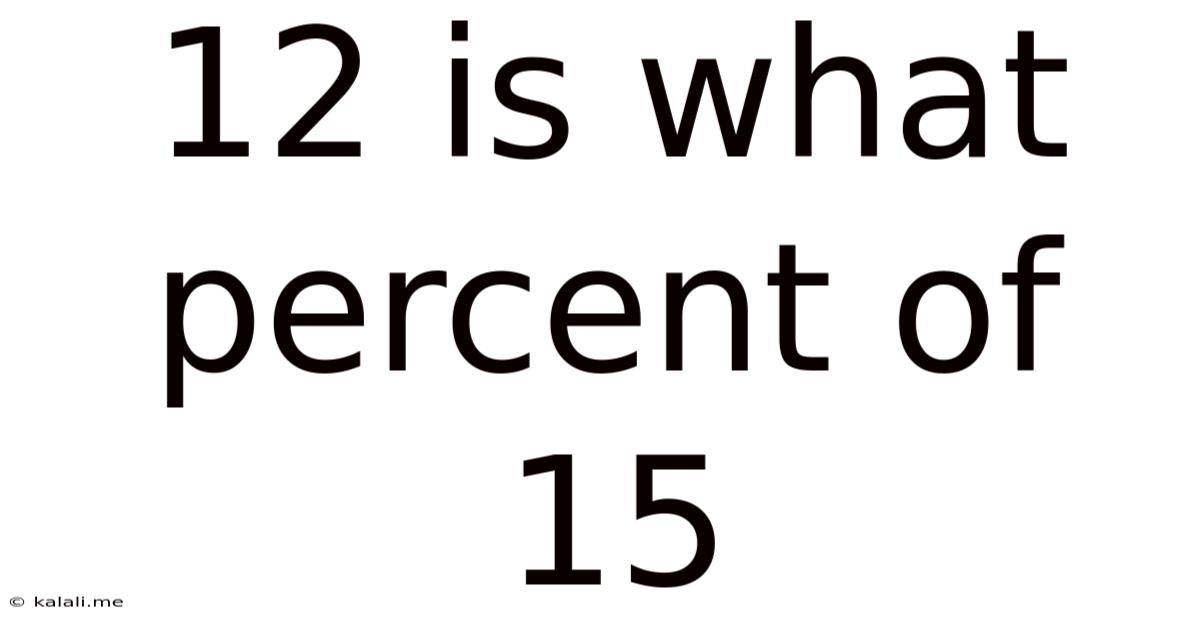
Table of Contents
12 is What Percent of 15? A Comprehensive Guide to Percentage Calculations
Finding out what percentage 12 represents of 15 might seem like a simple arithmetic problem. However, understanding the underlying principles of percentage calculations is crucial for various applications, from everyday budgeting to complex financial analysis. This comprehensive guide will delve into solving this specific problem and expand on the broader concepts of percentages, providing you with a strong foundation for tackling similar calculations in the future.
Understanding Percentages
A percentage is a way of expressing a number as a fraction of 100. The term "percent" literally means "out of one hundred." Therefore, 10% means 10 out of 100, which can be written as the fraction 10/100 or the decimal 0.10. Understanding this fundamental concept is essential for mastering percentage calculations.
Method 1: Using the Formula
The most straightforward way to calculate what percentage 12 is of 15 is to use the basic percentage formula:
(Part / Whole) x 100% = Percentage
In this case:
- Part: 12 (the number we want to express as a percentage)
- Whole: 15 (the total number)
Substituting these values into the formula:
(12 / 15) x 100% = 80%
Therefore, 12 is 80% of 15.
Method 2: Decimal Conversion
This method involves converting the fraction into a decimal first, and then multiplying by 100%.
- Divide the part by the whole: 12 / 15 = 0.8
- Multiply the decimal by 100%: 0.8 x 100% = 80%
This method yields the same result: 12 is 80% of 15.
Method 3: Using Proportions
Proportions offer another effective approach to solving percentage problems. We can set up a proportion:
12/15 = x/100
Where 'x' represents the percentage we're trying to find.
To solve for x:
- Cross-multiply: 12 * 100 = 15 * x
- Simplify: 1200 = 15x
- Solve for x: x = 1200 / 15 = 80
Therefore, 12 is 80% of 15.
Real-World Applications of Percentage Calculations
Understanding percentage calculations is invaluable in numerous real-world scenarios:
-
Finance: Calculating interest rates, discounts, tax rates, profit margins, and investment returns all rely heavily on percentage calculations. For instance, understanding the percentage change in your investment portfolio is crucial for making informed financial decisions.
-
Retail: Sales discounts, markups, and calculating the final price after taxes all involve percentages. Knowing how to calculate these quickly is essential for both consumers and retailers.
-
Science and Statistics: Percentages are widely used to represent data in scientific studies and statistical analysis. For example, researchers might report the percentage of participants who responded positively to a treatment.
-
Everyday Life: Calculating tips in restaurants, understanding discounts in stores, and even figuring out the percentage of tasks completed on a project all require a solid grasp of percentages.
Advanced Percentage Problems and Solutions
While the problem "12 is what percent of 15?" is relatively straightforward, let's explore more complex percentage scenarios:
Scenario 1: Finding the Whole
-
Problem: 20% of a number is 10. What is the number?
-
Solution: We can use the formula: (Part / Percentage) x 100 = Whole
(10 / 20) x 100 = 50
Therefore, the number is 50.
Scenario 2: Finding the Percentage Increase or Decrease
-
Problem: A product initially priced at $50 is now priced at $60. What is the percentage increase?
-
Solution: First, calculate the difference: $60 - $50 = $10
Next, divide the difference by the original price and multiply by 100%: ($10 / $50) x 100% = 20%
Therefore, the price increased by 20%.
Scenario 3: Compound Percentage Changes
-
Problem: A quantity increases by 10% and then decreases by 10%. What is the net percentage change?
-
Solution: This scenario highlights a common misconception. It's tempting to assume the net change is 0%, but that's incorrect. Let's say the initial quantity is 100.
- 10% increase: 100 + (10% of 100) = 110
- 10% decrease: 110 - (10% of 110) = 99
The net change is a 1% decrease. Compound percentage changes are not simply additive.
Tips and Tricks for Mastering Percentage Calculations
-
Practice regularly: The more you practice, the more comfortable you'll become with these calculations.
-
Use a calculator: Calculators can significantly speed up the process, especially for more complex problems. However, understanding the underlying concepts remains essential.
-
Learn the shortcuts: Memorizing common percentage equivalents (e.g., 25% = 1/4, 50% = 1/2) can save time.
-
Break down complex problems: Divide larger problems into smaller, manageable steps.
-
Check your work: Always double-check your calculations to ensure accuracy.
Conclusion
Calculating percentages is a fundamental skill applicable across a wide range of fields. While finding that 12 is 80% of 15 might appear straightforward, understanding the underlying methods and applying them to more complex problems is key to success. By mastering the techniques and practicing regularly, you can build confidence and proficiency in tackling percentage calculations with ease, empowering you to make better decisions in various aspects of your life. Remember to always double-check your answers to ensure accuracy, and don't hesitate to utilize calculators for efficiency, especially when dealing with larger numbers or more complex equations. Consistent practice and a strong grasp of the core principles will make you a percentage calculation expert in no time.
Latest Posts
Latest Posts
-
10 To The Power Of 13
Apr 10, 2025
-
What Is The Lcm Of 12 8
Apr 10, 2025
-
Is A Banana Turning Brown A Chemical Change
Apr 10, 2025
-
Whats 20 Percent Off 40 Dollars
Apr 10, 2025
-
79 Do F Bang Bao Nhieu Do C
Apr 10, 2025
Related Post
Thank you for visiting our website which covers about 12 Is What Percent Of 15 . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.