12 Out Of 20 In Percentage
Kalali
Apr 07, 2025 · 5 min read
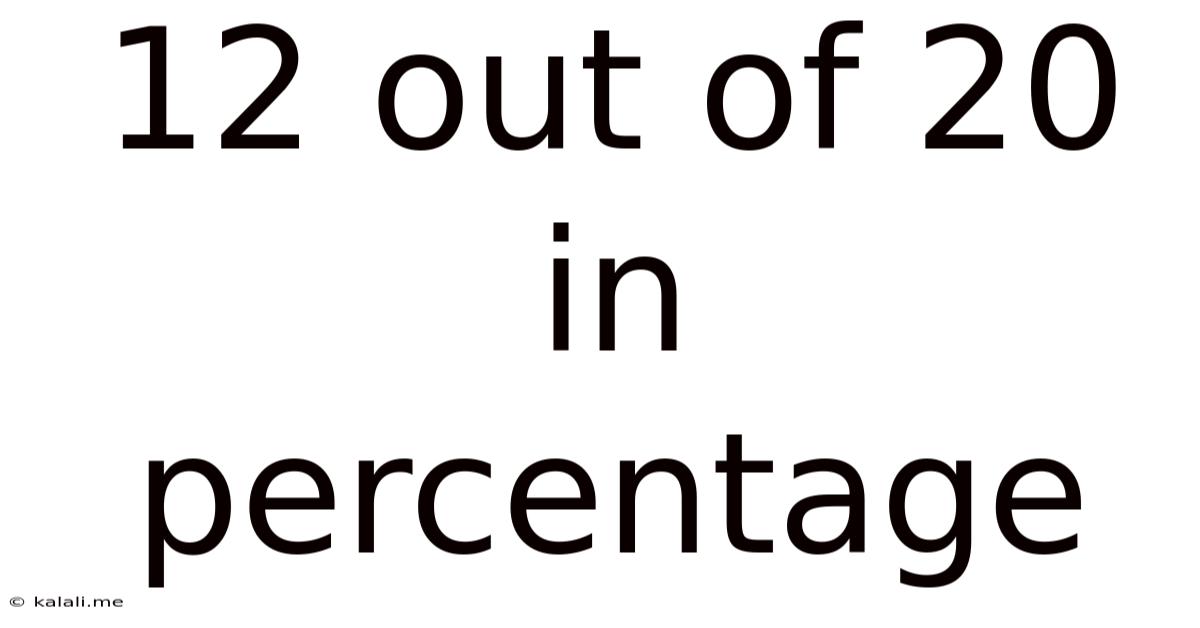
Table of Contents
12 Out of 20: Percentage, Fraction, and Real-World Applications
Calculating percentages is a fundamental skill applicable across numerous fields, from academic assessments to financial transactions and data analysis. Understanding how to express a portion of a whole as a percentage is crucial for interpreting information and making informed decisions. This comprehensive guide will delve into the specifics of calculating "12 out of 20" as a percentage, exploring the underlying mathematical concepts and providing practical examples to solidify your understanding.
Understanding the Fundamentals: Fractions and Percentages
Before we dive into the calculation, let's review the basic concepts of fractions and percentages. A fraction represents a part of a whole. In the case of "12 out of 20," the fraction is expressed as 12/20. This means 12 is the part, and 20 is the whole.
A percentage, denoted by the symbol %, represents a fraction of 100. Therefore, converting a fraction to a percentage involves finding the equivalent fraction with a denominator of 100.
Calculating 12 Out of 20 as a Percentage
The process of converting 12/20 to a percentage involves these steps:
-
Convert the fraction to a decimal: Divide the numerator (12) by the denominator (20): 12 ÷ 20 = 0.6
-
Convert the decimal to a percentage: Multiply the decimal by 100: 0.6 × 100 = 60
Therefore, 12 out of 20 is 60%.
Simplifying the Fraction: A Shortcut
Notice that the fraction 12/20 can be simplified. Both the numerator and the denominator are divisible by 4:
12 ÷ 4 = 3 20 ÷ 4 = 5
This simplifies the fraction to 3/5. Now, to convert 3/5 to a percentage:
-
Convert to a decimal: 3 ÷ 5 = 0.6
-
Convert to a percentage: 0.6 × 100 = 60%
This demonstrates that simplifying the fraction beforehand can make the calculation easier, particularly with larger numbers.
Real-World Applications of Percentage Calculations
The ability to calculate percentages is invaluable in various real-world scenarios:
1. Academic Performance:
Imagine a student scoring 12 out of 20 on a test. Knowing that this equates to 60% allows for a clear understanding of their performance relative to the total possible marks. This percentage can be compared to other scores and used to track academic progress.
2. Financial Calculations:
Percentages are fundamental in financial matters. For example, calculating interest rates, discounts, taxes, and profit margins all involve percentage calculations. Understanding percentages helps in budgeting, investing, and managing personal finances effectively. Consider a scenario where a product is discounted by 20%. If the original price is $50, the discount is $10 (20% of $50), resulting in a final price of $40.
3. Data Analysis and Statistics:
Percentages are essential tools for presenting and interpreting data. In surveys, polls, and market research, data is often expressed as percentages to provide a clear and concise representation of findings. For instance, if 12 out of 20 people surveyed prefer a particular brand, this represents 60% market share.
4. Sales and Marketing:
Companies use percentages extensively to track sales performance, conversion rates, and customer acquisition costs. Analyzing these metrics helps businesses understand their market position, identify trends, and refine their marketing strategies.
5. Healthcare:
In healthcare, percentages are vital in analyzing disease prevalence, treatment success rates, and patient outcomes. For example, a 60% success rate for a particular treatment indicates that the treatment was successful in 60 out of every 100 patients.
6. Scientific Research:
In scientific research, percentages are used to express experimental results, error margins, and statistical significance. Accurate percentage calculations are essential for drawing valid conclusions from experimental data.
7. Everyday Life:
Even everyday tasks frequently involve percentage calculations. For example, calculating tips in restaurants, determining sales tax on purchases, or understanding discounts on products all require understanding percentages.
Beyond the Basics: Working with Percentages
While calculating 12 out of 20 as a percentage is straightforward, mastering percentage calculations involves a broader understanding of different types of percentage problems:
-
Finding the percentage of a number: This involves multiplying the number by the percentage (expressed as a decimal). For example, finding 25% of 80 is 80 × 0.25 = 20.
-
Finding what percentage one number is of another: This involves dividing the part by the whole and multiplying by 100. This is the same principle used to calculate 12 out of 20 as a percentage.
-
Finding the original number when a percentage is known: This involves working backward. For example, if 60% of a number is 30, the original number can be found by dividing 30 by 0.6, which equals 50.
Practical Tips for Mastering Percentage Calculations
-
Practice regularly: The more you practice, the more comfortable and efficient you'll become in calculating percentages.
-
Use online calculators: While understanding the underlying concepts is crucial, online calculators can be helpful for double-checking answers and solving complex problems.
-
Break down complex problems: Complex percentage problems can be broken down into smaller, simpler steps to make the calculations easier to manage.
-
Understand the context: Always pay close attention to the context of the problem to ensure you're using the correct approach.
-
Utilize mental math strategies: With practice, you can develop mental math strategies to quickly estimate percentages. For example, 10% of a number is easily found by moving the decimal point one place to the left.
Conclusion
Mastering percentage calculations is an essential skill applicable across a wide array of contexts. Understanding how to convert fractions to percentages, simplifying fractions to streamline calculations, and applying these skills to real-world scenarios will significantly enhance your problem-solving capabilities and improve your ability to interpret data and make informed decisions. From academic assessments to financial planning and data analysis, the ability to accurately and efficiently calculate percentages is a valuable asset in both personal and professional settings. The seemingly simple calculation of "12 out of 20" serves as a gateway to understanding the broader applications and significance of percentage calculations in everyday life.
Latest Posts
Latest Posts
-
How Many Inches Are In 4 Meters
Apr 10, 2025
-
How Tall Is 90 Cm In Feet
Apr 10, 2025
-
What Is 5 Cups In Ounces
Apr 10, 2025
-
What Does The Diaphragm Do In A Microscope
Apr 10, 2025
-
What Is 175 Degrees Celsius In Fahrenheit
Apr 10, 2025
Related Post
Thank you for visiting our website which covers about 12 Out Of 20 In Percentage . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.