15 Is What Percent Of 100
Kalali
Apr 22, 2025 · 5 min read
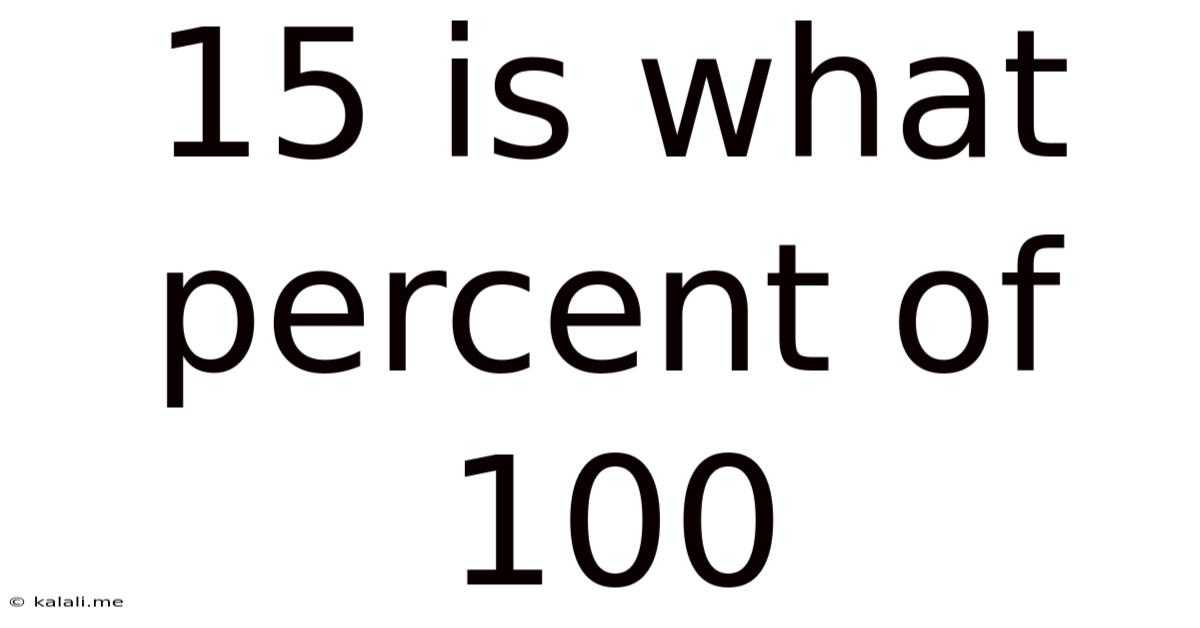
Table of Contents
15 is What Percent of 100? A Deep Dive into Percentage Calculations and Their Applications
This seemingly simple question, "15 is what percent of 100?", opens the door to a fascinating world of percentage calculations, their widespread applications, and the underlying mathematical principles. Understanding percentages is crucial in various aspects of life, from finance and budgeting to sales, statistics, and even everyday decision-making. This article will not only answer the initial question but also explore the broader context of percentage calculations, providing you with a comprehensive understanding of this fundamental mathematical concept.
Meta Description: Discover how to calculate percentages, understand the concept of "15 is what percent of 100?", and explore its real-world applications in finance, statistics, and more. This in-depth guide provides clear explanations and practical examples.
Understanding Percentages: A Foundation
A percentage is a way of expressing a number as a fraction of 100. The word "percent" itself comes from the Latin phrase "per centum," meaning "out of a hundred." Therefore, 15% means 15 out of 100, or 15/100. This fraction can be simplified to 3/20, but the percentage form is often preferred for its intuitive understanding and ease of comparison.
Percentages are invaluable because they allow us to compare proportions easily. Whether it's comparing sales figures, analyzing survey results, or understanding interest rates, percentages provide a standardized method for representing relative quantities.
Solving "15 is What Percent of 100?"
The answer to the question "15 is what percent of 100?" is straightforward. Since a percentage represents a fraction of 100, we can directly express 15 out of 100 as a percentage:
15/100 = 15%
Therefore, 15 is 15% of 100.
The General Formula for Percentage Calculations
The question above represents a specific instance of a more general problem: finding what percentage one number represents of another. The general formula to solve such problems is:
(Part / Whole) * 100 = Percentage
Where:
- Part: The number you want to express as a percentage.
- Whole: The total number.
- Percentage: The resulting percentage.
Real-World Applications of Percentage Calculations
Percentages are ubiquitous in numerous fields. Let's explore some key areas:
1. Finance and Budgeting:
- Interest Rates: Banks and financial institutions use percentages extensively to calculate interest on loans, savings accounts, and credit cards. Understanding interest rates is crucial for making informed financial decisions. For example, a 5% interest rate on a loan means you pay 5% of the loan amount as interest each year.
- Investment Returns: Investors track their returns on investments using percentages. A 10% return on investment means your investment increased by 10% of its initial value.
- Taxes: Taxes are often expressed as percentages of income, sales, or property value. Understanding tax rates is essential for budgeting and financial planning.
- Discounts and Sales: Retailers commonly use percentages to advertise discounts and sales. A "20% off" sale means you pay 80% of the original price.
2. Statistics and Data Analysis:
- Survey Results: Survey data is often summarized using percentages. For instance, if 60 out of 100 respondents prefer a particular product, this is reported as 60%.
- Data Representation: Percentages are used extensively in charts and graphs to represent proportions of data visually. Pie charts, bar graphs, and line graphs frequently use percentages to illustrate relationships between data points.
- Probability: Percentages are fundamental in expressing probabilities. For example, a 75% chance of rain indicates a high likelihood of precipitation.
3. Sales and Marketing:
- Sales Targets: Sales teams often set sales targets expressed as percentages of total potential sales. Reaching a 90% sales target means the team achieved 90% of its projected sales goals.
- Market Share: Companies track their market share, which is the percentage of the total market that they control. A 25% market share indicates that the company holds 25% of the overall market for a particular product or service.
- Conversion Rates: In online marketing, conversion rates (the percentage of website visitors who complete a desired action, such as making a purchase) are key performance indicators.
4. Everyday Life:
- Tips and Gratuities: Restaurants often suggest tipping percentages (e.g., 15%, 20%) of the bill total.
- Recipe Adjustments: When cooking, you might adjust ingredient quantities based on percentages. For example, increasing a recipe by 50% means you use 150% of the original ingredient amounts.
- Grading Systems: Many educational systems use percentages to represent grades (e.g., 90% = A, 80% = B).
Advanced Percentage Calculations
While the basic formula is straightforward, more complex percentage problems might require multiple steps. Consider these examples:
1. Finding the Whole when given the Part and Percentage:
If 20 is 40% of a number, what is that number?
- We can set up the equation: 20 = 0.40 * x (where x is the unknown number).
- Solving for x: x = 20 / 0.40 = 50.
- Therefore, 20 is 40% of 50.
2. Finding the Percentage Increase or Decrease:
If a price increased from $100 to $120, what is the percentage increase?
- The increase is $120 - $100 = $20.
- The percentage increase is ($20 / $100) * 100 = 20%.
3. Calculating Percentage Points:
Percentage points represent the arithmetic difference between two percentages. For example, if the unemployment rate increases from 5% to 8%, it increased by 3 percentage points, not 3%. This distinction is crucial for accurate interpretation of data.
Tips for Mastering Percentage Calculations
- Practice regularly: The more you practice percentage problems, the more comfortable you will become with the calculations.
- Use a calculator: For complex calculations, a calculator can save time and improve accuracy.
- Understand the context: Always carefully read the problem statement to understand what is being asked.
- Check your work: After solving a problem, double-check your answer to ensure its accuracy.
Conclusion
The seemingly simple question, "15 is what percent of 100?", serves as an excellent starting point for understanding the broader concept of percentages and their widespread applications. Mastering percentage calculations is a valuable skill applicable across various fields, from finance and statistics to everyday decision-making. By understanding the underlying principles and practicing regularly, you can confidently tackle percentage problems and effectively utilize this essential mathematical tool. Remember the fundamental formula (Part / Whole) * 100 = Percentage, and don't hesitate to use the various techniques and examples outlined in this article to improve your skills. The more you engage with percentage calculations, the more intuitive and natural they will become.
Latest Posts
Latest Posts
-
How Many Gallons Are In The Ocean
Apr 22, 2025
-
The Quantity Of Matter In An Object
Apr 22, 2025
-
How Tall In Feet Is 48 Inches
Apr 22, 2025
-
152 Cm Is How Many Inches
Apr 22, 2025
-
Is A Rectangle A Regular Polygon
Apr 22, 2025
Related Post
Thank you for visiting our website which covers about 15 Is What Percent Of 100 . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.