162 Out Of 200 In Percentage
Kalali
Apr 07, 2025 · 6 min read
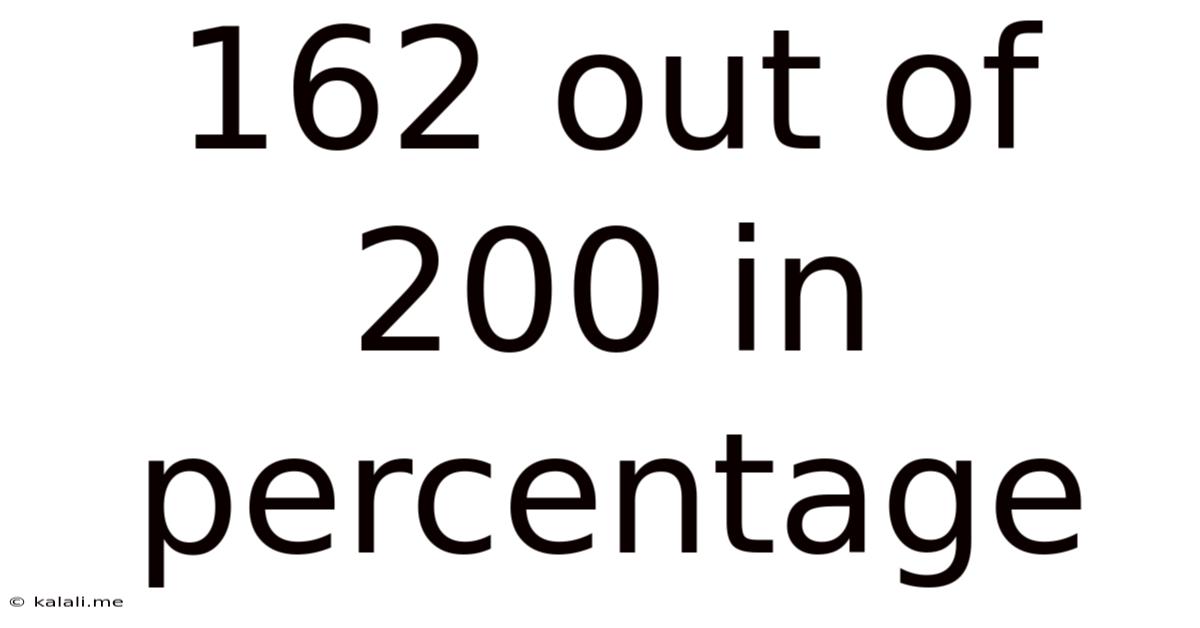
Table of Contents
162 out of 200 in Percentage: A Comprehensive Guide to Percentage Calculations
Calculating percentages is a fundamental skill with widespread applications in various aspects of life, from academic assessments and financial transactions to everyday decision-making. Understanding how to express a portion of a whole as a percentage is crucial for interpreting data, making comparisons, and drawing meaningful conclusions. This comprehensive guide will delve into the intricacies of calculating percentages, specifically focusing on determining the percentage that 162 represents out of 200, and provide you with a strong foundation for tackling similar problems in the future.
Understanding Percentages
Before we dive into the calculation of 162 out of 200, let's first solidify our understanding of percentages. A percentage is a way of expressing a number as a fraction of 100. The term "percent" literally means "out of 100" or "per hundred." The symbol "%" is used to represent percentages.
For example, 50% means 50 out of 100, which is equivalent to the fraction 50/100 or the decimal 0.5. Understanding this fundamental relationship between percentages, fractions, and decimals is essential for accurate percentage calculations.
Calculating 162 out of 200 as a Percentage
To determine the percentage that 162 represents out of 200, we follow these simple steps:
-
Formulate the Fraction: Express the given numbers as a fraction. In this case, the fraction is 162/200. This fraction represents the proportion of 162 out of the total 200.
-
Convert the Fraction to a Decimal: Divide the numerator (162) by the denominator (200). This gives us:
162 ÷ 200 = 0.81
-
Convert the Decimal to a Percentage: Multiply the decimal by 100 and add the percentage symbol (%). This yields:
0.81 × 100% = 81%
Therefore, 162 out of 200 is 81%.
Alternative Calculation Methods
While the above method is straightforward and widely applicable, there are other ways to calculate percentages that might be more suitable depending on the context and your comfort level with different mathematical approaches.
Using Proportions
Proportions can be a powerful tool for solving percentage problems. We can set up a proportion to solve for the unknown percentage (x):
162/200 = x/100
To solve for x, we cross-multiply:
162 * 100 = 200 * x
16200 = 200x
x = 16200 / 200
x = 81
Therefore, x = 81%, confirming our previous result.
Using a Calculator
Modern calculators often have built-in percentage functions. Simply input 162, press the division symbol (/), input 200, and then press the percentage (%) button. The calculator will directly display the result as 81%. This method is particularly useful for quick calculations, especially with larger numbers.
Practical Applications of Percentage Calculations
The ability to calculate percentages is not merely an academic exercise; it has far-reaching practical applications in many areas of life. Here are some examples:
Academic Performance
Percentages are commonly used to represent academic grades and scores. For instance, if a student answers 162 out of 200 questions correctly on a test, their score is 81%, indicating a strong performance.
Financial Calculations
Percentages are fundamental in financial calculations, such as calculating interest rates, discounts, taxes, and profit margins. Understanding percentage changes helps in tracking investment performance and making informed financial decisions. For instance, a 10% discount on a $200 item would result in a $20 reduction, leading to a final price of $180.
Data Analysis and Interpretation
In data analysis, percentages are essential for presenting data in a clear and easily understandable manner. They are frequently used in surveys, polls, and statistical reports to express proportions and trends. For example, a survey indicating that 81% of respondents support a particular policy provides a concise and powerful representation of public opinion.
Everyday Life
Percentage calculations pop up in everyday scenarios. For example, calculating tips in restaurants, understanding sales discounts at stores, or determining the percentage of completion of a project all involve percentage calculations. The ability to quickly and accurately calculate percentages empowers individuals to make better-informed decisions in these everyday situations.
Expanding on Percentage Calculations: Beyond the Basics
Understanding the basics of percentage calculation, as demonstrated with the 162 out of 200 example, is crucial. However, mastering percentage calculations involves understanding more complex scenarios.
Percentage Increase and Decrease
Calculating percentage increases or decreases involves finding the difference between two numbers and expressing this difference as a percentage of the original number.
For example, if a price increases from $100 to $120, the increase is $20. The percentage increase is calculated as:
(20/100) * 100% = 20%
Similarly, if a price decreases from $120 to $100, the decrease is $20. The percentage decrease is calculated as:
(20/120) * 100% = 16.67%
Note that the base for the percentage change is the original value.
Percentage Points vs. Percentage Change
It is crucial to differentiate between percentage points and percentage change. A change of, say, 10 percentage points means an absolute change of 10%. However, a 10% percentage change depends on the starting point. For instance, a change from 20% to 30% is a 10 percentage point increase but represents a 50% percentage change (10/20 * 100%).
Compounding Percentages
Compounding percentages involve applying a percentage change to the result of a previous percentage change. This is common in scenarios like compound interest calculations. For example, if an investment grows by 10% in the first year and then by another 10% in the second year, it's not a 20% total growth. Instead, the calculation is:
Year 1: $100 * 1.10 = $110 Year 2: $110 * 1.10 = $121
This represents a total growth of 21%.
Calculating the Original Value
Sometimes, you know the percentage and the final value, but you need to determine the original value. To do this, you reverse the percentage calculation.
For example, if 81% of a value is 162, we can set up the equation:
0.81 * x = 162
To solve for x (the original value), divide both sides by 0.81:
x = 162 / 0.81 = 200
This confirms that the original value was 200.
Conclusion
Mastering percentage calculations is a valuable skill with wide-ranging practical applications. This comprehensive guide has explored the calculation of 162 out of 200 as a percentage, illustrating different methods and highlighting the importance of accurate calculation. Understanding percentage calculations extends beyond simple arithmetic; it's about interpreting data, making informed decisions, and effectively communicating information across various contexts. Whether you're evaluating academic performance, analyzing financial data, or navigating everyday situations, the ability to confidently calculate and interpret percentages is a valuable asset. Continue practicing and expanding your understanding of percentage calculations to enhance your problem-solving skills and navigate the quantitative aspects of the world around you.
Latest Posts
Latest Posts
-
Chocolate Candy Melts In Your Hand Thermal Energy
Apr 07, 2025
-
Does Bbr3 Obey The Octet Rule
Apr 07, 2025
-
How Many Inches Are In 14 Cm
Apr 07, 2025
-
Cuanto Es 75 Cm En Pulgadas
Apr 07, 2025
-
Determine The Molecular Geometry Of Cbr4
Apr 07, 2025
Related Post
Thank you for visiting our website which covers about 162 Out Of 200 In Percentage . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.