17 3 As A Mixed Number
Kalali
Apr 17, 2025 · 5 min read
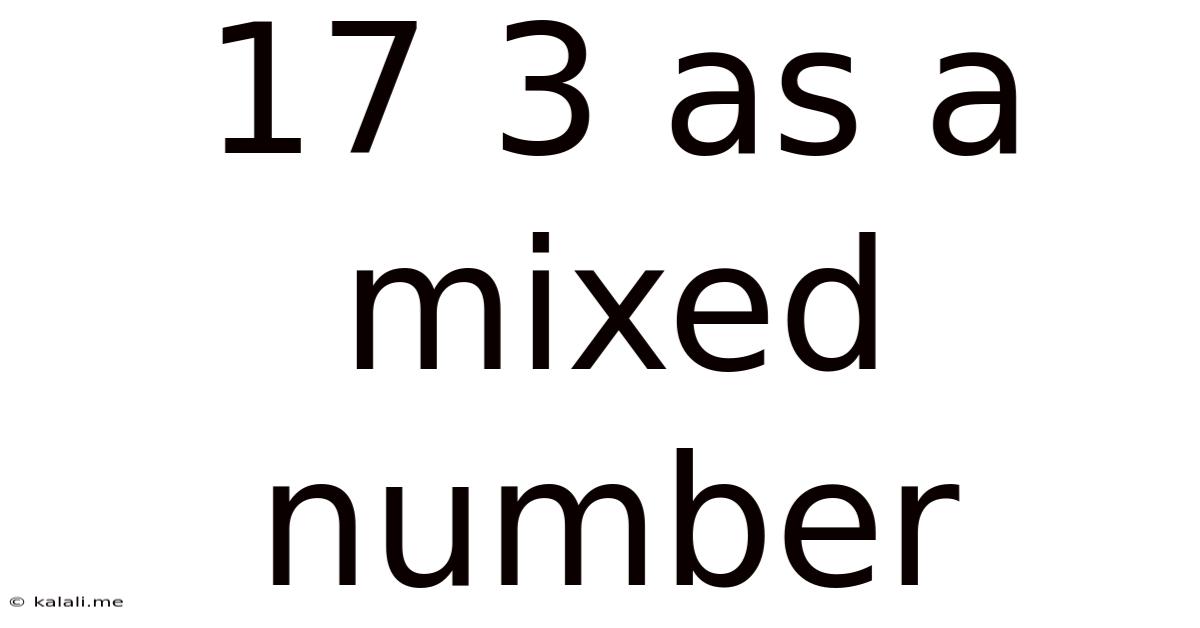
Table of Contents
Understanding 17/3 as a Mixed Number: A Comprehensive Guide
The seemingly simple fraction 17/3 presents a valuable opportunity to delve into the world of fractions, mixed numbers, and the crucial connection between them. This article provides a comprehensive explanation of how to convert the improper fraction 17/3 into a mixed number, explores the underlying concepts, and offers practical applications and examples. Understanding this conversion is fundamental to mastering arithmetic involving fractions and lays the groundwork for more advanced mathematical concepts.
What is a Mixed Number?
Before diving into the conversion of 17/3, let's define what a mixed number is. A mixed number combines a whole number and a proper fraction. A proper fraction is one where the numerator (the top number) is smaller than the denominator (the bottom number). For example, 1 ¾, 2 ⅓, and 5 ⅚ are all mixed numbers. They represent a quantity that's more than one whole unit but less than the next whole number. Mixed numbers offer a more intuitive way to represent quantities than improper fractions, especially in real-world scenarios.
What is an Improper Fraction?
Conversely, an improper fraction has a numerator that is greater than or equal to its denominator. Examples include 7/4, 11/5, and, in our case, 17/3. While improper fractions are perfectly valid mathematical expressions, they aren't always the most practical way to represent a quantity. This is where the conversion to a mixed number becomes extremely useful.
Converting 17/3 to a Mixed Number: The Step-by-Step Process
The conversion of an improper fraction like 17/3 to a mixed number involves a simple division process. Here's a step-by-step guide:
-
Divide the Numerator by the Denominator: Divide 17 (the numerator) by 3 (the denominator). This gives us 5 with a remainder of 2.
-
The Whole Number: The quotient (the result of the division) becomes the whole number part of the mixed number. In this case, the quotient is 5.
-
The Fraction: The remainder (the number left over after the division) becomes the numerator of the fraction part of the mixed number. The denominator remains the same as the original denominator. Therefore, the remainder 2 becomes the numerator, and the denominator remains 3. This gives us the fraction 2/3.
-
Combine the Whole Number and the Fraction: Combine the whole number (5) and the fraction (2/3) to form the mixed number. Therefore, 17/3 as a mixed number is 5 ⅔.
Visual Representation of 17/3
Imagine you have 17 identical objects, and you want to group them into sets of 3. You can form 5 complete sets of 3, with 2 objects remaining. This visually demonstrates that 17/3 is equivalent to 5 wholes and 2/3 of another whole. This visual approach reinforces the understanding of the conversion process.
Why Use Mixed Numbers?
Mixed numbers often provide a clearer and more intuitive representation of quantities in real-world contexts. For example, imagine measuring a length of wood. Saying you have 17/3 meters might be less understandable than saying you have 5 ⅔ meters. The mixed number clearly conveys that you have five full meters plus two-thirds of another meter. This makes it easier to grasp the magnitude of the quantity.
Converting Mixed Numbers Back to Improper Fractions
It's equally important to be able to convert a mixed number back into an improper fraction. This skill is essential for performing calculations involving mixed numbers. The process is as follows:
-
Multiply the Whole Number by the Denominator: Multiply the whole number by the denominator of the fraction. In our example (5 ⅔), this would be 5 x 3 = 15.
-
Add the Numerator: Add the result from step 1 to the numerator of the fraction. 15 + 2 = 17.
-
The New Numerator: This sum becomes the numerator of the improper fraction.
-
Retain the Denominator: The denominator remains the same as the original denominator in the mixed number. In our example, the denominator remains 3.
-
The Improper Fraction: Combine the new numerator and the denominator to form the improper fraction. This gives us 17/3, confirming the reverse conversion.
Practical Applications and Examples
Understanding the conversion between improper fractions and mixed numbers has wide-ranging applications across various fields:
-
Baking and Cooking: Recipes often require fractional amounts of ingredients. Converting improper fractions to mixed numbers provides a more user-friendly representation of these quantities. For instance, a recipe might call for 23/4 cups of flour; converting it to 5 ¾ cups makes the measurement clearer.
-
Construction and Engineering: Precise measurements are crucial in construction and engineering. Converting between improper and mixed fractions ensures accuracy and simplifies calculations when dealing with dimensions and materials.
-
Everyday Measurements: Whether it's measuring distances, volumes, or weights, converting between improper and mixed fractions improves comprehension and simplifies everyday tasks.
-
Advanced Mathematics: The understanding of improper fractions and mixed numbers is foundational to more advanced mathematical concepts such as algebra, calculus, and more. The ability to seamlessly convert between these forms is crucial for solving more complex problems.
Further Exploration and Related Concepts
Understanding the conversion of 17/3 to 5 ⅔ opens the door to a broader exploration of fractions and related concepts:
-
Equivalent Fractions: Understanding that 17/3 and 5 ⅔ are equivalent representations of the same quantity is crucial. This concept helps in simplifying fractions and performing calculations.
-
Simplifying Fractions: While 2/3 is already in its simplest form, learning to simplify fractions is essential for efficient mathematical operations.
-
Adding and Subtracting Fractions: Converting to a common denominator becomes easier with a strong grasp of fractions and mixed numbers.
-
Multiplying and Dividing Fractions: Working with mixed numbers requires converting them to improper fractions before performing multiplication or division, highlighting the importance of this conversion skill.
Conclusion:
Converting the improper fraction 17/3 to the mixed number 5 ⅔ is a fundamental skill in mathematics. This seemingly simple conversion unlocks a deeper understanding of fractions, their practical applications, and their significance in various fields. Mastering this process lays a strong foundation for more advanced mathematical concepts and enhances problem-solving abilities in everyday life. The ability to seamlessly move between improper fractions and mixed numbers is essential for efficiency and clarity in mathematical calculations and real-world applications. This understanding is not just about rote memorization but about grasping the underlying mathematical principles and applying them effectively.
Latest Posts
Latest Posts
-
Cuanto Es 50 Yardas En Metros
Apr 19, 2025
-
How Many Liters Is 70 Ounces
Apr 19, 2025
-
What Is The End Product Of Transcription
Apr 19, 2025
-
Cuanto Es 38 Centimetros En Pulgadas
Apr 19, 2025
-
How Many Fluid Ounces In 1 3 Cup
Apr 19, 2025
Related Post
Thank you for visiting our website which covers about 17 3 As A Mixed Number . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.