17 Out Of 25 As A Percent
Kalali
Apr 14, 2025 · 5 min read
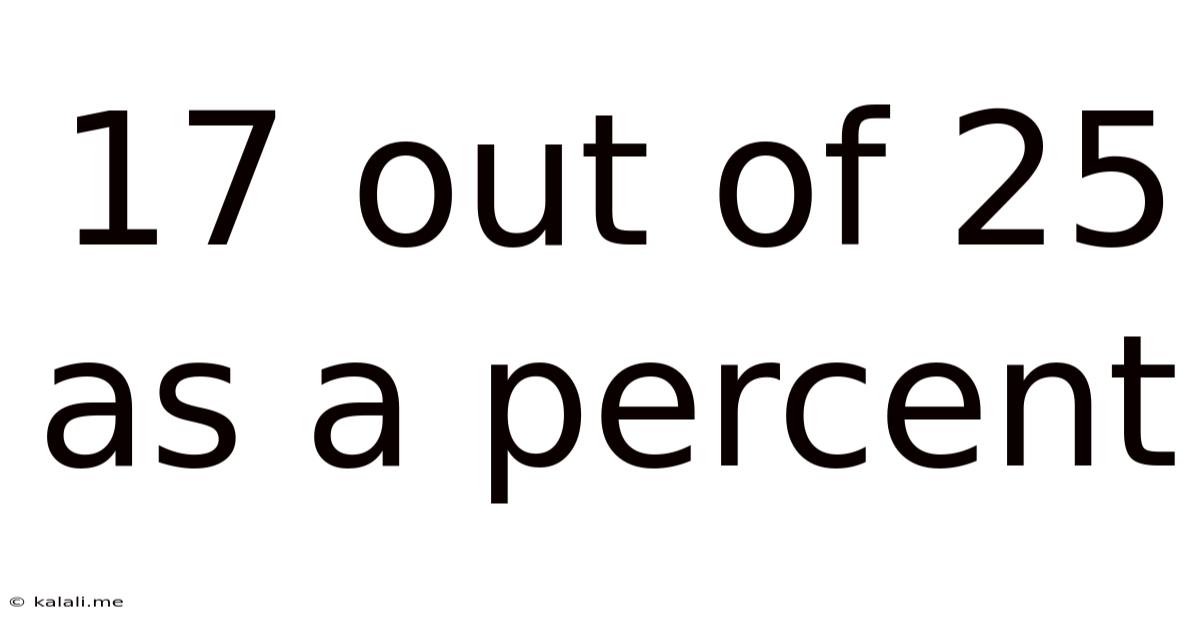
Table of Contents
17 out of 25 as a Percent: A Comprehensive Guide to Percentage Calculations
Calculating percentages is a fundamental skill with applications spanning various fields, from finance and academics to everyday life. Understanding how to convert fractions to percentages is crucial for interpreting data, making informed decisions, and excelling in many areas. This comprehensive guide will explore how to calculate 17 out of 25 as a percent, explain the underlying principles, and provide practical examples to solidify your understanding. We'll also delve into related percentage calculations and offer tips for mastering this essential skill.
What is a Percentage?
A percentage is a way of expressing a number as a fraction of 100. The word "percent" literally means "out of one hundred" ("per cent" from Latin). Percentages are denoted by the symbol "%". For instance, 50% means 50 out of 100, which can also be written as the fraction 50/100 or the decimal 0.5.
Calculating 17 out of 25 as a Percentage:
The core concept behind calculating percentages involves converting a fraction into an equivalent fraction with a denominator of 100. Let's break down how to determine 17 out of 25 as a percentage:
-
Express as a Fraction: The phrase "17 out of 25" is directly represented as the fraction 17/25.
-
Convert to a Fraction with a Denominator of 100: To achieve this, we need to find a number that, when multiplied by 25, equals 100. That number is 4 (25 x 4 = 100). To maintain the value of the fraction, we must multiply both the numerator and the denominator by 4:
(17 x 4) / (25 x 4) = 68/100
-
Express as a Percentage: Since the denominator is now 100, the numerator directly represents the percentage. Therefore, 68/100 is equivalent to 68%.
Therefore, 17 out of 25 is 68%.
Alternative Method: Using Decimal Conversion
Another approach to calculate percentages involves converting the fraction to a decimal first, then multiplying by 100.
-
Convert to a Decimal: Divide the numerator (17) by the denominator (25): 17 ÷ 25 = 0.68
-
Convert to a Percentage: Multiply the decimal by 100: 0.68 x 100 = 68%
This method offers an alternative way to reach the same result. The choice of method depends on personal preference and the specific problem at hand.
Practical Applications and Real-World Examples:
Understanding percentage calculations is crucial for numerous real-world scenarios:
-
Academic Performance: If a student answers 17 out of 25 questions correctly on a test, their score is 68%. This helps assess their understanding of the subject matter.
-
Sales and Discounts: A store offering a 68% discount on an item means the price is reduced by 68% of its original value. Understanding this allows consumers to calculate the final price after the discount.
-
Financial Calculations: Interest rates, loan repayments, and investment returns are often expressed as percentages. Accurate percentage calculations are vital for making sound financial decisions.
-
Statistical Analysis: Percentages are commonly used to represent proportions and probabilities in statistical analysis, allowing researchers to interpret and communicate data effectively.
-
Surveys and Polls: Results from surveys and polls are frequently presented as percentages, providing insights into public opinion and preferences.
Advanced Percentage Calculations:
Beyond the basic calculation of 17 out of 25, let's explore some related percentage problems:
-
Finding the Percentage Increase or Decrease: Suppose a quantity increases from 25 to 42. To calculate the percentage increase:
- Find the difference: 42 - 25 = 17
- Divide the difference by the original value: 17 ÷ 25 = 0.68
- Multiply by 100 to express as a percentage: 0.68 x 100 = 68%
Therefore, there is a 68% increase. Similar calculations can be used to find percentage decreases.
-
Finding a Percentage of a Number: What is 68% of 150?
- Convert the percentage to a decimal: 68% = 0.68
- Multiply the decimal by the number: 0.68 x 150 = 102
Therefore, 68% of 150 is 102.
-
Finding the Original Value: If 68% of a number is 102, what is the original number?
- Let the original number be 'x'. We can set up the equation: 0.68x = 102
- Solve for 'x': x = 102 ÷ 0.68 = 150
Therefore, the original number is 150.
Tips for Mastering Percentage Calculations:
-
Practice Regularly: Consistent practice is key to mastering any mathematical concept, including percentage calculations. Work through various examples to build your confidence and understanding.
-
Utilize Online Resources: Numerous online calculators and tutorials are available to assist with percentage calculations and provide additional practice problems.
-
Understand the Underlying Principles: Focus on understanding the fundamental concepts rather than simply memorizing formulas. This will make it easier to apply your knowledge to different situations.
-
Break Down Complex Problems: If you encounter a complex percentage problem, break it down into smaller, manageable steps. This can simplify the calculation process and reduce the likelihood of errors.
-
Check Your Work: Always check your answers to ensure accuracy. Use alternative methods or compare your results with online calculators to verify your calculations.
Conclusion:
Calculating 17 out of 25 as a percentage, which results in 68%, is a straightforward process that involves converting a fraction to a percentage. This essential skill has widespread applications across diverse fields, requiring a solid understanding of fraction-to-decimal conversions and the principles of percentage calculations. By mastering these concepts and practicing regularly, you can confidently tackle various percentage-related problems and improve your analytical skills in various aspects of your life, both personal and professional. Remember to utilize different methods, check your work, and explore additional resources to solidify your understanding and enhance your proficiency in this crucial mathematical skill. From interpreting test scores to understanding financial data, a strong grasp of percentages is an invaluable asset.
Latest Posts
Latest Posts
-
How Many Cups In 2 Gallons Of Water
Apr 15, 2025
-
How Many Seconds In Four Hours
Apr 15, 2025
-
What Are The Horizontal Rows On A Periodic Table Called
Apr 15, 2025
-
What Is The Conjugate Acid Of Hpo42
Apr 15, 2025
-
The Subatomic Particles Directly Involved In Forming Chemical Bonds Are
Apr 15, 2025
Related Post
Thank you for visiting our website which covers about 17 Out Of 25 As A Percent . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.