18 5 As A Mixed Number
Kalali
Apr 19, 2025 · 5 min read
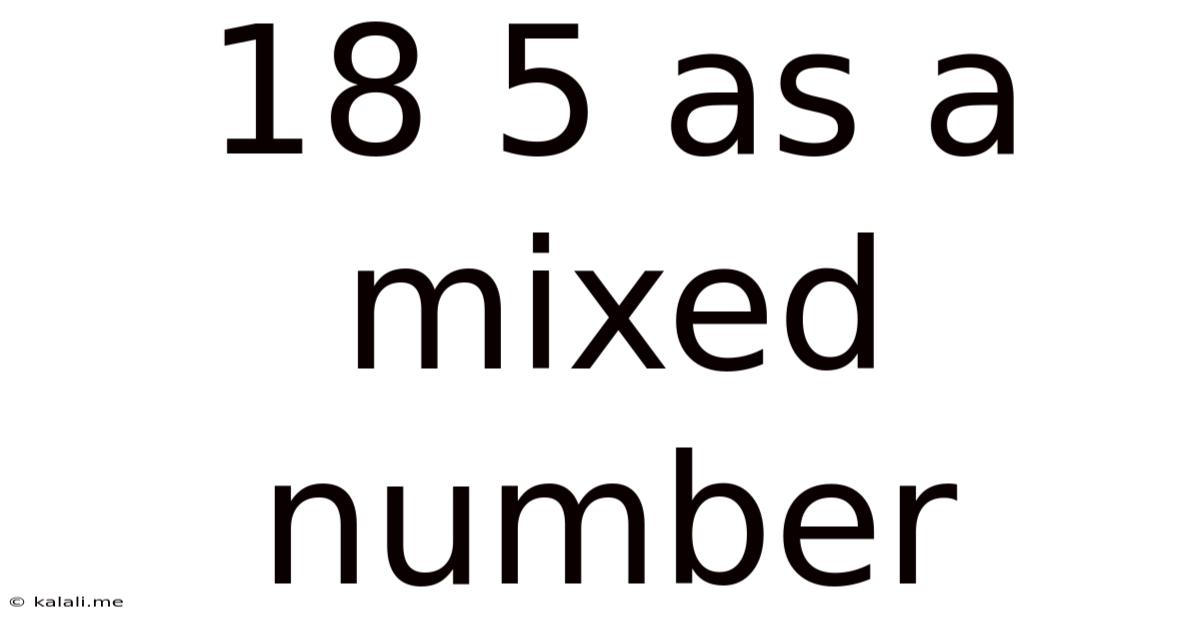
Table of Contents
Understanding 18/5 as a Mixed Number: A Comprehensive Guide
This article delves into the conversion of the improper fraction 18/5 into a mixed number, exploring the underlying concepts and providing practical examples. We'll cover various methods for performing this conversion, explain the significance of mixed numbers, and explore their applications in everyday life and advanced mathematics. Understanding this seemingly simple conversion is crucial for building a strong foundation in fractions and their applications.
What is a Mixed Number?
Before we dive into converting 18/5, let's define what a mixed number is. A mixed number is a combination of a whole number and a proper fraction. A proper fraction is a fraction where the numerator (the top number) is smaller than the denominator (the bottom number). For example, 1 ¼, 3 ⅔, and 2 ⅛ are all mixed numbers. They represent a quantity greater than one.
Why Use Mixed Numbers?
While improper fractions (like 18/5) accurately represent a value, mixed numbers offer a more intuitive and practical way to understand and represent quantities larger than one. They are easier to visualize and work with in many real-world scenarios. Imagine you have 18 slices of pizza, and each pizza has 5 slices. Expressing this as 18/5 is accurate but less clear than saying you have 3 whole pizzas and 3/5 of another. This is the power of mixed numbers – they make fractional amounts more relatable.
Converting 18/5 to a Mixed Number: Step-by-Step Guide
There are two primary methods to convert the improper fraction 18/5 into a mixed number:
Method 1: Division
This is the most common and straightforward method. It involves dividing the numerator (18) by the denominator (5):
-
Divide: 18 ÷ 5 = 3 with a remainder of 3.
-
Whole Number: The quotient (3) becomes the whole number part of the mixed number.
-
Fraction: The remainder (3) becomes the numerator of the fraction, and the original denominator (5) remains the denominator.
Therefore, 18/5 = 3 ⅗
Method 2: Repeated Subtraction
This method is less efficient for larger numbers but provides a deeper understanding of the concept. It involves repeatedly subtracting the denominator from the numerator until you get a remainder smaller than the denominator:
-
Subtract: 18 - 5 = 13
-
Subtract again: 13 - 5 = 8
-
Subtract again: 8 - 5 = 3
-
Counting Subtractions: We subtracted 5 three times (this is our whole number).
-
Remainder: The remaining number (3) is our new numerator.
-
Final Mixed Number: The whole number is 3, and the remaining fraction is 3/5. Therefore, 18/5 = 3 ⅗
Visualizing the Conversion
Imagine you have 18 equal-sized pieces of something. If you group them into sets of 5, you'll have 3 complete sets (representing the whole number 3). You'll have 3 pieces left over, which represents the fraction 3/5. This visual representation perfectly aligns with the result of our calculations.
Applications of Mixed Numbers
Mixed numbers are widely used in various fields:
-
Cooking and Baking: Recipes often use mixed numbers to specify ingredient quantities, such as 2 ½ cups of flour or 1 ¾ teaspoons of baking powder.
-
Construction and Engineering: Measurements in construction and engineering frequently involve mixed numbers, ensuring precision in building and designing structures.
-
Everyday Life: Many everyday situations involve fractions that are best expressed as mixed numbers, such as measuring lengths, splitting costs, or dividing resources.
-
Advanced Mathematics: Mixed numbers are essential in algebra, calculus, and other advanced mathematical fields, where they simplify complex calculations involving fractions.
Converting Mixed Numbers Back to Improper Fractions
It's also important to understand how to convert a mixed number back into an improper fraction. This is often required in more complex mathematical operations. Let's reverse our example:
To convert 3 ⅗ back to an improper fraction:
-
Multiply: Multiply the whole number (3) by the denominator (5): 3 * 5 = 15
-
Add: Add the result to the numerator (3): 15 + 3 = 18
-
Improper Fraction: The result (18) becomes the numerator, and the original denominator (5) remains the denominator. Therefore, 3 ⅗ = 18/5
Further Exploration: Equivalent Fractions and Simplification
Understanding equivalent fractions is vital when working with mixed numbers. Equivalent fractions represent the same value but have different numerators and denominators. For example, 3/5 is equivalent to 6/10, 9/15, and so on. Simplifying fractions, by finding the greatest common divisor (GCD) of the numerator and denominator, ensures the fraction is in its simplest form. In our case, 3/5 is already in its simplest form as 3 and 5 have no common divisors other than 1.
Practice Problems
To solidify your understanding, try converting these improper fractions to mixed numbers:
- 22/7
- 35/8
- 47/12
Solutions:
- 22/7 = 3 ⅛
- 35/8 = 4 ⅜
- 47/12 = 3 11/12
Conclusion
Converting an improper fraction like 18/5 to a mixed number, 3 ⅗, is a fundamental skill in mathematics. Understanding the process, its applications, and the ability to convert back and forth between improper fractions and mixed numbers is crucial for success in various academic and real-world scenarios. Mastering this concept builds a solid foundation for more advanced mathematical concepts and problem-solving. The methods described in this guide provide a comprehensive understanding and empower you to confidently handle similar conversions in the future. Remember to practice regularly to reinforce your skills and build fluency.
Latest Posts
Latest Posts
-
10 Ounces Is Equal To How Many Cups
Apr 22, 2025
-
How Many Feet Is 33 In
Apr 22, 2025
-
How Many Inches Are In 2 80 Ft
Apr 22, 2025
-
32 To The Power Of 2
Apr 22, 2025
-
Find The Inverse Of The Function Y 2x2 4
Apr 22, 2025
Related Post
Thank you for visiting our website which covers about 18 5 As A Mixed Number . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.