183 Out Of 200 As A Percentage
Kalali
Apr 07, 2025 · 5 min read
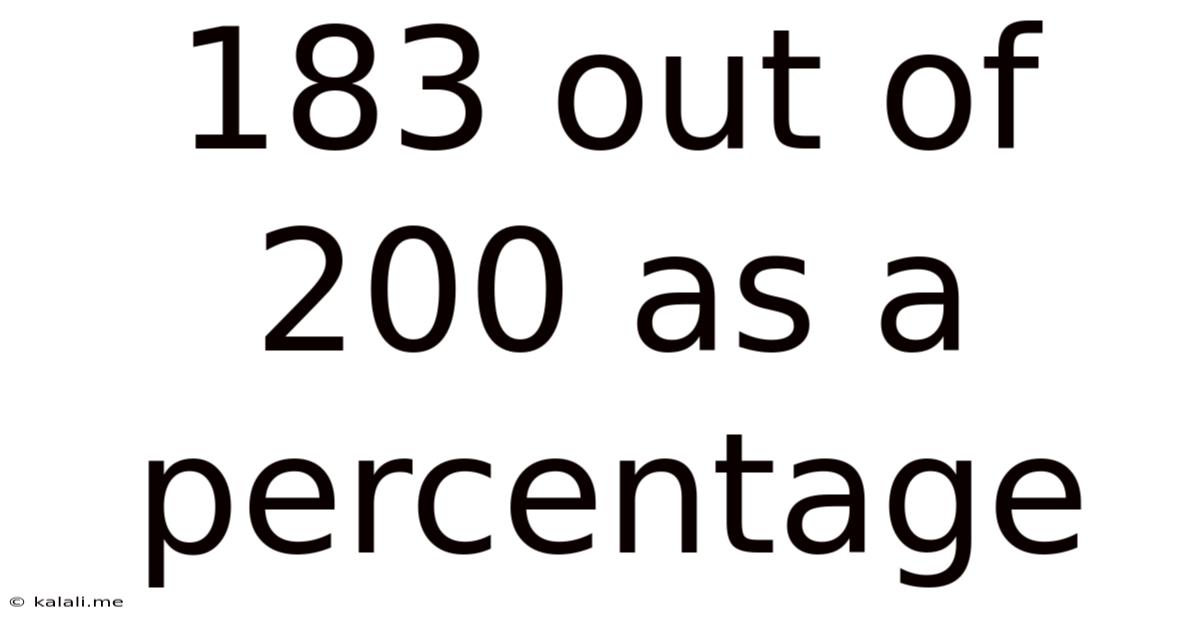
Table of Contents
183 out of 200 as a Percentage: A Comprehensive Guide to Percentage Calculations
Calculating percentages is a fundamental skill with applications across numerous fields, from academic assessments to financial transactions and data analysis. Understanding how to express a portion of a whole as a percentage is crucial for interpreting information and making informed decisions. This article delves into the calculation of 183 out of 200 as a percentage, providing a detailed explanation of the process and exploring various related concepts. We’ll also discuss practical applications and address common misconceptions.
Understanding Percentages
A percentage is a way of expressing a number as a fraction of 100. The symbol "%" represents "per cent," meaning "out of one hundred." For instance, 50% signifies 50 out of 100, which is equivalent to ½ or 0.5. Percentages are used extensively to represent proportions, rates, changes, and comparisons in various contexts.
Calculating 183 out of 200 as a Percentage
To determine what percentage 183 represents out of 200, we employ a straightforward formula:
(Part / Whole) * 100% = Percentage
In this case:
- Part: 183
- Whole: 200
Substituting these values into the formula:
(183 / 200) * 100% = 91.5%
Therefore, 183 out of 200 is 91.5%.
Different Methods for Calculating Percentages
While the above method is the most common and straightforward, there are alternative approaches to calculating percentages, particularly useful for mental calculations or when dealing with simpler fractions:
Method 1: Using Decimals
Convert the fraction to a decimal by dividing the part by the whole:
183 ÷ 200 = 0.915
Then, multiply the decimal by 100 to express it as a percentage:
0.915 * 100% = 91.5%
Method 2: Simplifying Fractions (for simpler cases)
If the fraction can be easily simplified, this can make the calculation quicker. However, this method isn't always practical, especially with larger numbers. For example, if you had 50 out of 100, you could simplify the fraction to ½, which is 50%. This method isn't efficient for 183/200.
Practical Applications of Percentage Calculations
Percentage calculations are indispensable across various domains:
1. Academic Performance:
Students frequently encounter percentage calculations to determine their grades. A score of 183 out of 200 on an exam, as we've calculated, translates to 91.5%, indicating a strong performance.
2. Financial Calculations:
Percentages are crucial in finance for calculating interest rates, discounts, taxes, profit margins, and returns on investment. For example, understanding percentage increases or decreases in stock prices is vital for informed investment decisions.
3. Data Analysis and Statistics:
Percentage calculations are foundational in data analysis for representing data proportions and conducting statistical analyses. Representing survey results, demographics, or experimental outcomes often involves expressing data as percentages.
4. Business and Marketing:
Businesses use percentages to track sales figures, market share, growth rates, and customer satisfaction. Marketing campaigns often analyze their success rates using percentage metrics.
5. Everyday Life:
Percentages are prevalent in everyday life, from calculating tips at restaurants to understanding discounts in stores, and interpreting nutritional information on food labels.
Common Misconceptions about Percentages
Despite the simplicity of the concept, some common misconceptions surrounding percentages can lead to errors:
1. Confusing Percentage Change with Absolute Change:
It's vital to differentiate between percentage change and absolute change. A percentage change reflects the proportional increase or decrease, while the absolute change represents the actual numerical difference. For example, a 10% increase in a $100 investment results in a $10 increase (absolute change), while the percentage change is 10%.
2. Incorrectly Calculating Percentage Increase/Decrease:
When calculating percentage increases or decreases, always base the calculation on the original value. A common error is to base the calculation on the new value, leading to inaccurate results.
3. Misinterpreting Percentages in Context:
The interpretation of percentages should always consider the context. A high percentage in one situation might not necessarily be significant in another. For example, a high percentage of customer satisfaction might be expected for a luxury brand but less so for a budget-friendly option.
Advanced Percentage Calculations: Beyond the Basics
While calculating 183 out of 200 is a straightforward example, percentage calculations can become more complex depending on the context. Here are some advanced scenarios:
1. Calculating Percentage Points:
Percentage points represent the absolute difference between two percentages. For instance, if the interest rate rises from 5% to 8%, the increase is 3 percentage points, not 3%.
2. Compounding Percentages:
When percentages are applied repeatedly over time, the concept of compounding comes into play. Compound interest is a classic example, where interest earned is added to the principal, and subsequent interest calculations are based on the increased amount.
3. Weighted Averages:
In situations with different weights or importance assigned to various components, weighted averages are calculated using percentages to determine the overall average.
4. Percentage Change Over Multiple Periods:
Calculating percentage changes over multiple periods requires careful consideration of the base value for each calculation to avoid inaccuracies.
Conclusion
Mastering percentage calculations is essential for navigating various aspects of life, from academic pursuits to professional endeavors and personal finance. Understanding the fundamental formula, exploring different calculation methods, and being aware of common misconceptions will ensure accurate and efficient percentage calculations. The calculation of 183 out of 200 as 91.5% serves as a clear example of the simple yet powerful nature of percentage calculations, highlighting their widespread applicability and importance in numerical literacy. Remember to always check your work and consider the context when interpreting percentage values.
Latest Posts
Latest Posts
-
How Many Seconds Are In 45 Minutes
Apr 07, 2025
-
24 Meters Is How Many Feet
Apr 07, 2025
-
The Most Numerous Cells Of Blood Are
Apr 07, 2025
-
How Many Inches Is 1 8 M
Apr 07, 2025
-
What Is The Percentage Of 16 25
Apr 07, 2025
Related Post
Thank you for visiting our website which covers about 183 Out Of 200 As A Percentage . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.