2 Pairs Of Parallel Sides And 4 Right Angles
Kalali
Mar 31, 2025 · 6 min read
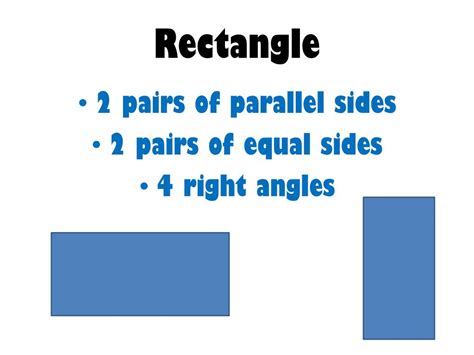
Table of Contents
2 Pairs of Parallel Sides and 4 Right Angles: Exploring the World of Rectangles
The simple statement "2 pairs of parallel sides and 4 right angles" immediately conjures up an image in our minds: a rectangle. This seemingly basic geometric shape is far more significant than its simplicity suggests, underlying countless aspects of our built environment and playing a crucial role in various mathematical concepts. This article delves into the fascinating world of rectangles, exploring their properties, applications, and relationships to other geometric figures.
Defining the Rectangle: More Than Just a Shape
A rectangle, in its purest form, is a quadrilateral – a polygon with four sides – possessing two defining characteristics:
-
Two pairs of parallel sides: This means that opposite sides are parallel to each other. This parallelism is fundamental to the rectangle's stability and the predictable relationships between its sides and angles.
-
Four right angles: Each interior angle of a rectangle measures exactly 90 degrees. This right-angled property is what gives rectangles their distinctive shape and makes them so useful in construction and design.
These two defining features differentiate a rectangle from other quadrilaterals like squares, parallelograms, and trapezoids. While a square is a special type of rectangle (with all sides equal), a parallelogram only requires parallel opposite sides (not necessarily right angles), and a trapezoid only needs one pair of parallel sides.
Understanding the Properties of Rectangles
Beyond the defining characteristics, several other properties stem from the parallel sides and right angles:
-
Opposite sides are equal in length: Because of the parallel sides and right angles, opposite sides are congruent (equal in length). This property is crucial for calculations involving area and perimeter.
-
Diagonals bisect each other: The diagonals of a rectangle (lines connecting opposite corners) intersect at their midpoints. This means each diagonal is divided into two equal segments.
-
Diagonals are equal in length: Unlike other quadrilaterals, the diagonals of a rectangle are congruent. This is a direct consequence of the right angles and the equal length of opposite sides.
-
Area Calculation: The area of a rectangle is simply calculated by multiplying the length by the width: Area = length × width. This straightforward formula is widely used in various fields, from interior design to land surveying.
-
Perimeter Calculation: The perimeter, or the total distance around the rectangle, is calculated by adding up all four sides: Perimeter = 2 × (length + width). This formula is fundamental for calculating the amount of material needed for framing or fencing.
Rectangles in the Real World: Ubiquitous Applications
Rectangles are not just abstract geometric shapes; they are fundamental to our everyday lives. Their prevalence stems from their inherent stability and ease of construction. Here are some examples of their widespread applications:
Architecture and Construction:
-
Buildings: Many buildings, from houses to skyscrapers, incorporate rectangular shapes extensively. Walls, doors, windows, and floors are frequently rectangular, creating a structured and predictable design.
-
Rooms: Most rooms in buildings are rectangular, offering efficient use of space and simplifying construction. This rectangular layout makes furniture placement and interior design straightforward.
-
Bricks and Blocks: Standard building materials, such as bricks and concrete blocks, are typically rectangular, facilitating efficient stacking and construction.
-
Furniture: Tables, desks, beds, and many other pieces of furniture are often rectangular, reflecting the adaptability and practicality of the shape.
Everyday Objects:
-
Screens: Computer monitors, televisions, and smartphone screens are usually rectangular, providing a convenient viewing area.
-
Paper and Books: Standard paper sizes (like A4 and letter) are rectangular, making printing, writing, and organization straightforward. Books, built from rectangular pages, are a fundamental part of human knowledge dissemination.
-
Playing Cards and Game Boards: Many games use rectangular playing cards or game boards, providing a defined and easily understood playing space.
-
Food Packaging: Many food items, from cereal boxes to pizza boxes, are packaged in rectangular containers for ease of stacking, transportation, and display.
Other Applications:
-
Art and Design: Rectangles are commonly used in graphic design, painting, and photography to create balanced and visually appealing compositions.
-
Maps and Charts: Rectangular grids are often used in maps and charts to represent geographical areas or data sets effectively.
-
Agriculture: Farmlands are often divided into rectangular plots for efficient planting and harvesting.
Rectangles and Other Geometric Shapes: Interrelationships
Rectangles are closely related to several other geometric figures, often serving as special cases or forming part of more complex shapes.
Squares: A Special Case of Rectangles
A square is a rectangle where all four sides are equal in length. It retains all the properties of a rectangle, but adds the additional property of equal side lengths. This makes squares highly symmetrical and particularly useful in tiling patterns and tessellations.
Parallelograms: Broader Family Member
A parallelogram is a quadrilateral with two pairs of parallel sides. Rectangles are a special type of parallelogram, specifically those with four right angles. Parallelograms, in general, do not have right angles and might have unequal side lengths.
Rhombuses: Another Relative
A rhombus is a quadrilateral with all four sides equal in length. While it shares the equal side length property with squares, a rhombus doesn't necessarily have right angles. A square is the only shape that is both a rhombus and a rectangle.
Trapezoids: Distant Cousins
A trapezoid (or trapezium) has only one pair of parallel sides. It's a more general quadrilateral and has less in common with rectangles, other than being a four-sided polygon.
Exploring Rectangles Through Geometry Theorems
Numerous geometric theorems revolve around rectangles, further showcasing their significance in mathematical understanding:
-
The Pythagorean Theorem: While not exclusively about rectangles, the Pythagorean theorem (a² + b² = c²) is crucial for calculating the length of a rectangle's diagonal, given its length and width.
-
Area and Perimeter Theorems: The theorems related to calculating area and perimeter are specific to rectangles, providing fundamental formulas for various applications.
-
Angle Sum Theorem: The angle sum theorem for quadrilaterals states that the sum of interior angles in any quadrilateral is 360°. Rectangles perfectly illustrate this theorem, with their four 90-degree angles adding up to 360°.
Rectangles in Advanced Mathematics:
Rectangles play a significant role in more advanced mathematical concepts. For example:
-
Calculus: Rectangles are used in Riemann sums to approximate the area under a curve, forming the foundation for integral calculus.
-
Linear Algebra: Rectangles and their properties are fundamental in understanding matrices and vectors.
-
Coordinate Geometry: Rectangles are used to define coordinate systems and help represent geometric shapes algebraically.
Conclusion: The Enduring Importance of Rectangles
The seemingly simple rectangle, defined by just two pairs of parallel sides and four right angles, is a shape of immense practical and theoretical significance. Its inherent stability, ease of construction, and predictable properties have made it a cornerstone of architecture, design, and various mathematical fields. From the buildings that house us to the screens we use, rectangles are an integral part of our world, demonstrating the enduring power of fundamental geometric shapes. Their ubiquity highlights the importance of understanding their properties and the diverse ways they contribute to our lives and our comprehension of the mathematical universe.
Latest Posts
Latest Posts
-
How Many Cups Is 33 Oz
Apr 02, 2025
-
Cuanto Es 10 Millas En Kilometros
Apr 02, 2025
-
How Many Cm Is 71 Inches
Apr 02, 2025
-
How Tall Is 65inches In Feet
Apr 02, 2025
-
Least Common Multiple Of 5 And 9
Apr 02, 2025
Related Post
Thank you for visiting our website which covers about 2 Pairs Of Parallel Sides And 4 Right Angles . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.
