20 Is What Percent Of 80
Kalali
Apr 11, 2025 · 5 min read
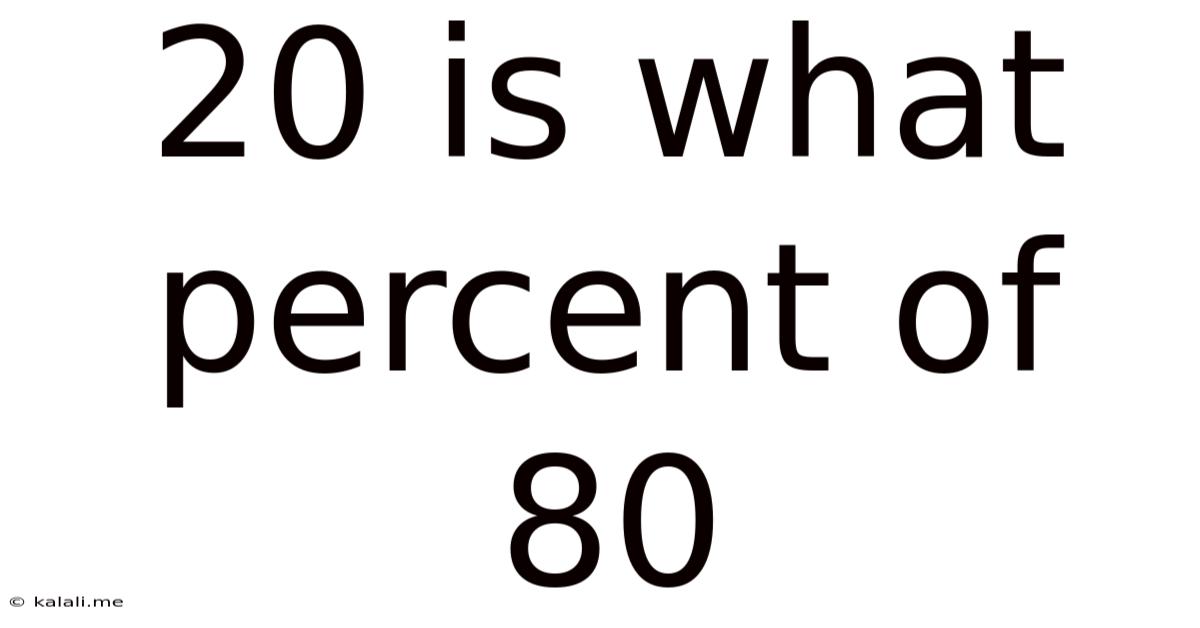
Table of Contents
20 is What Percent of 80: A Comprehensive Guide to Percentage Calculations
Calculating percentages is a fundamental skill applicable across numerous fields, from everyday budgeting and shopping to complex scientific research and financial analysis. Understanding how to solve percentage problems, like "20 is what percent of 80?", is crucial for navigating these situations confidently. This comprehensive guide will not only answer this specific question but also equip you with the knowledge and techniques to tackle any percentage calculation. We'll delve into the core concepts, explore multiple solution methods, and provide real-world examples to solidify your understanding.
What this article covers:
- Understanding Percentages and their Applications
- Three Methods for Solving "20 is What Percent of 80?"
- Practical Applications of Percentage Calculations
- Common Percentage Calculation Mistakes and How to Avoid Them
- Advanced Percentage Problems and Techniques
- Resources for Further Learning
Understanding Percentages and their Applications:
A percentage is a way of expressing a number as a fraction of 100. The term "percent" comes from the Latin words "per centum," meaning "out of one hundred." Percentages are incredibly versatile and used extensively in:
- Finance: Calculating interest rates, discounts, profits, and losses.
- Statistics: Representing data in charts and graphs, analyzing survey results.
- Science: Expressing concentrations, error margins, and experimental yields.
- Everyday Life: Determining sales tax, tips, and calculating discounts on purchases.
Mastering percentage calculations empowers you to make informed decisions, analyze data effectively, and solve various problems with confidence.
Three Methods for Solving "20 is What Percent of 80?"
There are several ways to determine what percentage 20 represents of 80. Let's explore three common methods:
Method 1: The Proportion Method
This method uses the concept of ratios and proportions. We can set up a proportion:
x/100 = 20/80
Where 'x' represents the unknown percentage. To solve for 'x', we cross-multiply:
80x = 20 * 100
80x = 2000
x = 2000 / 80
x = 25
Therefore, 20 is 25% of 80.
Method 2: The Decimal Method
This method involves converting the fraction to a decimal and then multiplying by 100 to express it as a percentage.
First, express the relationship as a fraction:
20/80
Then, simplify the fraction:
20/80 = 1/4
Now, convert the fraction to a decimal by dividing the numerator by the denominator:
1 ÷ 4 = 0.25
Finally, multiply the decimal by 100 to express it as a percentage:
0.25 * 100 = 25%
Therefore, 20 is 25% of 80.
Method 3: The Formula Method
This method uses a straightforward formula:
(Part / Whole) * 100 = Percentage
In our case:
(20 / 80) * 100 = 25%
This formula directly calculates the percentage by dividing the part (20) by the whole (80) and then multiplying by 100. This method is highly efficient and easily adaptable for various percentage problems.
Practical Applications of Percentage Calculations:
Let's illustrate the practical use of percentage calculations with some real-world examples:
-
Sales Discounts: A store offers a 20% discount on an item originally priced at $80. Using the formula, we calculate the discount: (20/100) * $80 = $16. The final price after the discount would be $80 - $16 = $64.
-
Calculating Tips: You want to leave a 15% tip on a meal costing $60. The tip amount is (15/100) * $60 = $9.
-
Understanding Interest Rates: If you invest $1000 at an annual interest rate of 5%, you'll earn (5/100) * $1000 = $50 in interest after one year.
-
Analyzing Test Scores: If you scored 45 out of 50 on a test, your percentage score is (45/50) * 100 = 90%.
-
Determining Tax Amounts: A 6% sales tax on a $50 purchase amounts to (6/100) * $50 = $3.
Common Percentage Calculation Mistakes and How to Avoid Them:
Several common errors can occur when calculating percentages. Here are some to watch out for:
-
Incorrect Fraction Setup: Ensure you correctly identify the "part" and the "whole" when setting up the fraction. The "part" is the value you're expressing as a percentage of the "whole."
-
Decimal Point Errors: Be careful when converting decimals to percentages and vice versa. Remember to multiply by 100 when converting decimals to percentages and divide by 100 when converting percentages to decimals.
-
Misinterpreting the Problem: Carefully read the problem statement to understand what is being asked. Determine what value represents the whole and what represents the part.
-
Rounding Errors: Be mindful of rounding errors, especially when dealing with multiple percentage calculations. Round to the appropriate number of decimal places based on the context.
To avoid these errors, always double-check your work, use a calculator when necessary, and understand the context of the problem before beginning your calculations.
Advanced Percentage Problems and Techniques:
Beyond the basic calculations, more complex scenarios involving percentages exist:
-
Percentage Increase/Decrease: Calculating the percentage change between two values. The formula is:
((New Value - Old Value) / Old Value) * 100. -
Compound Interest: Calculating interest earned on both the principal amount and accumulated interest. This requires understanding exponential growth.
-
Percentage Points vs. Percentages: Understanding the difference between a percentage point change and a percentage change. A percentage point change is a direct difference between two percentages, while a percentage change reflects the relative change.
-
Successive Percentages: Calculating the overall percentage change after multiple consecutive percentage changes. This often involves multiplying the decimal equivalents of each percentage change.
Resources for Further Learning:
Numerous online resources can help you further develop your understanding of percentage calculations. Explore educational websites and online calculators to practice different problems and deepen your knowledge. Khan Academy, for example, offers excellent tutorials on percentages and other mathematical concepts.
In conclusion, understanding how to calculate percentages is an essential skill with widespread applications. By mastering the methods outlined in this article – the proportion method, the decimal method, and the formula method – and by avoiding common mistakes, you'll be well-equipped to tackle any percentage problem with confidence. Remember to practice regularly and explore additional resources to further enhance your proficiency. The ability to confidently calculate and interpret percentages will undoubtedly benefit you in numerous aspects of your personal and professional life.
Latest Posts
Latest Posts
-
How Long Does It Take To Drive 2000 Miles
Jul 18, 2025
-
How Many Grams In A Teaspoon Of Cinnamon
Jul 18, 2025
-
How Long To Heat Water In Microwave
Jul 18, 2025
-
40 Oz Of Water Is How Many Cups
Jul 18, 2025
-
How Many Eighths In A Quarter Pound
Jul 18, 2025
Related Post
Thank you for visiting our website which covers about 20 Is What Percent Of 80 . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.