27 Is What Percent Of 30
Kalali
Apr 15, 2025 · 5 min read
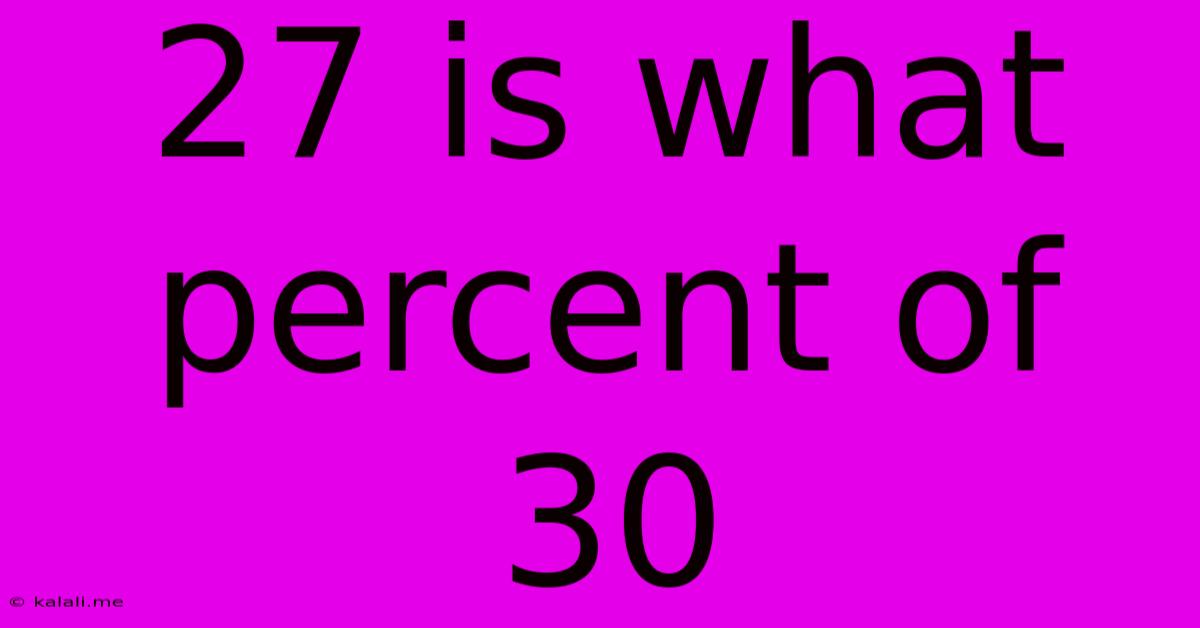
Table of Contents
27 is What Percent of 30? A Comprehensive Guide to Percentage Calculations
Understanding percentages is a fundamental skill with broad applications in everyday life, from calculating discounts and taxes to analyzing data and understanding financial reports. This article will delve into the question, "27 is what percent of 30?", providing a step-by-step solution, exploring different calculation methods, and expanding on the broader concept of percentage calculations and their real-world relevance. We will cover various approaches, including using proportions, decimals, and the common percentage formula, ensuring a complete understanding for all readers.
Meta Description: Learn how to calculate percentages with this in-depth guide. We solve the problem "27 is what percent of 30?" using multiple methods, providing a comprehensive understanding of percentage calculations and their real-world applications.
Understanding the Fundamentals of Percentages
A percentage is a fraction or ratio expressed as a number out of 100. It represents a portion of a whole. The symbol "%" is used to denote percentages. For instance, 50% means 50 out of 100, or 50/100, which simplifies to 1/2. Understanding this fundamental concept is crucial before tackling percentage calculations.
Method 1: Using Proportions to Solve "27 is What Percent of 30?"
Proportions offer a clear and intuitive way to solve percentage problems. We can set up a proportion to represent the problem:
- Part / Whole = Percentage / 100
In our case:
- 27 / 30 = x / 100
Where 'x' represents the percentage we need to find. To solve for x, we cross-multiply:
- 27 * 100 = 30 * x
- 2700 = 30x
- x = 2700 / 30
- x = 90
Therefore, 27 is 90% of 30.
Method 2: Using Decimals to Solve "27 is What Percent of 30?"
This method involves converting the fraction to a decimal and then multiplying by 100 to obtain the percentage.
First, express the relationship as a fraction:
- 27 / 30
Next, convert the fraction to a decimal by performing the division:
- 27 ÷ 30 = 0.9
Finally, multiply the decimal by 100 to express it as a percentage:
- 0.9 * 100 = 90%
Again, we confirm that 27 is 90% of 30.
Method 3: The Percentage Formula
The standard percentage formula can also be used:
- Percentage = (Part / Whole) * 100
Substituting the values from our problem:
- Percentage = (27 / 30) * 100
- Percentage = 0.9 * 100
- Percentage = 90%
This method reinforces the result: 27 is 90% of 30.
Real-World Applications of Percentage Calculations
The ability to calculate percentages is essential in various real-world scenarios:
- Sales and Discounts: Calculating discounts offered on products during sales events. For example, a 20% discount on a $100 item.
- Taxes: Determining the amount of sales tax or income tax payable.
- Finance: Calculating interest rates on loans or investments. Understanding APR (Annual Percentage Rate) is crucial for financial planning.
- Statistics and Data Analysis: Percentages are used extensively to represent proportions and trends in data sets, allowing for easier interpretation of complex information. For instance, understanding market share percentages or the percentage change in sales figures.
- Tip Calculation: Determining the appropriate tip amount at a restaurant.
- Grading Systems: Many educational institutions use percentages to represent student grades and performance.
Expanding on Percentage Calculations: Finding the Whole or the Part
While the initial problem focused on finding the percentage, percentage calculations can also be used to find the whole or the part given the percentage and either the whole or the part.
-
Finding the Whole: If you know the part and the percentage, you can find the whole. For example: "15 is 25% of what number?" This can be solved using the formula: Whole = (Part / Percentage) * 100.
-
Finding the Part: If you know the whole and the percentage, you can find the part. For example: "What is 15% of 200?" This is solved using the formula: Part = (Percentage / 100) * Whole.
Advanced Percentage Calculations: Percentage Change and Percentage Increase/Decrease
Beyond basic percentage calculations, understanding percentage change is vital for analyzing trends and growth.
-
Percentage Change: This measures the relative change between an old and a new value. The formula is: Percentage Change = [(New Value - Old Value) / Old Value] * 100. A positive result indicates an increase, while a negative result indicates a decrease.
-
Percentage Increase/Decrease: These are specific instances of percentage change, highlighting the increase or decrease from an initial value.
Tips for Mastering Percentage Calculations
- Practice Regularly: The more you practice, the more comfortable you'll become with these calculations. Try different variations of problems to solidify your understanding.
- Use Multiple Methods: Experiment with the different methods outlined above (proportions, decimals, and the formula) to find the approach that works best for you.
- Understand the Concepts: Don't just memorize formulas; make sure you understand the underlying concepts of percentages, fractions, and ratios.
- Check Your Answers: Always double-check your work to ensure accuracy.
- Use Calculators Wisely: While calculators can be helpful, relying on them too heavily can hinder your understanding of the process. Try to solve problems manually before using a calculator.
Conclusion: The Power of Percentage Understanding
Understanding percentage calculations is a crucial life skill with wide-ranging applications. By mastering these calculations, you’ll be better equipped to handle various tasks, from managing your finances to interpreting data and making informed decisions. The problem "27 is what percent of 30?" serves as a simple yet illustrative example of how percentage calculations can be applied, emphasizing the importance of grasping these fundamental mathematical concepts. Through consistent practice and a thorough understanding of the methods presented, you can confidently tackle any percentage-related problem you encounter. Remember to always focus on understanding the underlying principles, and you’ll find percentage calculations become much easier and more intuitive.
Latest Posts
Latest Posts
-
How Much Is 48 Oz Of Water
Jul 18, 2025
-
How Long Does It Take To Drive 2000 Miles
Jul 18, 2025
-
How Many Grams In A Teaspoon Of Cinnamon
Jul 18, 2025
-
How Long To Heat Water In Microwave
Jul 18, 2025
-
40 Oz Of Water Is How Many Cups
Jul 18, 2025
Related Post
Thank you for visiting our website which covers about 27 Is What Percent Of 30 . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.