3 Of 16 Is What Percent
Kalali
Mar 31, 2025 · 5 min read
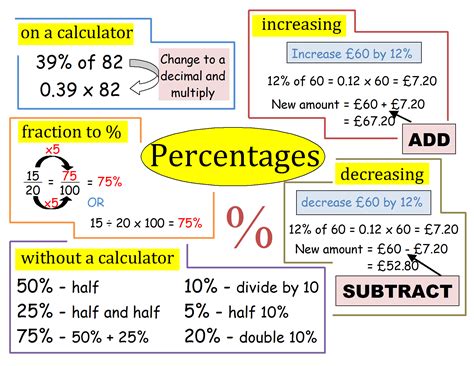
Table of Contents
3 of 16 is What Percent? A Comprehensive Guide to Percentage Calculations
Understanding percentages is a fundamental skill in many aspects of life, from calculating discounts and taxes to analyzing data and understanding statistics. This comprehensive guide will delve into the question "3 of 16 is what percent?" and provide you with a thorough understanding of the underlying principles and various methods for solving percentage problems. We'll explore different approaches, from the basic formula to more advanced techniques, ensuring you can confidently tackle similar percentage calculations in the future.
Understanding the Fundamentals of Percentages
Before we dive into the specifics of calculating "3 of 16 is what percent?", let's solidify our understanding of percentages. A percentage is simply a fraction expressed as a part of 100. The symbol "%" represents "per hundred" or "out of 100".
For example, 50% means 50 out of 100, which can be simplified to 1/2 or 0.5 as a decimal. Understanding this core concept is crucial for tackling any percentage calculation.
Method 1: Using the Basic Percentage Formula
The most straightforward method to determine what percentage 3 is of 16 is using the fundamental percentage formula:
(Part / Whole) * 100% = Percentage
In our case:
- Part: 3 (the number we're interested in)
- Whole: 16 (the total number)
Let's plug these values into the formula:
(3 / 16) * 100% = 18.75%
Therefore, 3 out of 16 is 18.75%.
Method 2: Converting the Fraction to a Decimal
Another approach involves converting the fraction 3/16 into a decimal and then multiplying by 100% to obtain the percentage.
-
Convert the fraction to a decimal: Divide 3 by 16: 3 ÷ 16 = 0.1875
-
Multiply by 100%: 0.1875 * 100% = 18.75%
This method yields the same result as the previous one: 18.75%.
Method 3: Using Proportions
We can also solve this problem using proportions. A proportion is a statement that two ratios are equal. We can set up a proportion to find the unknown percentage (x):
3/16 = x/100
To solve for x, we cross-multiply:
16x = 300
x = 300/16
x = 18.75
Therefore, x = 18.75%.
Practical Applications: Real-World Examples
Understanding percentage calculations is essential in numerous real-world scenarios. Let's explore some examples where this knowledge proves invaluable:
1. Sales and Discounts:
Imagine a store is offering a discount of 18.75% on an item. If the original price is $16, you can calculate the discount amount using the percentage we derived earlier:
Discount amount = 18.75% of $16 = (18.75/100) * $16 = $3
The final price after the discount would be $16 - $3 = $13.
2. Test Scores and Grades:
If you answered 3 out of 16 questions correctly on a test, your score would be 18.75%. This allows you to understand your performance relative to the total number of questions.
3. Financial Calculations:
Percentage calculations are frequently used in financial matters, such as calculating interest rates, returns on investments, and profit margins.
4. Data Analysis and Statistics:
In statistical analysis, percentages are used to represent proportions and to make comparisons within datasets. For example, if 3 out of 16 participants in a survey responded positively, this translates to an 18.75% positive response rate.
Expanding Your Understanding: More Complex Percentage Problems
While the problem "3 of 16 is what percent?" is relatively simple, it serves as a building block for understanding more complex percentage problems. Here are some examples:
-
Finding the whole when the part and percentage are known: If you know that 18.75% of a number is 3, how can you find the total number? This involves rearranging the basic percentage formula.
-
Finding the part when the whole and percentage are known: If you know that 25% of a number is 4, how can you determine the value of the 'part'?
-
Calculating percentage increase or decrease: Understanding how to calculate percentage changes is essential when comparing data over time or analyzing trends.
-
Compound interest calculations: Compound interest involves calculating interest on both the principal and accumulated interest. Percentage calculations form the core of this concept.
Mastering Percentages: Tips and Tricks
Here are some tips and tricks to help you master percentage calculations:
-
Practice regularly: The more you practice, the more confident you'll become.
-
Use different methods: Try all three methods (formula, decimal conversion, and proportions) to find the one that suits your understanding and problem-solving style.
-
Understand the concept, not just the formula: Grasp the fundamental idea of percentages as parts of 100.
-
Use online calculators and tools: While understanding the underlying principles is crucial, online calculators can be helpful for quick calculations and checking your answers.
-
Break down complex problems: Divide complex problems into smaller, manageable steps.
Conclusion: The Power of Percentage Calculations
The ability to accurately and efficiently calculate percentages is a valuable skill applicable across numerous disciplines. By understanding the basic formula, alternative methods, and practical applications, you can confidently tackle percentage problems of varying complexity. This guide has not only answered the question "3 of 16 is what percent?" but has also provided a strong foundation for tackling future percentage challenges. Remember to practice consistently to solidify your understanding and build confidence in your ability to work with percentages. The more you work with them, the more intuitive and effortless these calculations will become. Mastering percentages is a key step in developing strong numerical reasoning skills, enhancing your problem-solving abilities, and furthering your success in various academic and professional pursuits.
Latest Posts
Latest Posts
-
How Long Does It Take A Fossil To Form
Apr 02, 2025
-
How Many Inches Is In 15 Cm
Apr 02, 2025
-
How Much Water In 2 Quarts
Apr 02, 2025
-
What Is 8 5 Cm In Inches
Apr 02, 2025
-
42 Feet Is How Many Meters
Apr 02, 2025
Related Post
Thank you for visiting our website which covers about 3 Of 16 Is What Percent . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.
