36 Out Of 40 As A Percentage
Kalali
Mar 08, 2025 · 5 min read
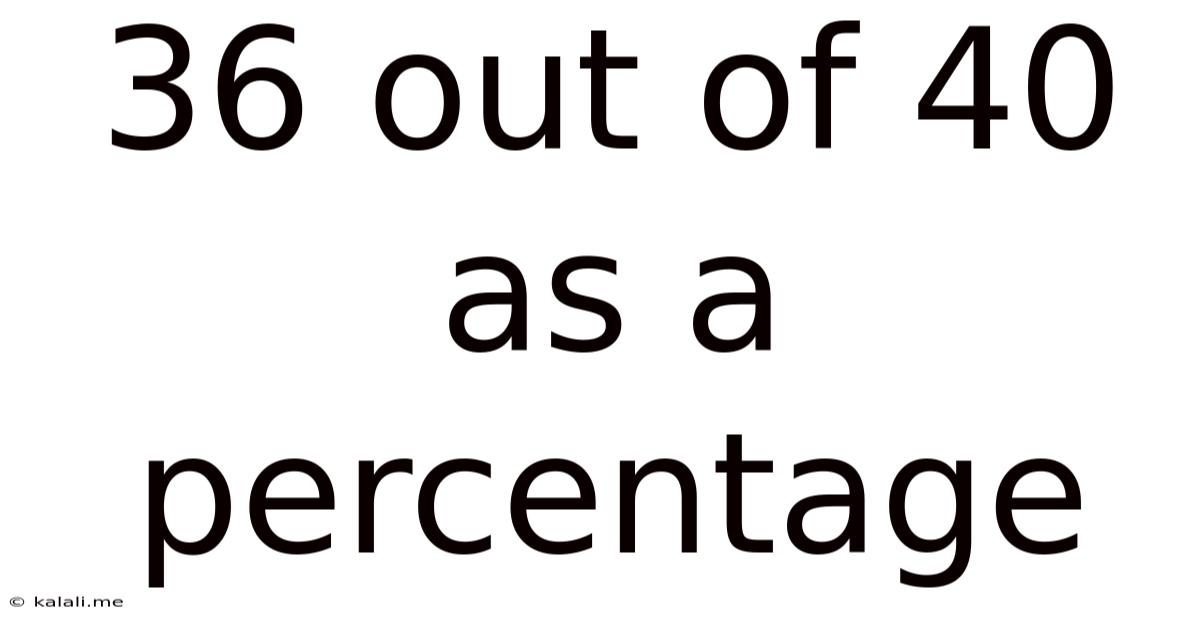
Table of Contents
36 out of 40 as a Percentage: A Comprehensive Guide
Calculating percentages is a fundamental skill applicable across numerous fields, from academic assessments to financial analyses. Understanding how to convert fractions into percentages is crucial for interpreting data and making informed decisions. This comprehensive guide delves into the calculation of 36 out of 40 as a percentage, providing various methods, practical applications, and insights into percentage calculations in general.
Understanding Percentages
Before diving into the specifics of 36 out of 40, let's solidify the foundational concept of percentages. A percentage represents a fraction of 100. The word "percent" itself derives from the Latin "per centum," meaning "out of a hundred." Therefore, any percentage can be expressed as a fraction with a denominator of 100. For example, 50% is equivalent to 50/100, which simplifies to 1/2.
Calculating 36 out of 40 as a Percentage: Method 1 (Fraction to Percentage)
The most straightforward method involves converting the given fraction (36/40) into a percentage. Here's a step-by-step guide:
-
Express as a Fraction: The problem "36 out of 40" is represented as the fraction 36/40.
-
Convert to Decimal: Divide the numerator (36) by the denominator (40): 36 ÷ 40 = 0.9
-
Multiply by 100: To express the decimal as a percentage, multiply by 100: 0.9 x 100 = 90
-
Add the Percentage Symbol: Finally, add the percentage symbol (%) to obtain the final answer: 90%
Therefore, 36 out of 40 is equal to 90%.
Calculating 36 out of 40 as a Percentage: Method 2 (Simplifying the Fraction)
This method simplifies the fraction before converting it to a percentage. This approach can be helpful when dealing with larger numbers or fractions that can be easily simplified.
-
Simplify the Fraction: Find the greatest common divisor (GCD) of 36 and 40, which is 4. Divide both the numerator and denominator by 4: 36 ÷ 4 = 9 and 40 ÷ 4 = 10. This simplifies the fraction to 9/10.
-
Convert to Decimal: Divide the numerator (9) by the denominator (10): 9 ÷ 10 = 0.9
-
Multiply by 100: Multiply the decimal by 100: 0.9 x 100 = 90
-
Add the Percentage Symbol: Add the percentage symbol: 90%
This method yields the same result: 90%.
Practical Applications of Percentage Calculations
Understanding percentage calculations is vital in various real-world scenarios. Here are some examples:
-
Academic Performance: If a student answers 36 out of 40 questions correctly on a test, their score is 90%. This is a common application of percentage calculations.
-
Sales and Discounts: Stores often advertise discounts as percentages. For example, a "20% off" sale means you pay 80% of the original price.
-
Financial Investments: Percentage calculations are fundamental in understanding investment returns, interest rates, and profit margins.
-
Data Analysis: Percentages are frequently used to represent proportions and trends in data analysis, making complex information easier to understand.
-
Surveys and Polls: Survey results are often expressed as percentages to represent the proportion of respondents who chose a particular answer.
Beyond the Basics: Working with Percentages
While calculating 36 out of 40 is relatively straightforward, mastering percentage calculations involves understanding several related concepts:
-
Finding the Percentage of a Number: To find a percentage of a number, multiply the number by the percentage (expressed as a decimal). For example, 20% of 50 is 50 x 0.20 = 10.
-
Finding the Percentage Increase or Decrease: To calculate percentage change, find the difference between the original value and the new value, divide the difference by the original value, and multiply by 100. For example, if a price increases from $100 to $120, the percentage increase is ((120-100)/100) x 100 = 20%.
-
Working with Percentages and Fractions: Remember that percentages can always be expressed as fractions (e.g., 25% = 1/4) and decimals (e.g., 25% = 0.25). Understanding these interrelationships is crucial for solving complex percentage problems.
-
Using a Calculator: Calculators are invaluable tools for percentage calculations, especially when dealing with more complex or numerous calculations. Many calculators have a dedicated percentage function.
Common Mistakes to Avoid When Calculating Percentages
While calculating percentages might seem simple, several common mistakes can lead to inaccurate results:
-
Incorrect Order of Operations: Remember to follow the correct order of operations (PEMDAS/BODMAS) when calculating percentages, especially when dealing with multiple operations.
-
Misinterpreting the Question: Carefully read the question to understand what is being asked. Ensure you are calculating the correct percentage.
-
Rounding Errors: Rounding off numbers too early in the calculation can lead to significant errors, particularly when dealing with multiple steps. Try to retain as many decimal places as possible until the final answer.
-
Using the Wrong Formula: Choose the appropriate formula based on the specific calculation required.
Advanced Applications and Real-World Scenarios
The fundamental understanding of calculating percentages, as demonstrated with 36 out of 40, extends to more complex scenarios:
-
Compound Interest: Calculating compound interest involves repeatedly applying percentage calculations over time. This is crucial for understanding savings accounts, loans, and investments.
-
Statistical Analysis: Percentages are widely used in statistical analysis to represent proportions, probabilities, and confidence intervals.
-
Budgeting and Finance: Budgeting requires extensive use of percentages to allocate funds, track spending, and project future expenses.
-
Data Visualization: Percentages are often used in charts and graphs to represent data visually, making trends and patterns easier to understand.
Conclusion: Mastering Percentage Calculations
Calculating 36 out of 40 as a percentage, resulting in 90%, is a simple yet fundamental demonstration of percentage calculations. Mastering this skill extends beyond simple calculations and becomes vital in numerous academic, professional, and personal situations. By understanding the methods, applications, and potential pitfalls, you can confidently tackle a wide range of percentage-related problems and confidently interpret data presented in percentage form. Remember to practice regularly and utilize available resources like calculators and online tutorials to refine your skills and enhance your understanding of this crucial mathematical concept. The ability to efficiently and accurately calculate percentages is a skill that will undoubtedly benefit you throughout your life.
Latest Posts
Latest Posts
-
700 Is 10 Times As Much As
Jul 12, 2025
-
How Much Is 120 Kilograms In Pounds
Jul 12, 2025
-
Born In 1990 How Old Am I
Jul 12, 2025
-
What Is The Average Iq For A 5 Year Old
Jul 12, 2025
-
How Many 12 Oz Cups In A Gallon
Jul 12, 2025
Related Post
Thank you for visiting our website which covers about 36 Out Of 40 As A Percentage . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.