4 Out Of 6 In Percentage
Kalali
Apr 18, 2025 · 5 min read
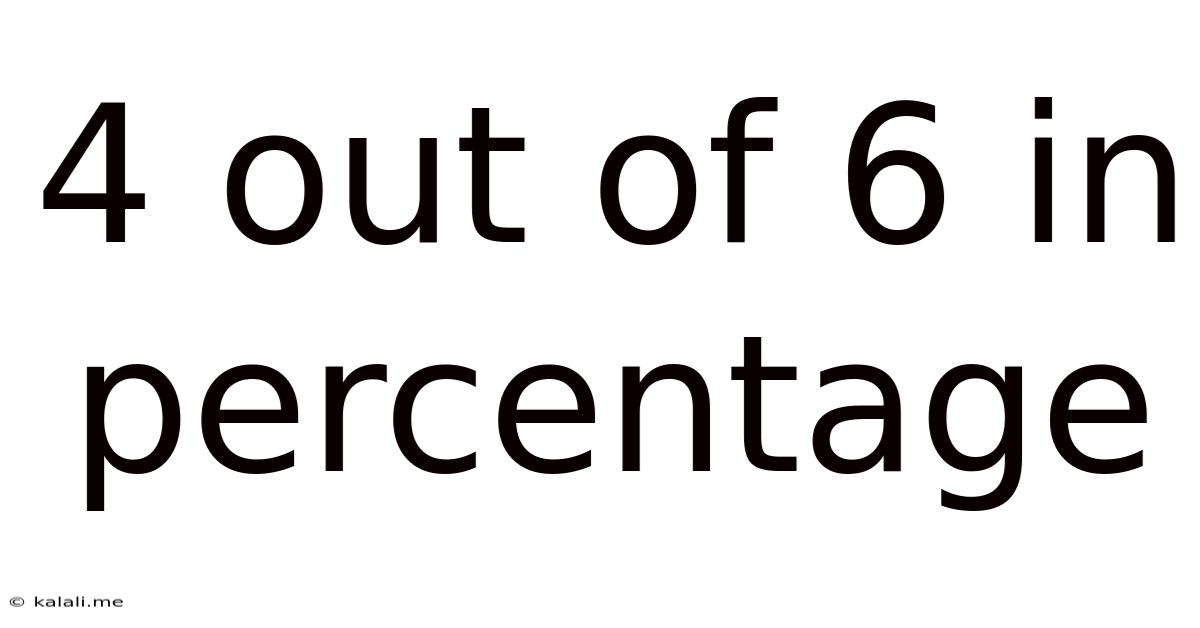
Table of Contents
Decoding the 4 out of 6 Percentage: A Comprehensive Guide
Meta Description: Understanding how to calculate "4 out of 6" as a percentage is crucial in various contexts. This guide provides a step-by-step explanation, explores different methods, and offers practical applications to help you master percentage calculations. We'll also delve into related concepts like ratios, proportions, and applications in real-world scenarios.
Calculating percentages is a fundamental skill with widespread applications, from academic assessments to financial analysis and everyday decision-making. One common scenario involves determining the percentage representation of a part relative to a whole. This article focuses on comprehensively explaining how to calculate "4 out of 6" as a percentage, providing multiple approaches and discussing related mathematical concepts. Understanding this seemingly simple calculation unlocks a deeper understanding of proportions and percentages, which are vital for numerous situations.
Understanding the Basics: Ratios and Percentages
Before diving into the calculation, let's refresh our understanding of ratios and percentages. A ratio expresses the quantitative relationship between two or more amounts. In our case, the ratio is 4:6, representing 4 successful attempts out of a total of 6 attempts.
A percentage, denoted by the symbol %, represents a fraction of 100. It indicates a proportion of a whole expressed as parts per hundred. Converting a ratio to a percentage allows for easier comparison and interpretation of proportions. For instance, understanding that 66.67% of attempts were successful offers a clearer picture than simply stating a 4:6 ratio.
Method 1: The Fraction Method – The most straightforward approach
The most intuitive way to calculate "4 out of 6" as a percentage is by first expressing it as a fraction.
-
Represent as a Fraction: The ratio 4 out of 6 can be written as the fraction 4/6.
-
Simplify the Fraction (Optional but Recommended): Simplifying the fraction makes the subsequent calculation easier. Both the numerator (4) and the denominator (6) are divisible by 2. Simplifying gives us 2/3.
-
Convert to a Decimal: To convert the fraction to a decimal, divide the numerator by the denominator: 2 ÷ 3 ≈ 0.6667 (We round to four decimal places for accuracy).
-
Convert to a Percentage: Multiply the decimal by 100 to express it as a percentage: 0.6667 x 100 = 66.67%.
Therefore, 4 out of 6 represents 66.67%.
Method 2: The Direct Percentage Calculation
This method avoids the intermediate step of simplifying the fraction and directly calculates the percentage.
-
Set up the Equation: We can set up a proportion: (4/6) = (x/100), where 'x' represents the percentage we want to find.
-
Cross-Multiply: Cross-multiplying gives us 6x = 400.
-
Solve for x: Divide both sides by 6: x = 400/6 ≈ 66.67.
-
Express as a Percentage: Therefore, x ≈ 66.67%, which is the same result as Method 1.
Method 3: Using a Calculator
Most calculators have a percentage function that simplifies the process. Simply enter 4 ÷ 6 and then multiply the result by 100 to obtain the percentage directly. This is the quickest method, especially for more complex calculations.
Understanding the Result: 66.67% - What does it mean?
The result, 66.67%, signifies that 4 represents approximately two-thirds (2/3) of 6. In any context where this calculation is applied, it indicates a successful rate of approximately 66.67%. This could represent a test score, a completion rate of tasks, a survey response rate, or any other situation where a part is compared to a whole.
Practical Applications of Percentage Calculations
The ability to calculate percentages like "4 out of 6" is crucial in numerous real-world scenarios:
-
Academic Performance: Calculating grades, test scores, and assignment marks often involves determining percentages. For example, if a student answered 4 out of 6 questions correctly on a quiz, their score would be 66.67%.
-
Business and Finance: Percentage calculations are fundamental in business analysis, including profit margins, sales growth, market share, and return on investment (ROI).
-
Statistics and Data Analysis: Percentages are used extensively in representing data, making it easier to understand trends and draw conclusions from statistical information.
-
Everyday Life: Calculating discounts, tips, tax rates, and other financial calculations frequently involve percentages.
-
Scientific Research: In scientific experiments, data analysis often involves calculating percentages to express the frequency of certain events or outcomes.
Beyond 4 out of 6: Extending the Concept
The principles discussed here extend to any scenario where you need to calculate a percentage from a given ratio. For example:
- 8 out of 10: 8/10 = 0.8 * 100 = 80%
- 15 out of 25: 15/25 = 3/5 = 0.6 * 100 = 60%
- 3 out of 5: 3/5 = 0.6 * 100 = 60%
Addressing Potential Confusion and Common Mistakes
A common mistake when calculating percentages is to incorrectly place the numerator and denominator. Always remember that the numerator (the top number) represents the part, and the denominator (the bottom number) represents the whole.
Another potential source of error is rounding. While rounding to a convenient number of decimal places is acceptable in many situations, it's essential to maintain accuracy, especially in financial or scientific contexts. Using more decimal places (e.g., 66.6667%) ensures a more precise result.
Advanced Applications and Related Concepts
Understanding percentage calculations opens doors to more complex mathematical concepts, such as:
-
Proportions: The ability to set up and solve proportions is crucial in solving various percentage problems, particularly those involving scaling or proportional relationships.
-
Ratios and Rates: Percentages are closely related to ratios and rates, and understanding their interrelationships enhances problem-solving capabilities.
-
Compound Interest: Understanding percentages is essential for grasping the concept of compound interest, which is critical in financial planning and investment analysis.
-
Statistical Inference: In statistics, percentage calculations form the basis for many inferential techniques used to draw conclusions from sample data.
Conclusion: Mastering the Percentage Calculation
Calculating "4 out of 6" as a percentage – and understanding the underlying principles – equips you with a valuable skill applicable in countless situations. Whether you're dealing with academic grades, financial matters, data analysis, or everyday calculations, the ability to accurately and efficiently determine percentages is an invaluable asset. By mastering the methods outlined in this guide and understanding the related mathematical concepts, you can confidently navigate numerous percentage-related challenges. Remember to practice regularly to solidify your understanding and build proficiency. This will empower you to confidently interpret and utilize percentages in all aspects of your life.
Latest Posts
Latest Posts
-
Cuanto Es 13 Grados Fahrenheit En Centigrados
Apr 19, 2025
-
How Much Is 39 Degrees Celsius In Fahrenheit
Apr 19, 2025
-
37 Inches Is How Many Centimeters
Apr 19, 2025
-
75 Is What Percent Of 25
Apr 19, 2025
-
What Does The Circle In A Triangle Mean
Apr 19, 2025
Related Post
Thank you for visiting our website which covers about 4 Out Of 6 In Percentage . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.