40 Is What Percent Of 160
Kalali
Apr 03, 2025 · 5 min read
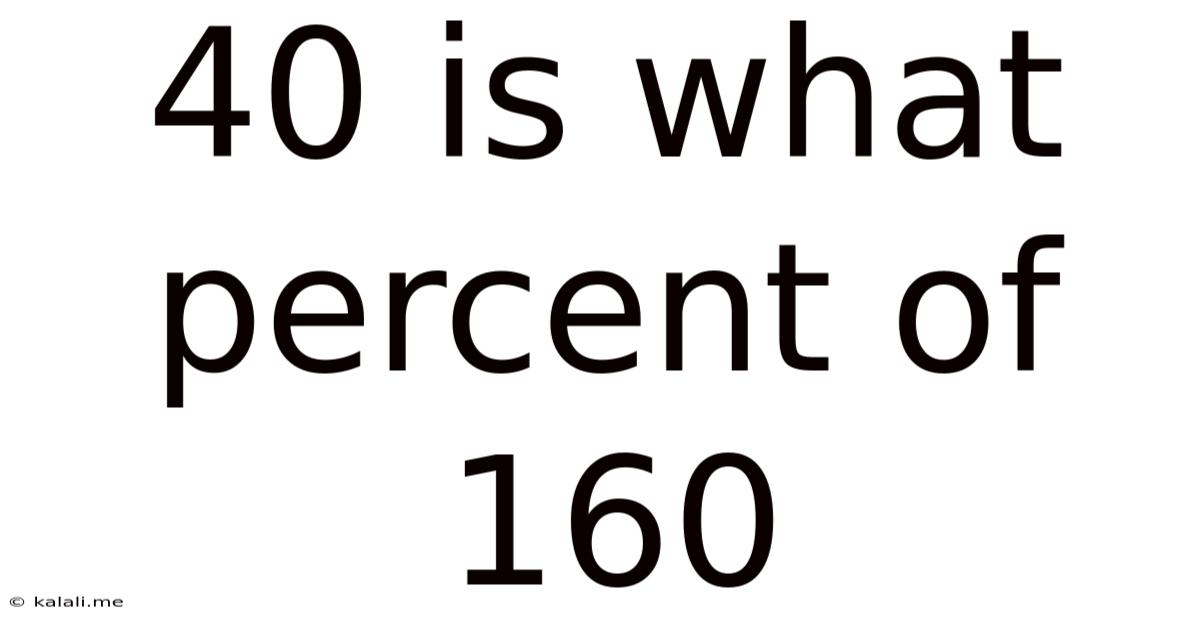
Table of Contents
40 is What Percent of 160? A Comprehensive Guide to Percentage Calculations
The question, "40 is what percent of 160?" might seem simple at first glance. However, understanding how to solve this problem unlocks a crucial skill applicable to countless everyday situations, from calculating discounts and tips to understanding financial reports and statistical data. This comprehensive guide will not only answer the question but will also delve into the underlying principles of percentage calculations, equipping you with the tools to tackle similar problems confidently.
Understanding Percentages
Before diving into the solution, let's establish a solid foundation in percentages. A percentage is simply a fraction expressed as a part of 100. The symbol "%" represents "per cent," meaning "out of one hundred." For example, 25% means 25 out of 100, which can be written as the fraction 25/100 or the decimal 0.25.
Key Concepts:
- Part: This represents the portion we are interested in (in our example, 40).
- Whole: This represents the total amount (in our example, 160).
- Percentage: This is the fraction of the whole expressed as a number out of 100.
Method 1: Using the Percentage Formula
The most straightforward method to solve "40 is what percent of 160?" involves using the basic percentage formula:
(Part / Whole) * 100 = Percentage
Let's substitute our values:
(40 / 160) * 100 = Percentage
Simplifying the fraction:
(1/4) * 100 = 25
Therefore, 40 is 25% of 160.
Method 2: Setting up a Proportion
Another effective approach is setting up a proportion. A proportion is an equation stating that two ratios are equal. We can represent the problem as:
40/160 = x/100
Where 'x' represents the unknown percentage. To solve for 'x,' we can cross-multiply:
40 * 100 = 160 * x
4000 = 160x
Now, divide both sides by 160:
x = 4000 / 160 = 25
Again, we find that 40 is 25% of 160.
Method 3: Using Decimal Equivalents
This method involves converting the fraction to a decimal and then multiplying by 100 to obtain the percentage.
First, divide the part by the whole:
40 / 160 = 0.25
Then, multiply the decimal by 100:
0.25 * 100 = 25%
This confirms that 40 is 25% of 160.
Real-World Applications of Percentage Calculations
The ability to calculate percentages is invaluable in numerous real-world scenarios. Here are a few examples:
-
Calculating Discounts: If a store offers a 20% discount on an item priced at $100, you can easily calculate the discount amount and the final price using percentage calculations. (20/100) * $100 = $20 discount, leaving a final price of $80.
-
Determining Tips: Calculating a 15% tip on a restaurant bill is another common application. For example, a $50 bill would have a tip of ($15/100) * $50 = $7.50.
-
Understanding Financial Statements: Financial reports often utilize percentages to represent ratios, growth rates, and other key metrics. Understanding percentage calculations allows you to interpret these reports effectively.
-
Analyzing Statistical Data: Percentages are extensively used in statistical analysis to represent proportions and probabilities. For instance, understanding the percentage of a population with certain characteristics is crucial in various research fields.
-
Calculating Grades: Many educational systems use percentages to represent student performance on assessments and determine final grades.
Expanding Your Percentage Skills: More Complex Scenarios
While the problem "40 is what percent of 160?" provides a fundamental understanding of percentage calculations, let's explore some more complex scenarios to solidify your grasp of the concept:
Scenario 1: Finding the Whole when given the Part and Percentage
Let's say you know that 30% of a number is 60. How do you find the original number (the whole)?
We can set up the equation:
(30/100) * x = 60
Solving for x:
x = 60 * (100/30) = 200
Therefore, the whole number is 200.
Scenario 2: Finding the Part when given the Whole and Percentage
Suppose you want to find 15% of 300.
Simply multiply the percentage (as a decimal) by the whole:
0.15 * 300 = 45
Therefore, 15% of 300 is 45.
Scenario 3: Percentage Increase/Decrease
Calculating percentage increase or decrease is another crucial skill. Let's say a stock price increases from $50 to $60. What is the percentage increase?
First, find the difference: $60 - $50 = $10
Then, divide the difference by the original value and multiply by 100:
($10 / $50) * 100 = 20%
The stock price increased by 20%.
Mastering Percentage Calculations: Practice and Resources
The key to mastering percentage calculations is consistent practice. The more you work through different types of problems, the more confident and proficient you'll become. Start with simple problems, gradually increasing the complexity. You can find countless practice problems online and in math textbooks.
Additionally, consider using online calculators or educational websites to check your work and learn alternative approaches to solving percentage problems. These resources can provide valuable feedback and help you identify areas where you need additional practice.
Conclusion: The Power of Percentages
Understanding percentage calculations is a fundamental skill with broad applications in various aspects of life. From everyday financial transactions to complex statistical analysis, the ability to accurately compute and interpret percentages is crucial for informed decision-making. By mastering the basic concepts and practicing different problem types, you'll equip yourself with a powerful tool that will greatly enhance your analytical skills and problem-solving abilities. Remember the basic formula, experiment with different methods, and consistently practice to unlock the full power of percentages. You've already taken the first step by exploring this guide – now, put your knowledge to work and see how percentages can help you navigate the world around you!
Latest Posts
Latest Posts
-
75 Cm Equals How Many Inches
Apr 04, 2025
-
Cuanto Es 80 Cm En Pulgadas
Apr 04, 2025
-
How Many Pounds Is 20 Killograms
Apr 04, 2025
-
What Percent Is 24 Of 40
Apr 04, 2025
Related Post
Thank you for visiting our website which covers about 40 Is What Percent Of 160 . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.
