5 Is What Percent Of 9
Kalali
Apr 24, 2025 · 5 min read
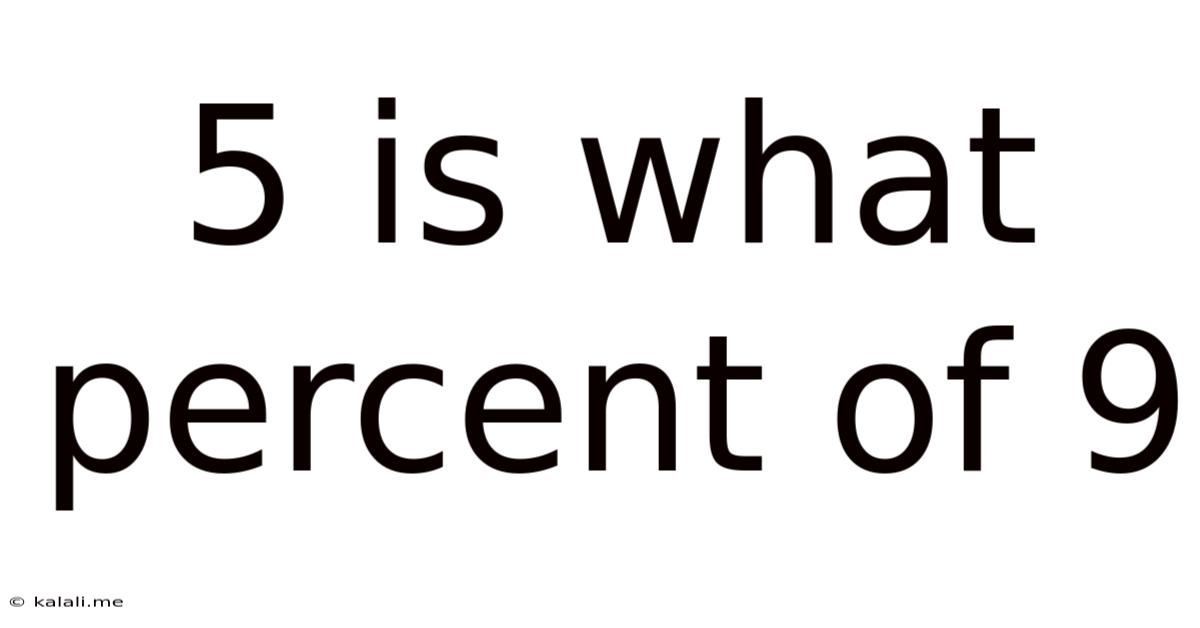
Table of Contents
5 is What Percent of 9? A Comprehensive Guide to Percentage Calculations
Finding out what percentage 5 represents of 9 might seem like a simple math problem, but understanding the underlying concepts and methods is crucial for various applications, from everyday budgeting and shopping to complex financial analyses and scientific calculations. This comprehensive guide will not only answer the question "5 is what percent of 9?" but also equip you with the knowledge to tackle similar percentage problems with ease and confidence. We'll explore multiple approaches, delve into the theory behind percentage calculations, and provide practical examples to solidify your understanding.
Meta Description: Learn how to calculate percentages with ease! This guide answers the question "5 is what percent of 9?" using multiple methods and explores the underlying principles of percentage calculations, providing practical examples for everyday use.
Understanding Percentages
Before diving into the calculation, let's solidify our understanding of percentages. A percentage is simply a fraction expressed as a part of 100. The symbol "%" represents "per cent," which literally translates to "out of one hundred." So, 50% means 50 out of 100, or 50/100, which simplifies to 1/2 or 0.5.
Percentages are a versatile way to represent proportions and ratios. They are widely used to express changes, rates, probabilities, and comparisons. Understanding percentages is essential for interpreting data, making informed decisions, and communicating effectively in various contexts.
Method 1: Using the Formula
The most straightforward method to determine what percentage 5 is of 9 involves using a basic percentage formula:
(Part / Whole) * 100% = Percentage
In this case:
- Part: 5 (the value we want to express as a percentage)
- Whole: 9 (the total value)
Substituting these values into the formula:
(5 / 9) * 100% = 55.555...%
Therefore, 5 is approximately 55.56% of 9. The recurring decimal is usually rounded to two decimal places for practical purposes.
Method 2: Setting up a Proportion
Another effective method involves setting up a proportion. A proportion is an equation stating that two ratios are equal. We can set up a proportion to solve for the unknown percentage (x):
5/9 = x/100
To solve for x, we can cross-multiply:
9x = 500
x = 500/9
x ≈ 55.56
Again, this confirms that 5 is approximately 55.56% of 9.
Method 3: Using Decimal Conversion
This method involves first converting the fraction 5/9 into a decimal and then multiplying by 100% to express it as a percentage.
Dividing 5 by 9 gives us approximately 0.5556.
Multiplying this decimal by 100%:
0.5556 * 100% = 55.56%
This method yields the same result, reinforcing the accuracy of our calculations.
Practical Applications and Real-World Examples
Understanding percentage calculations is vital in many everyday scenarios. Let's consider some practical examples:
-
Sales and Discounts: If a product is discounted by 20%, and its original price is $90, you can easily calculate the discount amount and the final price using percentages. 20% of $90 is (20/100) * $90 = $18. The final price would be $90 - $18 = $72.
-
Grade Calculations: If you scored 5 out of 9 on a quiz, your percentage score would be 55.56%, as we've calculated. This allows for easy comparison of scores across different quizzes with varying total marks.
-
Financial Analysis: Investors and financial analysts constantly use percentages to track returns, growth rates, and other key financial metrics. For example, calculating the percentage increase in profits from one year to the next is essential for understanding business performance.
-
Data Interpretation: Percentages are frequently used to represent data in charts, graphs, and reports. For example, in market research, percentages are used to show market share, customer preferences, and other crucial insights.
-
Cooking and Baking: Recipes often require adjustments based on the number of servings. Understanding percentages helps in scaling up or down recipes proportionally, ensuring consistent results.
Expanding Your Percentage Skills: More Complex Scenarios
While the example of "5 is what percent of 9?" is relatively straightforward, the principles can be applied to more complex scenarios. Consider these examples:
-
Calculating percentage increase or decrease: This involves finding the difference between two values and expressing it as a percentage of the original value. For example, if the price of an item increased from $50 to $60, the percentage increase is [(60-50)/50] * 100% = 20%.
-
Finding the original value given a percentage and a final value: Imagine you bought an item on sale for $72 after a 20% discount. To find the original price, you would solve the equation: 0.8x = 72, where x represents the original price. Solving for x, we get x = $90.
-
Dealing with multiple percentages: Sometimes you need to apply multiple percentage changes consecutively. For example, if an item is increased by 10% and then decreased by 10%, the final value will not be the same as the original value. This requires careful calculation, taking into account the intermediate values.
-
Understanding compound interest: Compound interest calculations rely heavily on percentage calculations. It involves calculating interest not only on the principal but also on accumulated interest from previous periods.
Conclusion: Mastering Percentage Calculations
Mastering percentage calculations is a valuable skill applicable across numerous disciplines and everyday situations. By understanding the underlying principles and practicing different methods, you can confidently tackle a wide range of percentage problems. Remember the fundamental formula, practice with various examples, and explore more complex scenarios to build a strong foundation in percentage calculations. The ability to accurately and efficiently calculate percentages is a key component of numerical literacy and effective problem-solving. From managing your personal finances to interpreting complex datasets, a firm grasp of percentages will significantly enhance your analytical abilities and decision-making skills.
Latest Posts
Latest Posts
-
Cuanto Es 1 78 Metros En Pies
Apr 25, 2025
-
How Many Ml In A Third Of A Cup
Apr 25, 2025
-
What Is Defined Terms In Geometry
Apr 25, 2025
-
What Is 750 Ml In Liters
Apr 25, 2025
-
How To Find No Of Neutrons
Apr 25, 2025
Related Post
Thank you for visiting our website which covers about 5 Is What Percent Of 9 . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.