5 Of 25 Is What Percent
Kalali
Mar 09, 2025 · 5 min read
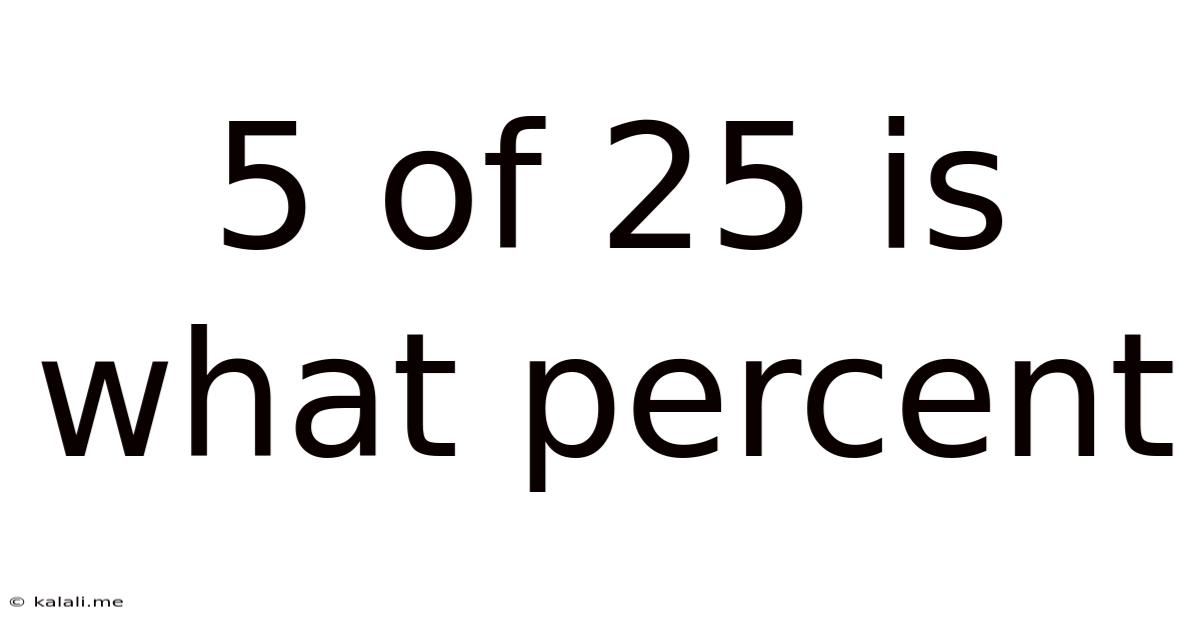
Table of Contents
5 out of 25 is What Percent? A Comprehensive Guide to Percentage Calculations
Understanding percentages is a fundamental skill in various aspects of life, from calculating discounts and taxes to analyzing data and understanding statistics. This comprehensive guide will not only answer the question, "5 out of 25 is what percent?" but also delve into the broader concept of percentage calculations, equipping you with the knowledge and tools to tackle any percentage-related problem.
Understanding Percentages: The Basics
A percentage is simply a fraction expressed as a number out of 100. The word "percent" itself comes from the Latin "per centum," meaning "out of a hundred." Therefore, 50% means 50 out of 100, or 50/100, which simplifies to 1/2 or 0.5.
This foundational understanding is crucial for grasping how to calculate percentages in various scenarios. We often encounter percentages in everyday life:
- Discounts: Stores advertise sales with percentage reductions (e.g., 20% off).
- Taxes: Governments levy taxes expressed as percentages (e.g., sales tax, income tax).
- Interest Rates: Banks and financial institutions use percentages to represent interest rates on loans and savings accounts.
- Data Analysis: Percentages are used extensively in data analysis and statistics to represent proportions and changes.
Calculating Percentages: The Formula
The fundamental formula for calculating percentages is:
(Part / Whole) * 100% = Percentage
Let's break this down:
- Part: This represents the specific amount you're interested in expressing as a percentage of the whole.
- Whole: This represents the total amount.
- Percentage: This is the result expressed as a percentage.
Using this formula, we can answer the core question of this article:
5 out of 25 is What Percent?
In this case:
- Part = 5
- Whole = 25
Applying the formula:
(5 / 25) * 100% = 20%
Therefore, 5 out of 25 is 20%.
Beyond the Basics: More Complex Percentage Calculations
While the basic formula is straightforward, several scenarios require a slightly more nuanced approach. Let's explore some of these:
1. Finding the Part when the Percentage and Whole are Known
Sometimes you know the percentage and the whole and need to find the part. The formula is rearranged as follows:
(Percentage / 100%) * Whole = Part
For example: What is 30% of 150?
(30% / 100%) * 150 = 45
Therefore, 30% of 150 is 45.
2. Finding the Whole when the Percentage and Part are Known
This scenario requires another rearrangement of the formula:
(Part / Percentage) * 100% = Whole
For example: 12 is 25% of what number?
(12 / 25%) * 100% = 48
Therefore, 12 is 25% of 48.
3. Calculating Percentage Increase or Decrease
Percentage increase and decrease are common in various contexts, such as comparing sales figures or tracking changes in population.
- Percentage Increase: [(New Value - Old Value) / Old Value] * 100%
- Percentage Decrease: [(Old Value - New Value) / Old Value] * 100%
For example: If sales increased from 100 to 120, the percentage increase is:
[(120 - 100) / 100] * 100% = 20%
4. Working with Fractions and Decimals
Percentages can be easily converted to fractions and decimals, and vice versa. This can be helpful in simplifying calculations.
- Percentage to Decimal: Divide the percentage by 100. (e.g., 25% = 0.25)
- Decimal to Percentage: Multiply the decimal by 100. (e.g., 0.75 = 75%)
- Percentage to Fraction: Express the percentage as a fraction with a denominator of 100 and simplify. (e.g., 40% = 40/100 = 2/5)
- Fraction to Percentage: Divide the numerator by the denominator and multiply by 100. (e.g., 3/4 = 0.75 * 100% = 75%)
Practical Applications of Percentage Calculations
The ability to calculate percentages has numerous practical applications across diverse fields:
1. Finance and Budgeting
- Calculating interest earned or paid: Understanding interest rates is crucial for managing savings and debt.
- Budgeting and expense tracking: Percentages help in analyzing spending habits and allocating resources efficiently.
- Investment returns: Tracking investment performance involves calculating percentage gains or losses.
2. Retail and Sales
- Calculating discounts and sales tax: This is essential for understanding the final price of goods and services.
- Analyzing sales figures: Percentages are used to track sales trends and assess the success of marketing campaigns.
- Profit margin calculation: Understanding profit margins is vital for businesses to evaluate their profitability.
3. Data Analysis and Statistics
- Representing proportions and distributions: Percentages are used to summarize and visualize data.
- Calculating rates and ratios: Many statistical calculations involve percentages.
- Interpreting survey results: Surveys often present results in percentages to make data easier to understand.
4. Everyday Life
- Tip calculation: Calculating tips in restaurants is a common application of percentages.
- Understanding nutritional information: Food labels often display nutritional information as percentages of recommended daily values.
- Comparing prices and values: Percentages are useful for comparing different deals and offers.
Mastering Percentages: Tips and Tricks
While the underlying concepts are simple, consistent practice is key to mastering percentage calculations. Here are some helpful tips:
- Practice regularly: Solve various percentage problems to build confidence and proficiency.
- Use a calculator: Calculators can significantly speed up calculations, especially for more complex problems.
- Break down complex problems: If a problem seems overwhelming, break it down into smaller, more manageable steps.
- Check your work: Always double-check your answers to avoid errors.
- Utilize online resources: Numerous online calculators and tutorials are available to assist in learning and practicing percentage calculations.
Conclusion: The Power of Percentages
Understanding and calculating percentages is a vital skill with broad applications in personal finance, business, data analysis, and everyday life. By mastering the fundamental concepts and formulas, you can confidently tackle any percentage-related problem and leverage this knowledge to make informed decisions. Remember that consistent practice is the key to building fluency and confidence in this important mathematical skill. The ability to easily determine that 5 out of 25 is 20% is just the beginning of a much wider understanding of how percentages shape our world.
Latest Posts
Latest Posts
-
How Many 32 Oz Make A Gallon
Jul 12, 2025
-
700 Is 10 Times As Much As
Jul 12, 2025
-
How Much Is 120 Kilograms In Pounds
Jul 12, 2025
-
Born In 1990 How Old Am I
Jul 12, 2025
-
What Is The Average Iq For A 5 Year Old
Jul 12, 2025
Related Post
Thank you for visiting our website which covers about 5 Of 25 Is What Percent . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.