5 Of 60 Is What Percent
Kalali
Mar 11, 2025 · 4 min read
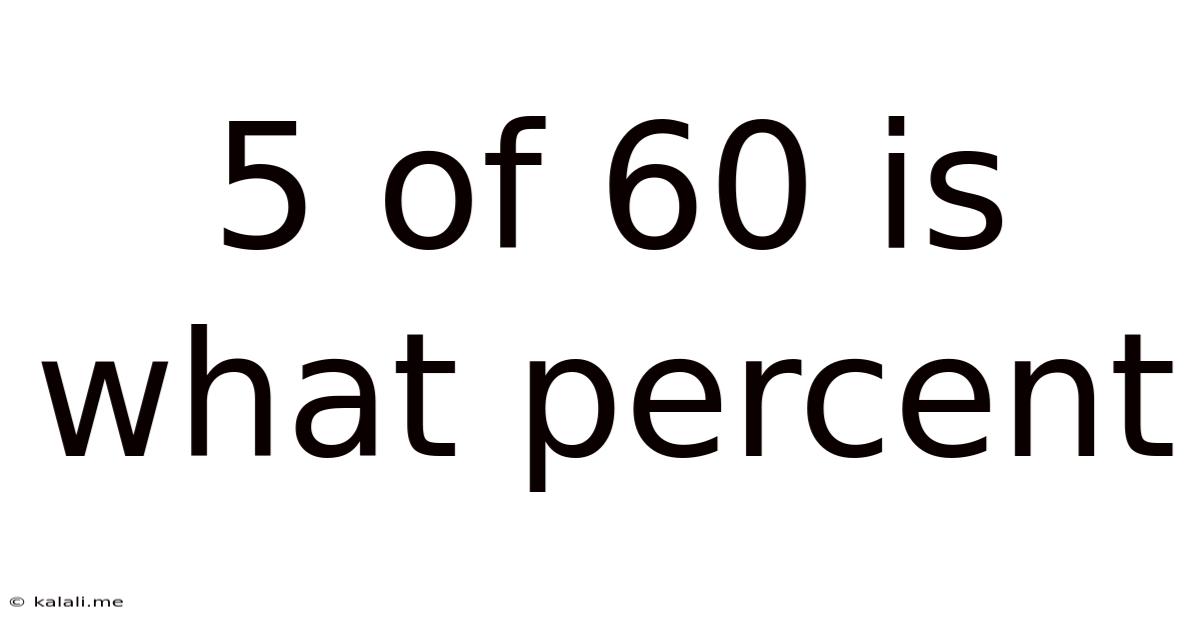
Table of Contents
5 out of 60 is what percent? A Comprehensive Guide to Percentage Calculations
Understanding percentages is a fundamental skill in many aspects of life, from calculating discounts and taxes to analyzing data and understanding statistics. This article will delve into the question, "5 out of 60 is what percent?", providing a step-by-step explanation of how to solve this problem and offering broader insights into percentage calculations. We'll explore different methods, address common pitfalls, and provide practical examples to solidify your understanding.
Understanding the Basics of Percentages
Before diving into the specific calculation, let's establish a firm foundation in understanding percentages. A percentage represents a fraction of 100. The word "percent" itself comes from the Latin "per centum," meaning "out of one hundred." Therefore, any percentage can be expressed as a fraction with a denominator of 100. For example, 25% is equivalent to 25/100, which simplifies to 1/4.
Method 1: Using the Formula
The most direct way to calculate the percentage is using a simple formula:
(Part / Whole) * 100% = Percentage
In our case:
- Part: 5 (the number we are considering as a portion of the whole)
- Whole: 60 (the total number)
Substituting these values into the formula:
(5 / 60) * 100% = Percentage
-
Divide the Part by the Whole: 5 / 60 = 0.08333...
-
Multiply by 100%: 0.08333... * 100% = 8.333...%
Therefore, 5 out of 60 is approximately 8.33%. We usually round percentages to two decimal places for practical purposes.
Method 2: Simplifying the Fraction
Alternatively, we can simplify the fraction before converting it to a percentage. This method can be particularly helpful when dealing with larger numbers.
-
Simplify the Fraction: The fraction 5/60 can be simplified by dividing both the numerator and denominator by their greatest common divisor, which is 5. This simplifies to 1/12.
-
Convert the Fraction to a Decimal: To convert the fraction 1/12 to a decimal, divide the numerator (1) by the denominator (12): 1 / 12 = 0.08333...
-
Multiply by 100%: 0.08333... * 100% = 8.33%
This method yields the same result: 8.33%.
Method 3: Using Proportions
Another approach involves setting up a proportion:
5/60 = x/100
Where 'x' represents the percentage we are trying to find. To solve for 'x':
-
Cross-multiply: 60x = 500
-
Solve for x: x = 500 / 60 = 8.333...
Therefore, x ≈ 8.33%, confirming our previous results.
Practical Applications: Real-World Examples
Understanding percentage calculations is crucial in various real-world scenarios. Let's explore a few examples:
-
Test Scores: If a student answered 5 questions correctly out of a total of 60 questions on a test, their score would be 8.33%.
-
Sales and Discounts: A store offering a discount of 8.33% on an item priced at $60 would reduce the price by $5.
-
Surveys and Statistics: If 5 out of 60 respondents in a survey chose a particular option, that option represents 8.33% of the responses.
-
Financial Calculations: Calculating interest rates, returns on investments, and profit margins often involves percentage calculations.
Common Mistakes to Avoid
Several common mistakes can lead to incorrect percentage calculations. Let's address some of them:
-
Incorrect Order of Operations: Always perform the division before multiplying by 100%.
-
Rounding Errors: Rounding off intermediate results too early can lead to inaccuracies in the final answer. It's best to round only the final result.
-
Confusing Part and Whole: Carefully identify the part and the whole in the problem. The whole is always the total amount or the total number of items.
-
Using Incorrect Formulas: Ensure you are using the correct formula for percentage calculation.
Expanding Your Understanding: Beyond the Basics
While this article focuses on calculating 5 out of 60 as a percentage, the principles discussed apply to a wide range of percentage problems. You can adapt these methods to calculate any percentage, regardless of the numbers involved.
Here are some further considerations:
-
Calculating Percentage Increase or Decrease: These calculations involve finding the percentage change between two values. The formula is: [(New Value - Old Value) / Old Value] * 100%.
-
Finding the Whole When the Percentage and Part are Known: If you know the percentage and the part, you can use the formula: (Part / Percentage) * 100 = Whole.
-
Working with Percentages Greater Than 100%: Percentages can exceed 100% when a value increases beyond its original amount.
-
Using Calculators and Spreadsheets: Calculators and spreadsheet software provide convenient tools for performing percentage calculations quickly and accurately.
Conclusion
Mastering percentage calculations is a valuable skill with wide-ranging applications. This comprehensive guide has explored different methods to determine that 5 out of 60 is approximately 8.33%, providing a solid foundation for understanding and applying percentage calculations in various contexts. Remember to practice regularly and avoid the common pitfalls mentioned to build confidence and accuracy in your calculations. By understanding the core principles and applying the appropriate methods, you can confidently tackle percentage problems and leverage this skill in your personal and professional life.
Latest Posts
Latest Posts
-
How Far Is 600 Miles In Hours
Jul 04, 2025
-
What Do You Get When You Phone A Bee
Jul 04, 2025
-
Was Jennifer Aniston In Sex And The City
Jul 04, 2025
-
Is Dried Oregano A Pure Substance Or Mixture
Jul 04, 2025
-
What Is Fgteev Duddys Real Phone Number
Jul 04, 2025
Related Post
Thank you for visiting our website which covers about 5 Of 60 Is What Percent . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.