5 Out Of 20 As A Percentage
Kalali
Apr 07, 2025 · 5 min read
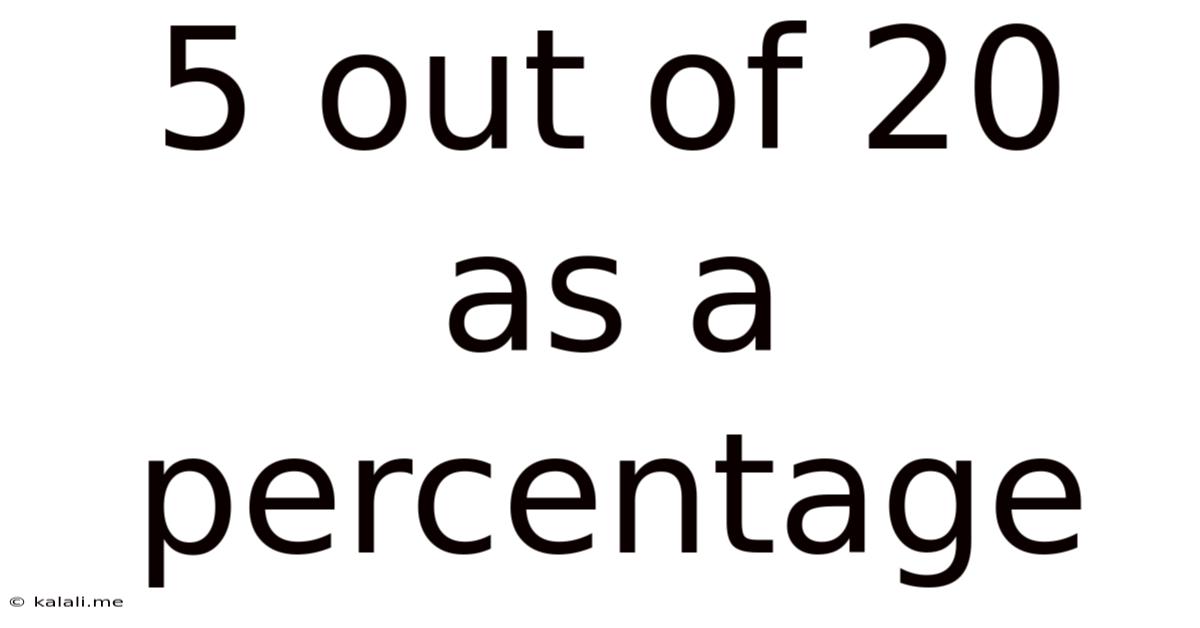
Table of Contents
5 Out of 20 as a Percentage: A Comprehensive Guide
Converting fractions to percentages is a fundamental skill in mathematics with widespread applications in various fields, from everyday budgeting to complex data analysis. Understanding how to express fractions as percentages is crucial for interpreting data, making comparisons, and generally improving numerical literacy. This comprehensive guide will delve into the process of converting 5 out of 20 into a percentage, exploring different methods, providing practical examples, and highlighting the importance of this conversion in real-world scenarios.
Understanding Percentages
Before diving into the specifics of converting 5 out of 20, let's establish a clear understanding of what a percentage represents. A percentage is a fraction or a ratio expressed as a number out of 100. The term "percent" literally means "out of one hundred" (per centum in Latin). Therefore, a percentage represents a proportion of a whole, where the whole is always considered to be 100%. For example, 50% means 50 out of 100, or half.
Method 1: Using the Fraction Method
The most straightforward approach to converting 5 out of 20 into a percentage involves expressing it as a fraction and then converting that fraction to a percentage.
Step 1: Express as a Fraction
The phrase "5 out of 20" directly translates to the fraction 5/20. This fraction represents the ratio of 5 to 20.
Step 2: Simplify the Fraction (Optional but Recommended)
Simplifying the fraction makes the subsequent calculation easier. We can simplify 5/20 by dividing both the numerator (5) and the denominator (20) by their greatest common divisor, which is 5. This simplifies the fraction to 1/4.
Step 3: Convert the Fraction to a Decimal
To convert the simplified fraction 1/4 to a decimal, we divide the numerator (1) by the denominator (4). 1 ÷ 4 = 0.25
Step 4: Convert the Decimal to a Percentage
To convert the decimal 0.25 to a percentage, we multiply it by 100 and add the percent sign (%). 0.25 x 100 = 25%.
Therefore, 5 out of 20 is equal to 25%.
Method 2: Using the Proportion Method
This method involves setting up a proportion to find the equivalent percentage.
Step 1: Set up a Proportion
We can set up a proportion using the given fraction (5/20) and an unknown percentage (x/100):
5/20 = x/100
Step 2: Cross-Multiply
Cross-multiplying the proportion gives us:
20x = 500
Step 3: Solve for x
To solve for x, we divide both sides of the equation by 20:
x = 500 / 20 = 25
Therefore, x = 25, meaning that 5 out of 20 is 25%.
Method 3: Using a Calculator
Modern calculators make percentage conversions extremely simple. Most calculators have a percentage function. You simply enter 5 ÷ 20 and then press the percentage button (%) to obtain the result: 25%. This method is quick and efficient, especially for more complex calculations.
Real-World Applications of Percentage Conversions
The ability to convert fractions to percentages is crucial in numerous real-world situations. Here are a few examples:
1. Academic Performance:
Imagine a student scored 5 out of 20 on a quiz. Converting this score to a percentage (25%) provides a clearer understanding of their performance relative to the total possible points. This allows for easier comparison with other students and assessment of overall progress.
2. Financial Calculations:
Businesses often use percentages to track various financial metrics. For instance, if a company made a profit of $5 on a $20 investment, the return on investment (ROI) would be 25%, providing valuable insights for future investment strategies. Understanding percentages is crucial for budgeting, calculating discounts, and analyzing financial statements.
3. Statistical Analysis:
In statistics, percentages are extensively used to represent data proportions and probabilities. If a survey showed that 5 out of 20 respondents preferred a particular product, this represents 25% preference. This information is crucial for market research and product development.
4. Sales and Discounts:
Retailers frequently use percentages to advertise discounts. A "25% off" sale signifies a reduction of 25% on the original price. Understanding percentages helps consumers calculate the final price and assess the value of discounts.
5. Data Visualization:
Percentages are often used in charts and graphs to visually represent data proportions. Using percentages makes it easier to compare data sets and highlight key trends. For instance, a pie chart showing customer preferences could effectively present the 25% preference for a particular product.
Beyond 5 out of 20: Mastering Percentage Conversions
While this guide focused on converting 5 out of 20 to a percentage, the methods described can be applied to any fraction. The core principle remains the same: express the fraction, simplify if possible, convert to a decimal, and then multiply by 100 to get the percentage. Practice is key to mastering this essential mathematical skill.
Troubleshooting Common Mistakes
Several common mistakes can occur when converting fractions to percentages. Here are some to avoid:
- Incorrect simplification of fractions: Always ensure the fraction is simplified correctly before converting to a decimal.
- Mistakes in decimal-to-percentage conversion: Remember to multiply the decimal by 100, not divide.
- Misunderstanding the concept of percentages: A percentage always represents a proportion out of 100.
Conclusion: The Importance of Percentage Understanding
The ability to convert fractions like 5 out of 20 into percentages is a crucial skill with far-reaching applications. From everyday financial calculations to complex statistical analyses, understanding percentages enhances numerical literacy and aids in interpreting data effectively. Mastering this skill empowers individuals to make informed decisions in various aspects of life, both personal and professional. By understanding the different methods and avoiding common pitfalls, you can confidently navigate the world of percentages and utilize them effectively in your daily life.
Latest Posts
Latest Posts
-
Determine The Empirical Formula From The Molecular Formula C6h12o6
Apr 09, 2025
-
350 Ml Equals How Many Cups
Apr 09, 2025
-
Percentage Of 8 Out Of 12
Apr 09, 2025
-
How Many Liters In 8 Gallons
Apr 09, 2025
-
Whats A 13 Out Of 15
Apr 09, 2025
Related Post
Thank you for visiting our website which covers about 5 Out Of 20 As A Percentage . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.