5 To The Power Of 1
Kalali
Apr 02, 2025 · 6 min read
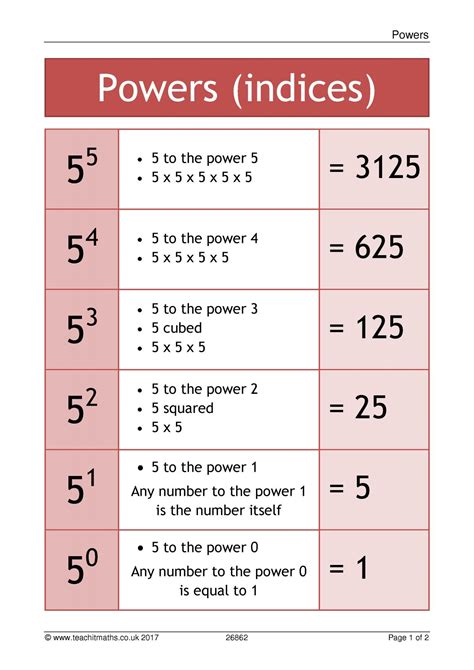
Table of Contents
5 to the Power of 1: A Deep Dive into the Fundamentals of Exponentiation
The seemingly simple expression "5 to the power of 1" (or 5¹), while appearing trivial at first glance, offers a rich opportunity to explore fundamental concepts in mathematics, particularly exponentiation. This seemingly basic calculation serves as a gateway to understanding more complex mathematical ideas, and provides a solid foundation for grasping advanced topics. This article delves deep into the meaning of this expression, its implications, and its connection to broader mathematical principles.
Understanding Exponentiation: The Power of Repeated Multiplication
Exponentiation, at its core, represents repeated multiplication. In the general form a<sup>b</sup>, 'a' is called the base, and 'b' is called the exponent or power. The expression signifies that the base 'a' is multiplied by itself 'b' times. For instance, 2³ (2 to the power of 3) means 2 * 2 * 2 = 8.
The Significance of the Exponent '1'
The exponent plays a crucial role in determining the result of the exponentiation. When the exponent is 1, as in 5¹, it signifies that the base is multiplied by itself only once. This leads to a straightforward and seemingly obvious result: 5¹ = 5.
However, this simplicity belies the importance of understanding the underlying principle. The fact that any number raised to the power of 1 equals itself is a fundamental property of exponentiation, crucial for establishing a consistent mathematical framework. It forms the base case for many mathematical proofs and algorithms.
Connecting 5¹ to the Identity Element in Multiplication
In mathematics, an identity element is a value that, when combined with another value using a specific operation, leaves that other value unchanged. For addition, the identity element is 0 (a + 0 = a). For multiplication, the identity element is 1 (a * 1 = a).
The result of 5¹ = 5 directly demonstrates the multiplicative identity property. Raising any number to the power of 1 is equivalent to multiplying that number by the multiplicative identity, which leaves the number unchanged. This connection highlights the inherent relationship between exponentiation and fundamental arithmetic operations.
Exploring the Patterns and Properties of Exponents
Understanding 5¹ allows us to explore broader patterns and properties associated with exponents:
-
The Power of Zero: While 5¹ is straightforward, considering 5⁰ introduces another key concept. Any non-zero number raised to the power of zero equals 1 (a⁰ = 1, where a ≠ 0). This might seem counterintuitive at first, but it's a consistent extension of the pattern. Consider the sequence: 5³, 5², 5¹, 5⁰. Observe the pattern: each term is obtained by dividing the previous term by 5. Following this pattern, 5⁰ = 5¹ / 5 = 1.
-
Negative Exponents: Extending further, negative exponents represent reciprocals. For example, 5⁻¹ = 1/5. This aligns with the pattern established above and ensures the consistency of the exponent rules across the entire number line.
-
Fractional Exponents: Fractional exponents introduce the concept of roots. For instance, 5<sup>1/2</sup> is the square root of 5. This expands the understanding of exponents beyond simple integer values, opening the door to irrational and complex numbers.
The Role of 5¹ in Algebraic Expressions and Equations
The simplicity of 5¹ makes it a seemingly insignificant term in algebraic equations. However, its existence is crucial in maintaining the structure and consistency of such equations. Consider the following scenarios:
-
Simplifying Expressions: In expressions like 3x² + 5¹x + 2, the 5¹x term maintains the linearity of the equation while contributing to the overall evaluation. While seemingly replaceable with just 5x, understanding that it’s indeed 5¹x highlights the underlying structure and the generalized form of polynomial expressions.
-
Solving Equations: In solving equations, the term 5¹ may be present, even though it may be implicitly understood. For instance, the equation x + 5 = 10 could be conceptually represented as x + 5¹ = 10, reinforcing the application of the exponent in a larger mathematical context.
The Application of Exponents in Advanced Mathematics and Real-World Scenarios
The concept explored in the seemingly basic 5¹ lays the groundwork for numerous advanced mathematical concepts:
-
Calculus: Exponents are fundamental to differential and integral calculus, where derivatives and integrals frequently involve exponential functions.
-
Logarithms: Logarithms are the inverse of exponential functions, meaning they are deeply connected to the concepts of exponents and powers.
-
Financial Modeling: Exponential functions are used extensively in compound interest calculations and modeling of growth and decay phenomena in finance and economics.
-
Physics and Engineering: Exponential functions describe radioactive decay, population growth, and other important real-world phenomena. The foundation of understanding these applications starts with the grasp of fundamental exponents such as 5¹.
Beyond the Numbers: The Pedagogical Value of 5¹
The expression 5¹ holds significant pedagogical value in mathematics education:
-
Building Foundational Understanding: It serves as an excellent starting point for teaching students the concept of exponents in a clear and uncomplicated way.
-
Developing Mathematical Intuition: The consistent application of the exponent rule allows students to develop an intuitive understanding of exponential notation and operations.
-
Promoting Conceptual Learning: By connecting 5¹ to the multiplicative identity and exploring patterns in other powers, educators can foster conceptual understanding rather than rote memorization.
-
Bridging to Advanced Concepts: Understanding 5¹ forms a solid base for advancing to more complex concepts such as negative exponents, fractional exponents, and logarithms.
5¹ and its Impact on Computer Science
The apparently simple 5¹ is not without its significance in the realm of computer science. While not explicitly used in complex algorithms, the understanding of the concept helps in comprehending the foundations of computation, especially when dealing with data structures and algorithms.
-
Bit Manipulation: In binary systems, understanding the concepts of powers of 2 (and indirectly, the principles of exponents) is essential for bit manipulation and understanding memory allocation. The underlying principle behind 5¹ strengthens one's grasp of the fundamental relationships in numerical systems.
-
Algorithmic Efficiency: Analyzing the efficiency of algorithms involves understanding how the computation time or space complexity grows with the input size. This often involves exponential functions, highlighting once again the importance of understanding the fundamentals of exponentiation.
Conclusion: The Unsung Importance of 5¹
In conclusion, while the calculation of 5¹ might seem trivial, it plays a significant role in building a strong foundation in mathematics and related fields. Its simplicity hides a profound connection to the multiplicative identity, the broader principles of exponentiation, and the numerous applications of these concepts in diverse areas of study. Understanding this simple expression is vital for navigating the complexities of more advanced mathematical concepts, making it a cornerstone of mathematical literacy. The seemingly insignificant 5¹ serves as a powerful reminder that even the most basic concepts can hold immense value and profound implications within the larger mathematical framework. It is a testament to the elegance and interconnectedness of mathematical principles.
Latest Posts
Latest Posts
-
What Indicates The Amplitude Of A Compressional Wave
Apr 03, 2025
-
How Many Mm Is 11 Cm
Apr 03, 2025
-
1 Meter 55 Cm In Feet
Apr 03, 2025
-
How Many Centimeters Are In 39 Millimeters
Apr 03, 2025
-
4 Over 3 As A Decimal
Apr 03, 2025
Related Post
Thank you for visiting our website which covers about 5 To The Power Of 1 . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.
