7 To The Power Of 0
Kalali
Mar 29, 2025 · 5 min read
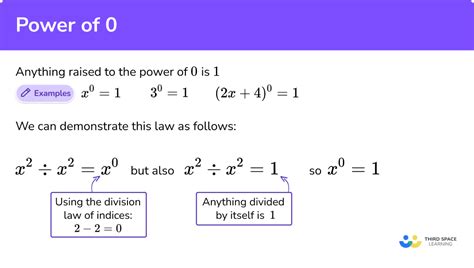
Table of Contents
7 to the Power of 0: Unveiling the Mystery Behind Zero Exponents
The seemingly simple mathematical expression, 7⁰, often sparks confusion and debate, particularly among those less familiar with the intricacies of exponential notation. While the answer itself is straightforward – 7⁰ = 1 – understanding why this is true requires delving into the fundamental principles governing exponents and their behavior. This comprehensive guide will explore this seemingly simple yet profoundly important concept, examining its implications across various mathematical fields and providing a solid foundation for further exploration.
Understanding Exponents: A Quick Refresher
Before diving into the specifics of zero exponents, let's review the basic principles of exponential notation. An exponent, also known as a power or index, indicates how many times a base number is multiplied by itself. For instance:
- 7² = 7 x 7 = 49 (7 raised to the power of 2, or 7 squared)
- 7³ = 7 x 7 x 7 = 343 (7 raised to the power of 3, or 7 cubed)
- 7⁴ = 7 x 7 x 7 x 7 = 2401 (7 raised to the power of 4)
Notice a pattern? As the exponent increases by one, we multiply the previous result by the base number (7). This consistent pattern is crucial to understanding why 7⁰ equals 1.
The Logic Behind 7⁰ = 1: A Multifaceted Approach
There are several ways to approach the explanation of 7⁰ = 1, each reinforcing the validity of the result. These different perspectives provide a deeper understanding of the underlying mathematical principles.
1. The Pattern Approach: Maintaining Consistency
Let's examine the pattern created by decreasing exponents:
- 7⁴ = 2401
- 7³ = 343 (2401 / 7)
- 7² = 49 (343 / 7)
- 7¹ = 7 (49 / 7)
Observe that each time the exponent decreases by one, we divide the previous result by the base number (7). Following this established pattern, the next step would be:
- 7⁰ = 7 / 7 = 1
This consistent pattern underscores the logical conclusion that 7⁰ must equal 1 to maintain the established sequence. The pattern dictates the rule; the rule doesn't dictate the pattern. This consistency is crucial in mathematical operations, maintaining predictability and reliability.
2. The Identity Element Approach: Preserving Multiplicative Identity
In mathematics, the identity element is a number that, when combined with another number using a specific operation, leaves that number unchanged. For multiplication, the identity element is 1. This means that any number multiplied by 1 remains the same.
Consider the following:
- 7¹ = 7
- 7¹ = 7⁰ * 7¹ (This is true because multiplying by 7⁰ doesn't change the value.)
For this equation to hold true, 7⁰ must be equal to 1. Otherwise, the equation would be unbalanced. This approach highlights the importance of maintaining the multiplicative identity in exponential operations.
3. The Empty Product Approach: The Product of No Numbers
Another perspective involves the concept of an empty product. An exponent essentially represents repeated multiplication. When the exponent is zero, it implies there are zero instances of multiplication. The product of no numbers, by convention, is defined as 1. This is analogous to the factorial of zero (0!), which is also defined as 1. This approach emphasizes the foundational definition of exponents and their relationship to multiplication.
Extending the Concept: Beyond 7⁰
The principle governing 7⁰ extends to all non-zero base numbers. In general, for any non-zero number 'a', a⁰ = 1. The exception is 0⁰, which is undefined; this will be discussed further below.
The rule that x⁰ = 1 (where x ≠ 0) is a fundamental concept that permeates numerous mathematical areas, influencing algebraic manipulations, calculus, and more. Understanding this concept thoroughly is vital for anyone pursuing a deeper understanding of mathematics.
Applications in Various Mathematical Fields
The rule a⁰ = 1 isn’t just a theoretical curiosity; it has practical implications across various branches of mathematics:
-
Algebra: Simplifying algebraic expressions often involves manipulating exponents, and the rule a⁰ = 1 is essential for correctly simplifying expressions. For example, in simplifying (x³y²z⁰)/x², we know that z⁰ = 1, simplifying the expression.
-
Calculus: Derivatives and integrals frequently involve exponential functions. Understanding how exponents behave, including the case of zero exponents, is critical for accurate calculations.
-
Probability and Statistics: Many statistical calculations rely heavily on exponential functions, and understanding the behavior of zero exponents becomes crucial for correct calculations, especially in working with probability distributions.
-
Computer Science: Exponents play a significant role in computational algorithms and data structures, impacting efficiency and scalability. Understanding zero exponents is fundamental to the correct functioning of many algorithms and data structures.
The Exception: Why 0⁰ Is Undefined
While a⁰ = 1 holds for all non-zero values of 'a', the case of 0⁰ is an exception and is considered undefined. This seemingly simple expression hides a more complex issue stemming from conflicting mathematical conventions.
Consider the following two limiting approaches:
-
Approach 1: Holding the base constant and letting the exponent approach zero: lim (x→0) x⁰ = 1 (for any x≠0). The limit approaches 1 consistently.
-
Approach 2: Holding the exponent constant and letting the base approach zero: lim (x→0) 0ˣ = 0 (for any x>0). The limit approaches 0.
These conflicting approaches highlight the reason for the undefined nature of 0⁰. Depending on the direction of approach, the result differs, making it impossible to assign a single, consistent value.
Conclusion: A Foundation of Mathematical Understanding
The seemingly simple expression 7⁰ = 1 is a cornerstone of exponential notation and a gateway to deeper mathematical understanding. By understanding the different perspectives – the pattern approach, the multiplicative identity approach, and the empty product approach – we can build a robust intuition about why this equation holds true. While 0⁰ remains an exception due to its inherent ambiguity, the overarching rule for non-zero bases provides a solid foundation for further exploration of exponents and their profound impact across various mathematical disciplines. Mastering this seemingly simple concept opens doors to a vast world of mathematical exploration and problem-solving. It truly is a cornerstone upon which much more complex mathematical ideas are built. From simple algebraic manipulation to sophisticated calculus problems, understanding 7⁰ = 1 (and the broader concept of zero exponents) is an essential step toward mathematical proficiency.
Latest Posts
Latest Posts
-
101 Do F Bang Bao Nhieu Do C
Mar 31, 2025
-
List The First 5 Multiples Of 1
Mar 31, 2025
-
Matter That Has A Uniform And Definite Composition
Mar 31, 2025
-
2 Percent Of 2 Fluid Ounces
Mar 31, 2025
-
Both Parents Have Brown Eyes Baby Has Blue
Mar 31, 2025
Related Post
Thank you for visiting our website which covers about 7 To The Power Of 0 . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.
