80 Is What Percent Of 250
Kalali
Apr 17, 2025 · 5 min read
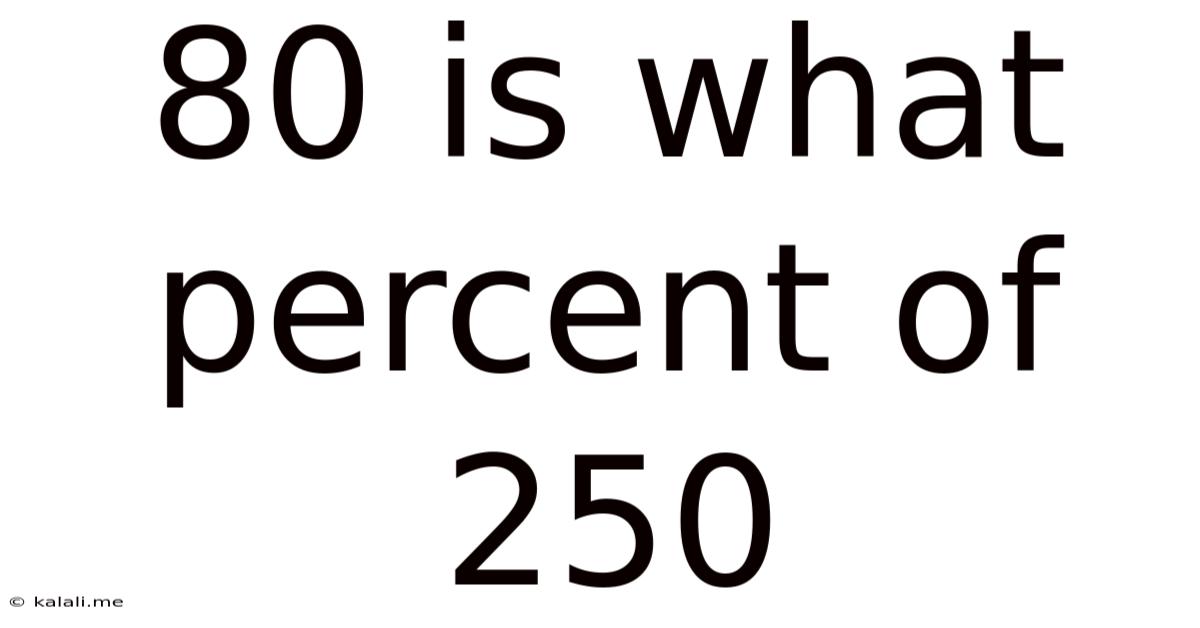
Table of Contents
80 is What Percent of 250? A Comprehensive Guide to Percentage Calculations
This seemingly simple question – "80 is what percent of 250?" – opens the door to a world of practical applications in various fields, from everyday budgeting and shopping to complex statistical analysis and scientific research. Understanding how to calculate percentages is a fundamental skill, and this article will delve deep into the process, providing multiple methods, practical examples, and advanced concepts to solidify your understanding.
Meta Description: Learn how to calculate percentages effectively! This comprehensive guide explains multiple methods to solve "80 is what percent of 250?", covering fundamental concepts and advanced applications. Master percentage calculations and enhance your problem-solving skills.
Understanding Percentages: The Basics
A percentage is a fraction or ratio expressed as a number out of 100. The term "percent" is derived from the Latin "per centum," meaning "out of one hundred." Therefore, 50% means 50 out of 100, or 50/100, which simplifies to 1/2 or 0.5. Percentages are a convenient way to express proportions and make comparisons.
Method 1: The Formula Approach
The most straightforward way to find what percent 80 is of 250 is using the basic percentage formula:
(Part / Whole) * 100 = Percentage
In this case:
- Part: 80 (the number we're considering as a portion of the whole)
- Whole: 250 (the total amount)
Substituting the values into the formula:
(80 / 250) * 100 = 32%
Therefore, 80 is 32% of 250.
Method 2: Using Proportions
Another effective method involves setting up a proportion:
80 / 250 = x / 100
Where 'x' represents the unknown percentage. To solve for x, we cross-multiply:
80 * 100 = 250 * x
8000 = 250x
x = 8000 / 250
x = 32
Therefore, x = 32%, confirming our previous result. This method highlights the relationship between fractions and percentages.
Method 3: Decimal Conversion
This approach involves converting the fraction into a decimal and then multiplying by 100 to obtain the percentage:
80 / 250 = 0.32
0.32 * 100 = 32%
This method is particularly useful when dealing with more complex fractions or when using a calculator. The decimal representation provides an intermediate step for easier understanding.
Practical Applications: Real-World Examples
Percentage calculations are ubiquitous in our daily lives. Here are a few examples demonstrating their practical applications:
-
Sales and Discounts: A store offers a 20% discount on an item priced at $150. The discount amount is (20/100) * $150 = $30, and the final price is $150 - $30 = $120. Similarly, if a shirt is on sale for $25, and its original price was $50, it's a 50% discount ((50-25)/50 * 100 = 50%).
-
Tax Calculations: A 6% sales tax on a $500 purchase is (6/100) * $500 = $30. The total cost including tax is $500 + $30 = $530. Understanding tax percentages is crucial for budgeting and financial planning.
-
Grade Calculations: If you scored 85 out of 100 on a test, your percentage score is (85/100) * 100 = 85%. This helps determine your overall performance and grade point average.
-
Interest Rates: Calculating interest on loans or investments frequently involves percentages. For example, a 5% annual interest rate on a $1000 investment will yield $50 in interest after one year. Understanding compound interest, which involves calculating interest on both the principal and accumulated interest, requires a solid grasp of percentage calculations.
-
Data Analysis: Percentages are indispensable in statistical analysis. They help represent proportions within datasets, allowing for comparisons and drawing conclusions. For example, understanding market share often involves analyzing percentages of total sales held by different companies.
Advanced Concepts and Considerations
While the basic percentage calculation is straightforward, understanding certain nuances can enhance your proficiency:
-
Percentage Increase and Decrease: Calculating percentage changes involves finding the difference between two values and expressing it as a percentage of the original value. For instance, an increase from 100 to 120 is a 20% increase ((120-100)/100 * 100 = 20%), while a decrease from 150 to 120 represents a 20% decrease ((150-120)/150 * 100 = 20%). Note that the base for percentage change is the original value.
-
Compounding Percentages: When percentages are applied sequentially, the resulting value is not simply the sum of the individual percentages. This is especially relevant in scenarios like compound interest where interest earned in one period is added to the principal, and interest is calculated on the increased amount in the next period. Understanding this compounding effect is crucial for long-term financial planning.
-
Percentage Points vs. Percentage Change: It’s important to distinguish between percentage points and percentage change. A change from 10% to 15% is a 5 percentage point increase, but a 50% increase relative to the initial 10%.
-
Working with Percentages Greater than 100%: Percentages can exceed 100%, indicating a value more than the initial amount. This is common in contexts like growth rates, where a value may more than double. For instance, a 150% increase means the final value is 2.5 times the initial value (1 + 1.5 = 2.5).
Troubleshooting and Common Mistakes
Several common mistakes can arise when calculating percentages:
-
Incorrect Formula Application: Ensure you use the correct formula – (Part / Whole) * 100 – and substitute the values accurately.
-
Misinterpreting the Question: Carefully read the question to identify the "part" and the "whole" correctly.
-
Calculation Errors: Double-check your calculations to avoid arithmetic mistakes. Using a calculator can help minimize errors.
-
Confusing Percentage Points and Percentage Change: Always clarify whether the problem requires a percentage point difference or a percentage change.
Conclusion: Mastering Percentage Calculations
Understanding percentage calculations is a vital skill across numerous disciplines. From personal finance to scientific research, the ability to accurately calculate and interpret percentages empowers informed decision-making. By grasping the fundamental concepts, exploring different methods, and understanding potential pitfalls, you can confidently tackle any percentage-related problem. Remember to practice regularly and apply your knowledge in real-world scenarios to reinforce your understanding and enhance your problem-solving capabilities. The seemingly simple question, "80 is what percent of 250?", thus serves as a gateway to a wider world of mathematical applications and practical skills.
Latest Posts
Latest Posts
-
14 3 As A Mixed Number
Apr 19, 2025
-
How Many Cups Of Water Is 24 Ounces
Apr 19, 2025
-
What Is 2 3 8 As A Decimal
Apr 19, 2025
-
How To Tell A Chemical Reaction Has Occurred
Apr 19, 2025
-
How Much Is 23 Degrees Celsius In Fahrenheit
Apr 19, 2025
Related Post
Thank you for visiting our website which covers about 80 Is What Percent Of 250 . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.