9 Is What Percent Of 20
Kalali
Apr 08, 2025 · 5 min read
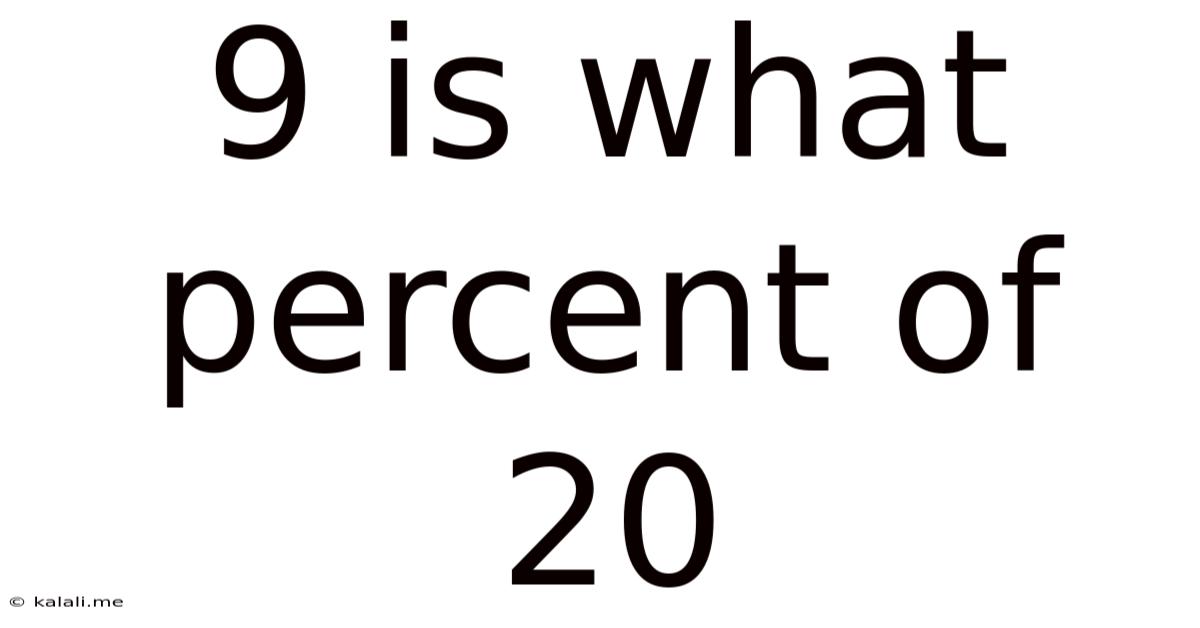
Table of Contents
9 is What Percent of 20? A Deep Dive into Percentage Calculations and Their Applications
This seemingly simple question, "9 is what percent of 20?", opens the door to a surprisingly wide world of mathematical concepts and practical applications. While the answer itself is straightforward, understanding the underlying principles and exploring various methods of solving it provides a valuable foundation for tackling more complex percentage problems. This article will delve into the calculation, explore different approaches, and demonstrate the relevance of percentage calculations in everyday life and various professional fields.
Meta Description: Discover multiple ways to calculate what percentage 9 represents of 20. This in-depth guide explores the fundamental principles of percentage calculations and their real-world applications, from everyday finances to complex scientific analyses.
Understanding Percentages: The Foundation
Before jumping into the calculation, let's solidify our understanding of percentages. A percentage is simply a fraction expressed as a number out of 100. The symbol "%" represents "per hundred" or "out of 100". Therefore, 50% means 50 out of 100, or 50/100, which simplifies to 1/2.
Method 1: The Basic Formula
The most fundamental way to calculate percentages is using the formula:
(Part / Whole) * 100% = Percentage
In our case:
- Part: 9
- Whole: 20
Substituting these values into the formula:
(9 / 20) * 100% = 45%
Therefore, 9 is 45% of 20.
Method 2: Using Proportions
Another effective method involves setting up a proportion:
9/20 = x/100
Where 'x' represents the percentage we're trying to find. To solve for 'x', we cross-multiply:
20x = 900
x = 900 / 20
x = 45
Therefore, 9 is 45% of 20. This method highlights the relationship between fractions and percentages.
Method 3: Decimal Conversion
We can also convert the fraction to a decimal and then multiply by 100%:
9 / 20 = 0.45
0.45 * 100% = 45%
This method is particularly useful when dealing with calculations that involve decimals or when using calculators that don't directly support percentage calculations.
Beyond the Basic Calculation: Real-World Applications
While the calculation itself is straightforward, the concept of percentages finds applications across a vast array of fields:
1. Finance and Personal Budgeting:
-
Interest Rates: Understanding percentage calculations is crucial for comprehending interest rates on loans, mortgages, and savings accounts. Calculating the interest accrued on investments or the total cost of a loan with interest requires proficiency in percentage calculations. For instance, understanding that a 5% interest rate on a $10,000 loan means you'll pay $500 in interest in the first year is vital for financial planning.
-
Discounts and Sales: Sales and discounts are frequently expressed as percentages. Calculating the final price of an item after a 20% discount requires understanding how to subtract a percentage from the original price. This is a common application used daily by consumers.
-
Taxes: Sales tax, income tax, and property tax are all calculated as percentages. Knowing how to calculate these taxes is crucial for managing personal finances effectively. Calculating your tax liability or the tax on a purchase involves utilizing percentage calculations.
-
Investment Returns: Tracking investment performance often involves calculating percentage changes in the value of investments over time. Knowing the percentage increase or decrease in your investment portfolio allows you to make informed decisions about your financial future. This requires comparing the difference between the initial investment and final value as a percentage of the original investment.
2. Science and Data Analysis:
-
Statistical Analysis: Percentages are fundamental to statistical analysis. Expressing data as percentages allows for easier comparison and interpretation of results. For example, expressing survey results as percentages helps to understand the proportion of respondents who chose a specific option.
-
Scientific Experiments: Many scientific experiments involve calculating percentages to represent the results. For example, calculating the percentage of successful trials in an experiment or the percentage of a specific element in a compound.
-
Data Visualization: Charts and graphs often use percentages to represent data proportions effectively and understandably. Percentages provide a standardized way to compare different data sets and visually communicate findings. Pie charts and bar graphs often utilize percentages to illustrate proportions.
3. Business and Marketing:
-
Market Share: Companies use percentage calculations to determine their market share relative to their competitors. Knowing your percentage of the market allows you to set strategic goals.
-
Profit Margins: Calculating profit margins as a percentage of revenue is essential for business performance analysis. Profit margins indicate the profitability of your business model.
-
Sales Growth: Tracking sales growth year over year as a percentage helps assess the success of marketing campaigns and overall business strategies.
-
Conversion Rates: In online marketing, conversion rates (e.g., the percentage of website visitors who make a purchase) are crucial metrics for evaluating campaign effectiveness. Analyzing conversion rates is critical for optimizing marketing strategies and improving ROI.
4. Everyday Life:
-
Tip Calculation: Calculating tips in restaurants involves calculating a percentage of the total bill.
-
Recipe Scaling: Adjusting recipe ingredients to serve more or fewer people often requires understanding and applying percentage changes.
-
Comparison Shopping: Comparing the prices of different items while considering discounts or sales often involves using percentages to determine the best deal.
Expanding on Percentage Calculations: More Complex Scenarios
While the example of "9 is what percent of 20?" is relatively simple, the principles extend to more complex scenarios:
-
Calculating Percentage Increase or Decrease: This involves finding the difference between two numbers and expressing it as a percentage of the original number. For instance, if a price increases from $10 to $12, the percentage increase is ((12-10)/10) * 100% = 20%.
-
Finding the Original Value: If you know a percentage and the resulting value, you can work backward to find the original value. For example, if a discounted price of $80 represents 80% of the original price, the original price was $80 / 0.80 = $100.
-
Compound Percentages: These calculations involve applying a percentage multiple times, such as calculating compound interest.
Conclusion: Mastering Percentages for a Brighter Future
Understanding percentage calculations is not just about solving mathematical problems; it's about developing a crucial life skill. From managing your personal finances to analyzing complex data, proficiency in percentage calculations empowers you to make informed decisions, interpret information critically, and excel in various aspects of life and professional endeavors. The seemingly simple question, "9 is what percent of 20?", serves as a gateway to a broader understanding of a fundamental concept with far-reaching implications. Mastering these calculations will undoubtedly benefit you in numerous situations, both personally and professionally.
Latest Posts
Latest Posts
-
How Many Calories Does 1 G Of Uranium Have
Apr 17, 2025
-
Cuanto Es 20 Pulgadas En Metros
Apr 17, 2025
-
What Is 40 Percent Of 10
Apr 17, 2025
-
150 Ml Is How Many Cups
Apr 17, 2025
-
What Is 2 5 8 As A Decimal
Apr 17, 2025
Related Post
Thank you for visiting our website which covers about 9 Is What Percent Of 20 . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.