A Limit Involving The Cosine Functio
Kalali
Apr 02, 2025 · 6 min read
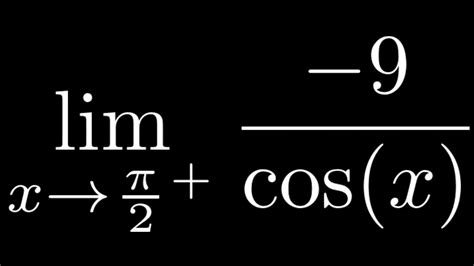
Table of Contents
A Limit Involving the Cosine Function: Exploring the Beauty of Mathematical Limits
The world of mathematics is brimming with fascinating concepts, and among them, limits hold a special place. Limits describe the behavior of a function as its input approaches a particular value. Understanding limits is crucial for comprehending calculus and its numerous applications in science, engineering, and beyond. This article delves deep into a specific limit involving the cosine function, exploring its nuances and providing a detailed explanation of its derivation and significance. We'll uncover the beauty hidden within this seemingly simple mathematical expression.
Understanding Limits: A Foundation
Before we embark on our journey into the specific limit involving the cosine function, let's refresh our understanding of limits. A limit of a function f(x) as x approaches a (denoted as lim<sub>x→a</sub> f(x)) describes the value that f(x) approaches as x gets arbitrarily close to a, but not necessarily equal to a. It's crucial to note that the function f(x) doesn't need to be defined at x = a for the limit to exist.
Key Concepts:
-
Intuitive Understanding: Imagine walking along a path represented by the graph of a function. As you approach a specific point on the path, the limit represents the altitude (y-value) you're approaching. You might never actually reach that exact point, but you can get arbitrarily close to it.
-
Formal Definition (ε-δ Definition): This provides a rigorous mathematical definition of a limit. Essentially, it states that for any small positive number ε (epsilon), there exists a positive number δ (delta) such that if 0 < |x - a| < δ, then |f(x) - L| < ε, where L is the limit. This means that we can make f(x) arbitrarily close to L by choosing x sufficiently close to a.
-
One-Sided Limits: Sometimes, the behavior of a function as it approaches a point from the left (lim<sub>x→a<sup>-</sup></sub> f(x)) differs from its behavior as it approaches from the right (lim<sub>x→a<sup>+</sup></sub> f(x)). If these one-sided limits are equal, then the overall limit exists.
The Limit in Focus: lim<sub>x→0</sub> (cos(x) - 1) / x
Now, let's focus on the limit we'll be exploring in detail:
lim<sub>x→0</sub> (cos(x) - 1) / x
This limit is a classic example that often arises in calculus and its applications. It may appear simple at first glance, but it requires a careful approach to evaluate correctly. Simply substituting x = 0 directly results in an indeterminate form (0/0), which is not helpful. Therefore, we need to employ more sophisticated techniques.
Method 1: Using L'Hôpital's Rule
L'Hôpital's Rule is a powerful tool for evaluating limits of indeterminate forms. It states that if the limit of the ratio of two functions f(x) and g(x) as x approaches a results in an indeterminate form (0/0 or ∞/∞), then the limit is equal to the limit of the ratio of their derivatives:
lim<sub>x→a</sub> f(x) / g(x) = lim<sub>x→a</sub> f'(x) / g'(x) (provided the limit on the right exists).
Applying L'Hôpital's Rule to our limit:
- f(x) = cos(x) - 1
- g(x) = x
- f'(x) = -sin(x)
- g'(x) = 1
Therefore:
lim<sub>x→0</sub> (cos(x) - 1) / x = lim<sub>x→0</sub> (-sin(x)) / 1 = -sin(0) = 0
Thus, using L'Hôpital's Rule, we elegantly determine that the limit is 0.
Method 2: Using Taylor Series Expansion
Another powerful method to evaluate this limit involves the Taylor series expansion of the cosine function around x = 0. The Taylor series expansion of cos(x) is given by:
cos(x) = 1 - x²/2! + x⁴/4! - x⁶/6! + ...
Substituting this into our limit:
lim<sub>x→0</sub> (cos(x) - 1) / x = lim<sub>x→0</sub> [(1 - x²/2! + x⁴/4! - x⁶/6! + ...) - 1] / x
Simplifying:
lim<sub>x→0</sub> (-x²/2! + x⁴/4! - x⁶/6! + ...) / x = lim<sub>x→0</sub> (-x/2! + x³/4! - x⁵/6! + ...)
Now, as x approaches 0, all terms containing x will approach 0. Therefore:
lim<sub>x→0</sub> (-x/2! + x³/4! - x⁵/6! + ...) = 0
Again, we arrive at the same conclusion: the limit is 0.
Significance and Applications
The limit lim<sub>x→0</sub> (cos(x) - 1) / x = 0 holds significant importance in various areas of mathematics and its applications:
-
Calculus: This limit is fundamental in deriving derivatives and integrals involving trigonometric functions. It showcases the interplay between limits, derivatives, and trigonometric identities.
-
Differential Equations: Many differential equations involving trigonometric functions rely on this limit for their solutions.
-
Physics and Engineering: Applications in areas like wave propagation, oscillations, and signal processing frequently encounter limits involving trigonometric functions, where this specific limit plays a crucial role in simplifying complex expressions.
-
Numerical Analysis: This limit is often used in developing numerical methods for approximating the solutions of differential equations and other mathematical problems. Understanding this limit helps us evaluate the accuracy and stability of these methods.
-
Probability and Statistics: While less direct, the underlying principles of limits and approximations related to this limit can be used in certain probabilistic scenarios involving continuous random variables and their distributions.
Exploring Related Limits
Understanding this particular limit opens the door to exploring related limits involving trigonometric functions. For example, we can consider limits involving other trigonometric functions like sine or tangent. The techniques used to evaluate lim<sub>x→0</sub> (cos(x) - 1) / x, such as L'Hôpital's Rule and Taylor series expansion, can be readily adapted to evaluate similar limits.
For instance, the limit lim<sub>x→0</sub> sin(x)/x is another classic limit that equals 1. This limit is also crucial in many mathematical and scientific applications. By understanding the methods applied to the cosine limit, one can approach this sine limit with similar techniques and gain a deeper appreciation for the interconnectedness of these mathematical concepts.
Furthermore, exploring limits involving combinations of trigonometric functions can lead to further insights and understanding. The application of techniques like L'Hopital's rule and Taylor series expansions remain invaluable tools in navigating the intricacies of these mathematical expressions.
Conclusion: The Elegance of Limits
This article has explored in detail a specific limit involving the cosine function: lim<sub>x→0</sub> (cos(x) - 1) / x. We've demonstrated two different methods for evaluating this limit—L'Hôpital's Rule and Taylor series expansion—highlighting the versatility and power of these mathematical tools. We've also touched upon the significance and applications of this limit, emphasizing its fundamental role in calculus, differential equations, physics, engineering, and other related fields. Understanding this limit not only deepens our understanding of calculus but also underscores the elegance and power of mathematical limits in solving complex problems across various disciplines. The exploration of this limit serves as a springboard for understanding more complex limits and reinforces the fundamental importance of limits in higher-level mathematics and its applications. It's a testament to the beauty and utility hidden within seemingly simple mathematical expressions.
Latest Posts
Latest Posts
-
Which Word Best Describes The Tone Of The Passage
Jul 06, 2025
-
How Long Does It Take To Count To One Billion
Jul 06, 2025
-
Why Did The Kangaroo See A Psychiatrist
Jul 06, 2025
-
How Long Would It Take To Walk To China
Jul 06, 2025
-
Step Up To The Streets Final Dance
Jul 06, 2025
Related Post
Thank you for visiting our website which covers about A Limit Involving The Cosine Functio . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.