A Ratio That Compares A Number To 100
Kalali
Mar 27, 2025 · 6 min read
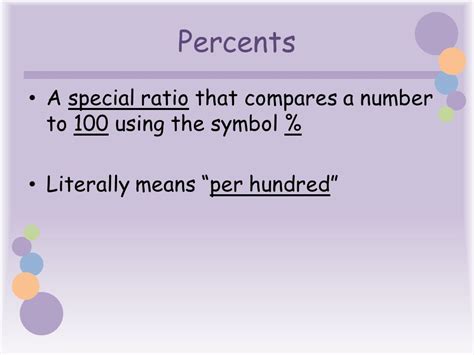
Table of Contents
- A Ratio That Compares A Number To 100
- Table of Contents
- Understanding Percentages: The Ratio That Compares a Number to 100
- What is a Percentage?
- Calculating Percentages: Different Methods
- Method 1: Using the Fraction Method
- Method 2: Using Decimal Multiplication
- Method 3: Using Proportions
- Applications of Percentages in Real Life
- 1. Finance and Business:
- 2. Statistics and Data Analysis:
- 3. Science and Engineering:
- 4. Everyday Life:
- Understanding Percentage Increase and Decrease
- Percentage Increase:
- Percentage Decrease:
- Common Percentage Calculations and Their Applications
- Advanced Applications and Considerations
- Avoiding Common Mistakes with Percentages
- Conclusion
- Latest Posts
- Latest Posts
- Related Post
Understanding Percentages: The Ratio That Compares a Number to 100
Percentages are a fundamental concept in mathematics and everyday life. They represent a ratio that compares a number to 100, providing a standardized way to express proportions and fractions. Understanding percentages is crucial for various applications, from calculating discounts and taxes to interpreting statistical data and analyzing financial reports. This comprehensive guide delves deep into the world of percentages, exploring their calculation, applications, and significance.
What is a Percentage?
A percentage, denoted by the symbol %, represents a fraction of 100. It indicates how many parts of a whole are present out of 100 equal parts. For instance, 50% represents 50 out of 100, or half of a whole. Essentially, it's a convenient way to express a ratio where the second term is always 100.
Key Characteristics of Percentages:
- Standardized Comparison: Percentages allow for easy comparison between different quantities, even if they represent different whole amounts. For example, comparing a 10% increase in sales across two companies with vastly different initial sales figures is straightforward using percentages.
- Intuitive Understanding: The base of 100 makes percentages highly intuitive and readily understood by most people. It's much easier to grasp the concept of a 25% discount than a 1/4 discount.
- Wide Applicability: Percentages find application in numerous fields, including finance, statistics, science, and everyday life.
Calculating Percentages: Different Methods
There are several ways to calculate percentages, depending on the information available and the context of the problem.
Method 1: Using the Fraction Method
This is the most fundamental approach, directly reflecting the definition of a percentage as a fraction of 100.
Formula: (Part / Whole) * 100 = Percentage
Example: If you scored 45 out of 60 on a test, what's your percentage score?
(45 / 60) * 100 = 75%
Method 2: Using Decimal Multiplication
This method is efficient for quick calculations, particularly when dealing with decimal values.
Formula: Decimal * 100 = Percentage
Example: To express 0.75 as a percentage:
0.75 * 100 = 75%
Method 3: Using Proportions
This method is useful when you know a percentage and want to find the corresponding part or whole.
Formula: Percentage / 100 = Part / Whole
Example: If 20% of a number is 15, what is the number?
20 / 100 = 15 / x Solving for x gives x = 75
Applications of Percentages in Real Life
Percentages are ubiquitous in our daily lives, appearing in various contexts:
1. Finance and Business:
- Interest Rates: Banks and financial institutions use percentages to express interest rates on loans, savings accounts, and investments.
- Profit and Loss: Businesses calculate their profit margins and loss percentages to assess their financial performance.
- Discounts and Sales Tax: Retailers use percentages to offer discounts and calculate sales tax.
- Investment Returns: Investors track their investment returns using percentage gains or losses.
- Inflation and Economic Growth: Economists use percentages to measure inflation rates and economic growth.
2. Statistics and Data Analysis:
- Statistical Data Representation: Percentages are widely used to represent data in charts, graphs, and reports.
- Probability and Odds: Percentages are used to express probabilities and odds in various statistical contexts.
- Surveys and Polls: Results from surveys and polls are often expressed as percentages to show the proportion of respondents who chose a particular option.
3. Science and Engineering:
- Scientific Measurements: Percentages are used to express the concentration of solutions and other scientific measurements.
- Error Analysis: Scientists use percentages to express experimental errors and uncertainties.
- Efficiency Calculations: Engineers use percentages to evaluate the efficiency of various systems and processes.
4. Everyday Life:
- Tip Calculations: People use percentages to calculate tips in restaurants.
- Recipe Scaling: Cooks use percentages to scale recipes up or down.
- Grade Calculations: Students use percentages to understand their grades in school.
- Comparison Shopping: Consumers use percentages to compare prices and find the best deals.
Understanding Percentage Increase and Decrease
Calculating percentage change, whether an increase or decrease, is a common application of percentages.
Percentage Increase:
Formula: [(New Value - Old Value) / Old Value] * 100
Example: If the price of a product increased from $50 to $60, the percentage increase is:
[(60 - 50) / 50] * 100 = 20%
Percentage Decrease:
Formula: [(Old Value - New Value) / Old Value] * 100
Example: If the price of a product decreased from $60 to $48, the percentage decrease is:
[(60 - 48) / 60] * 100 = 20%
Common Percentage Calculations and Their Applications
Here are some frequently encountered percentage calculations and their real-world applications:
- Finding a Percentage of a Number: This is used to calculate discounts, sales tax, commissions, and tips. For example, finding 15% of $200 to calculate a discount.
- Finding the Percentage One Number is of Another: This is used to calculate grades, conversion rates, and market share. For example, determining what percentage 30 is of 150.
- Finding a Number When a Percentage is Known: This is used to determine the original price after a discount or to calculate the total amount given a percentage of a part. For example, finding the original price if a discounted price of $80 represents 80% of the original price.
- Calculating Percentage Change (Increase or Decrease): This is widely used in finance, economics, and statistics to track changes in values over time.
Advanced Applications and Considerations
Beyond the basic calculations, percentages play a crucial role in more complex scenarios:
- Compound Interest: Percentages are fundamental in calculating compound interest, where interest earned is added to the principal amount, and subsequent interest is calculated on the increased balance.
- Statistical Significance: In statistical analysis, percentages help determine the significance of observed results and the reliability of inferences.
- Financial Modeling: Sophisticated financial models heavily rely on percentages to project future financial performance and assess risk.
- Data Visualization: Percentages are essential for creating informative and visually appealing data visualizations such as pie charts and bar graphs.
Avoiding Common Mistakes with Percentages
Several common errors can arise when working with percentages. It's crucial to be mindful of these potential pitfalls:
- Using the Incorrect Base: Always ensure you are using the correct base value when calculating percentages. For instance, when calculating a percentage increase, the base is the original value.
- Confusing Percentage Points with Percentages: A change from 10% to 20% is a 10 percentage point increase, but a 100% percentage increase.
- Misinterpreting Percentage Changes: Percentage changes are not additive. A 10% increase followed by a 10% decrease does not result in the original value.
- Incorrect Rounding: Always round appropriately to maintain accuracy and avoid misleading results.
Conclusion
Percentages are a powerful tool for expressing proportions, making comparisons, and analyzing data. Understanding their calculation methods and applications is crucial for success in numerous fields, from personal finance to complex scientific research. By mastering the concepts discussed in this article and being aware of common pitfalls, you can effectively utilize percentages to interpret information, make informed decisions, and communicate your findings clearly and accurately. The versatility and widespread applicability of percentages make them an indispensable tool in our quantitative world.
Latest Posts
Latest Posts
-
Cuanto Es 100 Pies En Metros
Mar 31, 2025
-
How Much Is 6 Liters In Gallons
Mar 31, 2025
-
43 Grados Centigrados Convertirlos A Fahrenheit
Mar 31, 2025
-
How Many Minutes In 17 Hours
Mar 31, 2025
-
How Many Feet Is 161 Cm
Mar 31, 2025
Related Post
Thank you for visiting our website which covers about A Ratio That Compares A Number To 100 . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.
