Altitude Median Angle Bisector Perpendicular Bisector
Kalali
Apr 01, 2025 · 6 min read
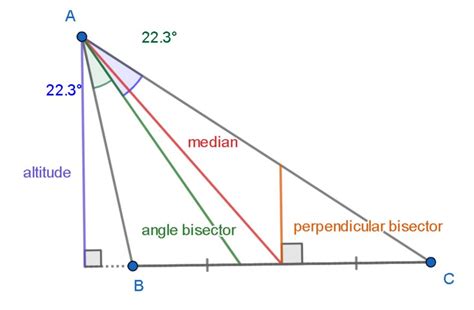
Table of Contents
Altitude, Median, Angle Bisector, and Perpendicular Bisector: A Comprehensive Guide
Understanding the fundamental concepts of altitude, median, angle bisector, and perpendicular bisector is crucial for mastering geometry. These lines hold unique properties within triangles and other geometric shapes, leading to various applications in problem-solving and advanced mathematical concepts. This comprehensive guide will delve deep into each of these lines, exploring their definitions, properties, unique characteristics, and how they relate to one another.
Defining the Lines
Before exploring their properties and relationships, let's clearly define each line segment within the context of a triangle:
1. Altitude
An altitude of a triangle is a line segment from a vertex perpendicular to the opposite side (or its extension). The point where the altitude intersects the opposite side (or its extension) is called the foot of the altitude. Every triangle has three altitudes, and they all meet at a single point called the orthocenter.
- Important Note: In an obtuse triangle, two altitudes fall outside the triangle. However, they are still defined as the perpendicular line segments from a vertex to the opposite side (extended if necessary).
2. Median
A median of a triangle is a line segment from a vertex to the midpoint of the opposite side. Every triangle has three medians, and they all intersect at a single point called the centroid. The centroid is the center of mass of the triangle; if you were to cut a triangle out of a uniform material, it would balance perfectly on a pin placed at the centroid.
3. Angle Bisector
An angle bisector of a triangle is a line segment that divides an angle into two congruent angles. Each angle in a triangle has an angle bisector. The three angle bisectors intersect at a single point called the incenter. The incenter is the center of the inscribed circle (incircle) of the triangle, meaning a circle can be drawn inside the triangle that is tangent to all three sides.
4. Perpendicular Bisector
A perpendicular bisector of a side of a triangle is a line segment that is perpendicular to a side and passes through its midpoint. Each side of a triangle has a perpendicular bisector. The three perpendicular bisectors intersect at a single point called the circumcenter. The circumcenter is the center of the circumscribed circle (circumcircle) of the triangle, meaning a circle can be drawn that passes through all three vertices of the triangle.
Properties and Relationships
Each of these lines has unique properties, and their interplay creates fascinating relationships within a triangle:
1. Altitude Properties
- Perpendicularity: The most defining property is its perpendicularity to the opposite side.
- Orthocenter: The three altitudes always concur (intersect at a single point) at the orthocenter.
- Right-angled Triangles: In a right-angled triangle, two altitudes coincide with the legs of the triangle, and the third altitude is the line segment from the right angle to the hypotenuse.
2. Median Properties
- Midpoint: A median connects a vertex to the midpoint of the opposite side.
- Centroid: The three medians intersect at the centroid, which divides each median into a 2:1 ratio. The distance from a vertex to the centroid is twice the distance from the centroid to the midpoint of the opposite side.
- Center of Mass: The centroid is the center of mass of the triangle.
3. Angle Bisector Properties
- Angle Division: An angle bisector divides the angle into two equal angles.
- Incenter: The three angle bisectors intersect at the incenter, which is equidistant from the three sides of the triangle.
- Incircle: The incenter is the center of the incircle, a circle tangent to all three sides of the triangle.
- Angle Bisector Theorem: This theorem states that the ratio of the lengths of the two segments created by the angle bisector on the opposite side is equal to the ratio of the lengths of the two sides forming the angle.
4. Perpendicular Bisector Properties
- Perpendicularity and Midpoint: A perpendicular bisector is perpendicular to a side and passes through its midpoint.
- Circumcenter: The three perpendicular bisectors intersect at the circumcenter, which is equidistant from the three vertices of the triangle.
- Circumcircle: The circumcenter is the center of the circumcircle, a circle passing through all three vertices of the triangle.
Interrelationships between the Lines
The four lines – altitude, median, angle bisector, and perpendicular bisector – are often studied together because of their interesting interrelationships. While they are generally distinct, certain types of triangles exhibit unique relationships:
-
Equilateral Triangles: In an equilateral triangle, the altitude, median, angle bisector, and perpendicular bisector from a vertex all coincide with each other. This is a unique property of equilateral triangles due to their symmetry.
-
Isosceles Triangles: In an isosceles triangle (a triangle with two equal sides), the altitude, median, and angle bisector from the vertex between the two equal sides coincide. The perpendicular bisector of the base is distinct but still holds its general properties.
-
Right-angled Triangles: In a right-angled triangle, the median to the hypotenuse is half the length of the hypotenuse. The circumcenter is the midpoint of the hypotenuse. The orthocenter lies at the right-angled vertex.
Applications and Advanced Concepts
Understanding altitudes, medians, angle bisectors, and perpendicular bisectors extends beyond basic geometry and finds applications in various fields:
- Computer Graphics: These lines are used in algorithms for triangulating surfaces, creating meshes for 3D models and simulations.
- Engineering and Architecture: Understanding the properties of these lines is vital in structural design and creating stable and balanced structures.
- Physics: The centroid plays a crucial role in calculating the center of mass and moments of inertia.
- Advanced Geometry: Concepts like the Euler line (connecting the orthocenter, centroid, and circumcenter) and the nine-point circle (a circle passing through nine significant points related to the triangle's geometry) rely heavily on these fundamental lines.
Problem-Solving and Examples
Let's consider a few examples illustrating the use of these lines in problem-solving:
Example 1: Given a triangle with vertices A, B, and C, and the lengths of its sides, find the coordinates of the centroid.
Solution: To find the centroid, you would calculate the average of the x-coordinates and the average of the y-coordinates of the vertices.
Example 2: Given an isosceles triangle, prove that the altitude from the vertex angle bisects the base.
Solution: This proof involves using congruent triangles created by the altitude, showcasing that the altitude divides the base into two equal segments.
Example 3: In a right-angled triangle, show that the circumcenter is the midpoint of the hypotenuse.
Solution: This proof uses the properties of perpendicular bisectors and the fact that the circumcircle passes through all three vertices.
Conclusion
Understanding the properties and interrelationships of altitudes, medians, angle bisectors, and perpendicular bisectors provides a solid foundation for more advanced geometric concepts. Their unique properties and applications make them essential elements in geometry, offering numerous problem-solving opportunities and insights into the beauty and logic of mathematical structures. By grasping these concepts and their applications, you enhance your geometrical reasoning skills and open doors to more complex mathematical explorations. Remember that practice is key to mastering these concepts; working through various problems and proofs will solidify your understanding and allow you to apply them effectively in different contexts.
Latest Posts
Latest Posts
-
Plants Carry Out Both Photosynthesis And Cellular Respiration
Apr 02, 2025
-
Bromine Readily Forms The Following Monatomic Ion
Apr 02, 2025
-
Cuanto Es 20 Libras En Kilos
Apr 02, 2025
-
The Shaft Of A Long Bone Is The
Apr 02, 2025
-
What Four Substances Are Recycled During Photosynthesis And Respiration
Apr 02, 2025
Related Post
Thank you for visiting our website which covers about Altitude Median Angle Bisector Perpendicular Bisector . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.
