Describe The Transformation Of The Parent Function
Kalali
Mar 30, 2025 · 6 min read
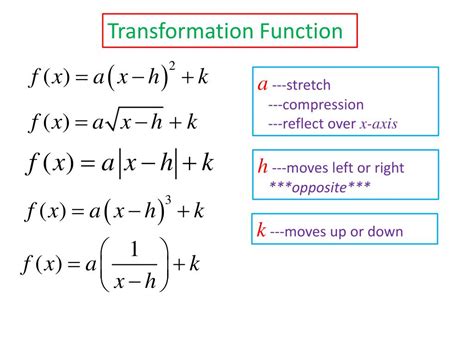
Table of Contents
Describing the Transformation of Parent Functions: A Comprehensive Guide
Understanding parent functions and their transformations is crucial for mastering algebra and calculus. Parent functions are the basic building blocks of many more complex functions. Learning how to manipulate these parent functions allows us to graph and analyze a wide variety of equations with ease. This comprehensive guide will delve into the core concepts, exploring various transformations and providing practical examples to solidify your understanding.
What are Parent Functions?
Parent functions are the simplest forms of function families. Each family has unique characteristics and a distinct graph. These functions serve as a foundation for understanding more complex functions. By recognizing the parent function, we can predict the shape and behavior of a more complex version. Here are some common parent functions:
- Linear: f(x) = x
- Quadratic: f(x) = x²
- Cubic: f(x) = x³
- Square Root: f(x) = √x
- Cube Root: f(x) = ³√x
- Absolute Value: f(x) = |x|
- Reciprocal: f(x) = 1/x
- Exponential: f(x) = a<sup>x</sup> (where a > 0 and a ≠ 1)
- Logarithmic: f(x) = log<sub>a</sub>(x) (where a > 0 and a ≠ 1)
Transformations of Parent Functions: The Key Players
Transformations alter the graph of a parent function, shifting, stretching, compressing, or reflecting it. These transformations can be categorized as:
1. Vertical Shifts
A vertical shift moves the entire graph up or down along the y-axis. This is achieved by adding or subtracting a constant value to the function:
- f(x) + k: Shifts the graph k units upwards. If k is positive, the shift is upwards; if k is negative, the shift is downwards.
Example: If f(x) = x², then f(x) + 3 = x² + 3 shifts the parabola three units upwards.
2. Horizontal Shifts
A horizontal shift moves the graph left or right along the x-axis. This is achieved by adding or subtracting a constant value within the function's argument:
- f(x - h): Shifts the graph h units to the right. Note that a positive h shifts it to the right, while a negative h shifts it to the left. This is often counter-intuitive to beginners.
Example: If f(x) = √x, then f(x - 2) = √(x - 2) shifts the square root graph two units to the right.
3. Vertical Stretches and Compressions
Vertical stretches or compressions scale the graph vertically, making it taller or shorter. This is achieved by multiplying the function by a constant value:
- af(x): If |a| > 1, the graph is stretched vertically by a factor of |a|. If 0 < |a| < 1, the graph is compressed vertically by a factor of |a|. If a is negative, there's also a reflection across the x-axis.
Example: If f(x) = x³, then 2f(x) = 2x³ stretches the cubic function vertically by a factor of 2. 1/2f(x) = (1/2)x³ compresses it vertically by a factor of 1/2.
4. Horizontal Stretches and Compressions
Horizontal stretches or compressions scale the graph horizontally, making it wider or narrower. This is achieved by multiplying the x within the function by a constant value:
- f(bx): If |b| > 1, the graph is compressed horizontally by a factor of 1/|b|. If 0 < |b| < 1, the graph is stretched horizontally by a factor of 1/|b|. If b is negative, there's also a reflection across the y-axis.
Example: If f(x) = |x|, then f(2x) = |2x| compresses the absolute value function horizontally by a factor of 1/2. f(1/2x) = |(1/2)x| stretches it horizontally by a factor of 2.
5. Reflections
Reflections flip the graph across an axis.
- -f(x): Reflects the graph across the x-axis (vertical reflection).
- f(-x): Reflects the graph across the y-axis (horizontal reflection).
Example: If f(x) = x², then -f(x) = -x² reflects the parabola across the x-axis, and f(-x) = (-x)² = x² which means the parabola remains unchanged under y-axis reflection (due to its symmetry).
Combining Transformations
Often, multiple transformations are applied to a parent function simultaneously. The order of operations is crucial when applying multiple transformations. Generally, the order is:
- Horizontal Shifts: Apply any horizontal shifts (h).
- Horizontal Stretches/Compressions: Apply any horizontal stretches or compressions (b).
- Reflections: Apply any reflections.
- Vertical Stretches/Compressions: Apply any vertical stretches or compressions (a).
- Vertical Shifts: Apply any vertical shifts (k).
This order ensures the transformations are applied correctly and produces the expected result.
Examples of Combined Transformations
Let's explore some examples illustrating the combination of multiple transformations:
Example 1: Consider the function g(x) = -2(x + 1)² - 3. This is a transformation of the parent function f(x) = x².
- Horizontal Shift: (x + 1) shifts the graph one unit to the left.
- Vertical Stretch: The 2 stretches the graph vertically by a factor of 2.
- Reflection across the x-axis: The negative sign reflects the graph across the x-axis.
- Vertical Shift: The -3 shifts the graph three units down.
Example 2: Consider the function h(x) = 1/2(x - 3)³ + 2. This is a transformation of the parent function f(x) = x³.
- Horizontal Shift: (x - 3) shifts the graph three units to the right.
- Vertical Compression: The 1/2 compresses the graph vertically by a factor of 1/2.
- Vertical Shift: The +2 shifts the graph two units up.
Applying Transformations to Different Parent Functions
The principles of transformations apply consistently across all parent functions. However, the visual effects will vary depending on the parent function's inherent shape and characteristics. Understanding the unique features of each parent function is crucial for accurate visualization and interpretation of transformations.
Advanced Transformations and Considerations
Beyond the basic transformations, there are more advanced transformations involving rotations, shears, and other more complex geometric manipulations. These are usually covered in higher-level mathematics courses. Moreover, it is important to remember that transformations affect the domain and range of the function. Understanding how transformations change the domain and range is key to a complete understanding of the transformed function.
Conclusion
Mastering the transformation of parent functions is a cornerstone of mathematical understanding. By thoroughly comprehending the effects of vertical and horizontal shifts, stretches, compressions, and reflections, we can analyze and graph a vast array of functions with efficiency and accuracy. Remember to practice consistently, applying these principles to various examples to build a solid understanding. The ability to visualize and predict the effects of transformations is a powerful tool that greatly enhances your problem-solving skills in mathematics. Further exploration into advanced transformation techniques will solidify your mathematical foundations and open doors to more complex mathematical concepts.
Latest Posts
Latest Posts
-
12 Out Of 14 As A Percentage
Apr 01, 2025
-
How Many Feet Is 17 Meters
Apr 01, 2025
-
How Much Is 24 Oz In Cups
Apr 01, 2025
-
How Much Is 5 Quarts In Cups
Apr 01, 2025
-
How Many Cups Are In 6 Ounces
Apr 01, 2025
Related Post
Thank you for visiting our website which covers about Describe The Transformation Of The Parent Function . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.
