Determine The Theoretical Yield Of Pbcl2
Kalali
Apr 06, 2025 · 6 min read
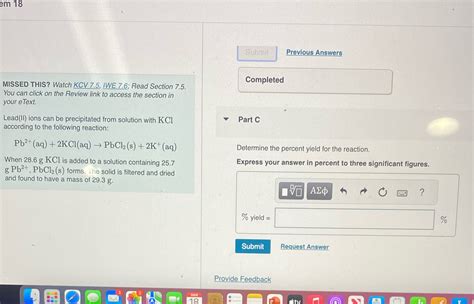
Table of Contents
Determining the Theoretical Yield of PbCl₂: A Comprehensive Guide
Determining the theoretical yield of lead(II) chloride (PbCl₂) is a fundamental exercise in stoichiometry, a cornerstone of chemistry. Understanding this process not only helps you ace your chemistry exams but also provides a crucial foundation for various applications in chemical engineering and research. This comprehensive guide will walk you through the process, explaining the concepts, calculations, and potential pitfalls to avoid. We'll cover everything from understanding molar masses to accounting for limiting reagents, ensuring you have a firm grasp of this vital chemical concept.
Understanding Theoretical Yield
Before delving into the specifics of PbCl₂ calculation, let's establish a clear understanding of theoretical yield. Theoretical yield represents the maximum amount of product that can be formed in a chemical reaction, assuming complete conversion of the limiting reactant. It's a calculated value based on the stoichiometry of the balanced chemical equation and the amount of reactants used. In reality, the actual yield (the amount of product actually obtained) is often lower due to various factors like incomplete reactions, side reactions, and losses during purification.
The Balanced Chemical Equation: The Foundation of Stoichiometry
The first step in determining the theoretical yield of any product is to write and balance the relevant chemical equation. For PbCl₂, a common method of production involves reacting lead(II) nitrate (Pb(NO₃)₂) with sodium chloride (NaCl) in an aqueous solution. The balanced equation is:
Pb(NO₃)₂(aq) + 2NaCl(aq) → PbCl₂(s) + 2NaNO₃(aq)
This equation tells us that one mole of lead(II) nitrate reacts with two moles of sodium chloride to produce one mole of lead(II) chloride and two moles of sodium nitrate. The (aq) denotes aqueous solutions, and (s) indicates a solid precipitate. The balanced equation provides the crucial mole ratios needed for stoichiometric calculations.
Determining Molar Masses: The Key to Conversion
Molar mass is the mass of one mole of a substance, expressed in grams per mole (g/mol). To calculate the theoretical yield, you need the molar masses of the reactants and the product. These values can be found on the periodic table by summing the atomic masses of each atom in the chemical formula.
- Pb(NO₃)₂: The molar mass of lead(II) nitrate is approximately 331.2 g/mol (207.2 g/mol for Pb + 2 * (14.0 g/mol for N + 3 * 16.0 g/mol for O)).
- NaCl: The molar mass of sodium chloride is approximately 58.5 g/mol (23.0 g/mol for Na + 35.5 g/mol for Cl).
- PbCl₂: The molar mass of lead(II) chloride is approximately 278.1 g/mol (207.2 g/mol for Pb + 2 * 35.5 g/mol for Cl).
These molar masses are essential for converting between grams and moles, a crucial step in stoichiometric calculations.
Identifying the Limiting Reagent: The Bottleneck of the Reaction
In many reactions, one reactant is completely consumed before the others. This reactant is called the limiting reagent, and it dictates the maximum amount of product that can be formed. The other reactants are considered to be in excess. To identify the limiting reagent, we need to compare the mole ratios of the reactants to the stoichiometric ratios in the balanced equation.
Let's say we have 10 grams of Pb(NO₃)₂ and 5 grams of NaCl. To determine the limiting reagent:
-
Convert grams to moles:
- Moles of Pb(NO₃)₂ = (10 g) / (331.2 g/mol) ≈ 0.030 moles
- Moles of NaCl = (5 g) / (58.5 g/mol) ≈ 0.085 moles
-
Compare mole ratios: The balanced equation shows a 1:2 mole ratio of Pb(NO₃)₂ to NaCl. We have 0.030 moles of Pb(NO₃)₂. To react completely, we would need 2 * 0.030 moles = 0.060 moles of NaCl. Since we have 0.085 moles of NaCl, there is an excess of NaCl. Therefore, Pb(NO₃)₂ is the limiting reagent.
Calculating Theoretical Yield: Putting it All Together
Now that we've identified the limiting reagent (Pb(NO₃)₂), we can calculate the theoretical yield of PbCl₂. The balanced equation tells us that 1 mole of Pb(NO₃)₂ produces 1 mole of PbCl₂. Therefore, 0.030 moles of Pb(NO₃)₂ will produce 0.030 moles of PbCl₂.
Finally, we convert moles of PbCl₂ back to grams:
Grams of PbCl₂ = (0.030 moles) * (278.1 g/mol) ≈ 8.34 g
Therefore, the theoretical yield of PbCl₂ in this example is approximately 8.34 grams.
Factors Affecting Actual Yield and Percent Yield
The theoretical yield is a calculated value; the actual yield obtained in a laboratory setting is often lower. Several factors contribute to this discrepancy:
- Incomplete Reactions: Not all reactants may react completely. Equilibrium limitations, slow reaction rates, or insufficient reaction time can lead to lower yields.
- Side Reactions: Unwanted side reactions can consume reactants, reducing the amount available for the desired reaction.
- Loss of Product: During the experimental process, some product might be lost due to spillage, transfer losses, or incomplete separation and purification.
- Impurities in Reactants: The presence of impurities in the starting materials can affect the reaction efficiency.
To assess the efficiency of a reaction, we calculate the percent yield:
Percent Yield = (Actual Yield / Theoretical Yield) * 100%
For example, if the actual yield of PbCl₂ in our experiment was 7.5 grams, the percent yield would be:
Percent Yield = (7.5 g / 8.34 g) * 100% ≈ 90%
A high percent yield (close to 100%) indicates an efficient reaction, while a low percent yield suggests potential problems with the experimental procedure or the reaction itself.
Advanced Considerations: Purity and Error Analysis
In real-world scenarios, the purity of reactants and products needs to be considered. If the Pb(NO₃)₂ used was only 95% pure, this would reduce the effective amount available for the reaction, leading to a lower theoretical yield. Similarly, impurities in the collected PbCl₂ will affect the actual yield and the calculated percent yield.
Furthermore, a thorough error analysis is crucial. Identify potential sources of error in the experiment, such as measurement uncertainties in weighing the reactants and the product, and consider their impact on the final result. Reporting uncertainties associated with the theoretical yield is essential for accurate scientific communication.
Conclusion: Mastering Stoichiometry for Accurate Predictions
Determining the theoretical yield of PbCl₂ involves a systematic approach that builds upon fundamental stoichiometric principles. By understanding the balanced chemical equation, molar masses, limiting reagents, and potential sources of error, you can accurately predict the maximum amount of product achievable in a chemical reaction. Mastering this skill is not just about passing exams; it’s about developing a crucial understanding of chemical processes that extends to numerous applications in various scientific and industrial fields. Remember, meticulous experimental work and careful attention to detail are critical for maximizing yields and obtaining reliable results.
Latest Posts
Latest Posts
-
What Is 9 16 In Decimal
Apr 08, 2025
-
What Is The Reciprocal Of 5
Apr 08, 2025
-
How Many Grams Are In 2 2 Pounds
Apr 08, 2025
-
How Many Feet And Inches Is 130 Cm
Apr 08, 2025
-
How Many Calories Are In Uranium 235
Apr 08, 2025
Related Post
Thank you for visiting our website which covers about Determine The Theoretical Yield Of Pbcl2 . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.