El 10 Por Ciento De 1000
Kalali
Mar 26, 2025 · 5 min read
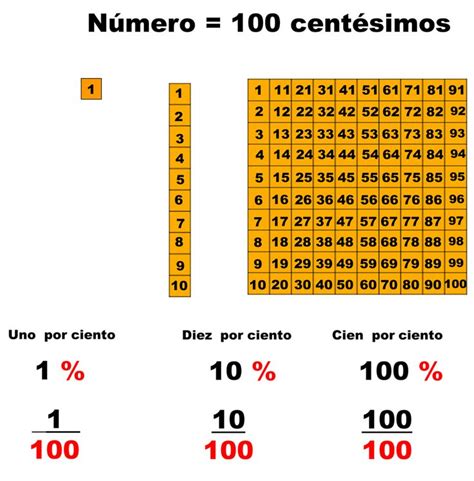
Table of Contents
What is 10% of 1000? A Deep Dive into Percentages and Their Applications
Finding 10% of 1000 is a fundamental calculation that forms the basis of many real-world applications. Understanding how to calculate percentages efficiently is crucial for various tasks, from managing personal finances to analyzing business data. This article will explore the simple method of calculating 10% of 1000, delve into the broader concept of percentages, and showcase their practical applications in everyday life.
Calculating 10% of 1000: The Simple Method
The simplest way to calculate 10% of 1000 is to remember that a percentage is simply a fraction out of 100. Therefore, 10% can be expressed as 10/100, which simplifies to 1/10. To find 10% of 1000, we simply multiply 1000 by 1/10:
1000 * (1/10) = 100
Therefore, 10% of 1000 is 100.
This method is incredibly efficient, especially when dealing with multiples of 10. It highlights the direct relationship between percentages and fractions.
Understanding Percentages: A Deeper Look
Percentages are a fundamental concept in mathematics and are used extensively in various fields. They represent a fraction of a whole, expressed as a number out of 100. The symbol "%" denotes a percentage.
Several key aspects of percentages are:
-
Representing Parts of a Whole: Percentages are invaluable for representing parts of a whole, whether it's a portion of a population, a discount on a product, or a change in a value. For example, a 20% increase in sales indicates that sales have grown by 20 out of every 100 units.
-
Comparing Quantities: Percentages provide a standardized way to compare quantities, even if they are of different magnitudes. For instance, comparing a 10% increase in profit for a small business to a 5% increase in profit for a large corporation allows for relative comparison regardless of the absolute profit figures.
-
Expressing Ratios: Percentages can also represent ratios. A success rate of 50% signifies that for every 100 attempts, 50 were successful.
-
Calculations and Applications: Percentage calculations are employed across diverse areas, including:
- Finance: Calculating interest rates, discounts, taxes, profit margins, and returns on investments.
- Statistics: Representing data distributions, probabilities, and changes in variables.
- Business: Analyzing sales figures, market shares, and growth rates.
- Science: Expressing experimental results, error margins, and concentrations.
Methods for Calculating Percentages
While the 10% of 1000 calculation was straightforward, various methods can be used for calculating percentages, especially for more complex scenarios:
-
Using the Decimal Equivalent: Convert the percentage to its decimal equivalent by dividing by 100. For example, 25% becomes 0.25. Then, multiply the decimal by the number. To find 25% of 500, calculate 0.25 * 500 = 125.
-
Using Proportions: Set up a proportion to solve for the unknown percentage. For example, to find x% of 800 that equals 160, set up the proportion: x/100 = 160/800. Solve for x to find the percentage.
-
Using a Calculator: Calculators have a percentage function (%) that simplifies calculations significantly. Simply enter the number, press the percentage button, and then enter the percentage.
-
Using Online Percentage Calculators: Numerous online tools can calculate percentages quickly and accurately. These calculators are particularly useful for complex percentage calculations or for users who are not comfortable with manual calculations.
Real-World Applications of Percentage Calculations
The ability to calculate percentages effectively extends far beyond simple mathematical exercises. Here are some real-world examples demonstrating their practical value:
-
Personal Finance:
- Calculating Savings: If you want to save 15% of your monthly income, knowing how to calculate percentages helps determine the amount you need to set aside.
- Budgeting: Percentages help allocate your budget effectively across different categories like housing, food, transportation, and entertainment.
- Investment Returns: Understanding percentage returns on investments is vital for making informed financial decisions.
- Discounts and Sales Tax: Calculating discounts and sales tax accurately ensures you understand the final cost of purchases.
-
Business and Economics:
- Profit Margins: Businesses calculate profit margins (percentage of profit relative to revenue) to assess their financial health.
- Market Share: Analyzing market share (percentage of a market controlled by a company) helps businesses strategize for growth.
- Growth Rates: Tracking percentage growth in sales, profits, or market share enables businesses to monitor their performance.
- Inflation Rates: Understanding inflation (percentage increase in the price of goods and services) helps in economic forecasting and decision-making.
-
Science and Statistics:
- Data Analysis: Percentages are crucial for representing data distributions, calculating probabilities, and understanding statistical significance.
- Experimental Results: Scientists express experimental results as percentages to standardize and compare their findings.
- Error Margins: Percentages help quantify the uncertainty associated with experimental measurements.
Advanced Percentage Calculations and Concepts
While calculating 10% of 1000 is a basic operation, more advanced concepts related to percentages exist:
-
Percentage Change: This calculates the increase or decrease in a value expressed as a percentage. The formula is: [(New Value - Old Value) / Old Value] * 100.
-
Percentage Point Change: This is the difference between two percentages. For example, an increase from 20% to 25% is a 5 percentage point increase, not a 25% increase.
-
Compound Interest: This involves calculating interest on both the principal amount and accumulated interest from previous periods. Compound interest significantly affects long-term investments.
-
Percentage Increase/Decrease: Calculating percentage increase or decrease is vital for understanding growth or decline in various metrics like sales, profits, or populations. For instance, a 10% increase in a quantity of 1000 would be calculated as 1000 * 0.10 = 100, resulting in a new quantity of 1100. Conversely, a 10% decrease would result in a new quantity of 900.
Conclusion: The Ubiquity of Percentages
The simple calculation of 10% of 1000 serves as a gateway to understanding the broader significance of percentages in our daily lives. From managing personal finances to analyzing complex business data, the ability to perform percentage calculations accurately and efficiently is an indispensable skill. Mastering these concepts allows for informed decision-making across diverse fields and empowers individuals to navigate the quantitative aspects of the world effectively. Whether it's understanding discounts, analyzing financial reports, or interpreting scientific data, percentages are an integral part of understanding and interpreting numerical information in a meaningful way. The foundation of this understanding begins with simple calculations like finding 10% of 1000, a building block that leads to a deeper comprehension of percentages' power and versatility.
Latest Posts
Latest Posts
-
What Percentage Is 15 Of 75
Mar 29, 2025
-
How Many Feet Are 63 Inches
Mar 29, 2025
-
How Many Ft Is 22 Inches
Mar 29, 2025
-
38 Cm Is Equal To How Many Inches
Mar 29, 2025
-
3000 Mg Is How Many Grams
Mar 29, 2025
Related Post
Thank you for visiting our website which covers about El 10 Por Ciento De 1000 . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.
