Equations With Variable On Both Sides
Kalali
Apr 03, 2025 · 5 min read
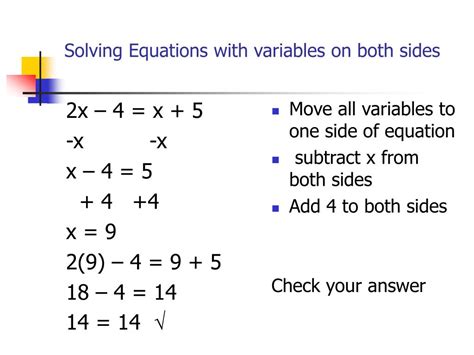
Table of Contents
Equations with Variables on Both Sides: A Comprehensive Guide
Solving equations is a fundamental skill in algebra. While simple equations might involve variables only on one side of the equals sign, many real-world problems translate into equations with variables appearing on both sides. This comprehensive guide will equip you with the strategies and understanding needed to confidently tackle these more complex equations. We'll cover various techniques, provide numerous examples, and explore common pitfalls to avoid.
Understanding the Basics
Before diving into solving equations with variables on both sides, let's solidify our understanding of fundamental concepts. An equation is a mathematical statement that indicates two expressions are equal. The equals sign (=) separates the two expressions. A variable is a symbol, typically a letter (like x, y, or z), representing an unknown value. The goal when solving an equation is to find the value of the variable that makes the equation true.
For instance, in the equation 3x + 5 = 14, 'x' is the variable, and we need to determine the value of 'x' that satisfies the equation. In contrast, an equation like 2x + 7 = x + 12 has the variable 'x' on both sides of the equals sign. This is the type of equation we'll focus on in this guide.
Strategies for Solving Equations with Variables on Both Sides
The core principle behind solving these equations is to isolate the variable on one side of the equals sign. This involves applying inverse operations (addition/subtraction, multiplication/division) to both sides of the equation, maintaining balance. Here's a step-by-step approach:
1. Simplify Each Side
Begin by simplifying each side of the equation independently. This involves combining like terms (terms with the same variable raised to the same power).
Example:
2x + 5 + x = 3x + 11 - x
Simplify the left side: 3x + 5
Simplify the right side: 2x + 11
The equation becomes: 3x + 5 = 2x + 11
2. Eliminate the Variable from One Side
Next, choose either side of the equation and eliminate the variable from it. This usually involves subtracting the term containing the variable from both sides. Remember to perform the same operation on both sides to maintain the equation's balance.
Continuing the Example:
Let's eliminate the '2x' from the right side:
Subtract 2x from both sides: 3x + 5 - 2x = 2x + 11 - 2x
This simplifies to: x + 5 = 11
3. Isolate the Variable
Now, focus on isolating the variable by performing inverse operations. In this case, we need to remove the '+5' from the left side.
Subtract 5 from both sides: x + 5 - 5 = 11 - 5
This simplifies to: x = 6
Therefore, the solution to the equation 2x + 5 + x = 3x + 11 - x is x = 6.
Working with Different Equation Structures
Let's explore how to handle variations in equation structures:
Equations with Fractions
Equations with fractions can appear intimidating, but they are solved using the same fundamental principles. The key is to eliminate the fractions by finding a common denominator and multiplying both sides of the equation by it.
Example:
(x/2) + 3 = (x/4) + 5
Find the common denominator (4):
Multiply both sides by 4: 4 * ((x/2) + 3) = 4 * ((x/4) + 5)
Simplify: 2x + 12 = x + 20
Now, solve this equation using the steps outlined previously:
Subtract 'x' from both sides: x + 12 = 20
Subtract 12 from both sides: x = 8
Equations with Parentheses
Equations with parentheses require careful application of the distributive property before proceeding with other steps.
Example:
2(x + 3) = 4x - 2
Distribute the 2 on the left side: 2x + 6 = 4x - 2
Subtract 2x from both sides: 6 = 2x - 2
Add 2 to both sides: 8 = 2x
Divide both sides by 2: x = 4
Equations with Decimals
Equations with decimals can be solved directly, but many find it easier to eliminate the decimals by multiplying both sides by a power of 10.
Example:
0.5x + 1.2 = 0.2x + 3.7
Multiply both sides by 10: 5x + 12 = 2x + 37
Subtract 2x from both sides: 3x + 12 = 37
Subtract 12 from both sides: 3x = 25
Divide both sides by 3: x = 25/3 or approximately 8.33
Checking Your Solutions
After finding a solution, it's crucial to check your work. Substitute the value you found for the variable back into the original equation. If both sides of the equation are equal, your solution is correct.
Example (using x = 6 from the first example):
Original equation: 2x + 5 + x = 3x + 11 - x
Substitute x = 6: 2(6) + 5 + 6 = 3(6) + 11 - 6
Simplify: 12 + 5 + 6 = 18 + 11 - 6
Simplify further: 23 = 23
Since both sides are equal, our solution x = 6 is correct.
Common Mistakes to Avoid
- Incorrectly applying the distributive property: Pay close attention to signs when distributing.
- Forgetting to perform the same operation on both sides: This will disrupt the equation's balance and lead to an incorrect solution.
- Making errors in arithmetic: Double-check your calculations to avoid simple mistakes.
- Not checking your solution: Always substitute your answer back into the original equation to verify its accuracy.
Advanced Applications and Extensions
The techniques discussed above form the foundation for solving a vast array of more complex equations. These include:
- Systems of equations: Equations with multiple variables that must be solved simultaneously.
- Quadratic equations: Equations where the variable is raised to the power of 2.
- Inequalities: Equations involving inequality symbols (<, >, ≤, ≥). Solving inequalities involves similar techniques, but with some crucial differences related to reversing the inequality sign when multiplying or dividing by a negative number.
Conclusion
Solving equations with variables on both sides is a crucial algebraic skill with numerous practical applications. By mastering the strategies outlined in this guide, understanding the fundamental concepts, and practicing regularly, you will develop confidence and proficiency in tackling these types of equations. Remember to always simplify, maintain balance, and check your solution to ensure accuracy. With consistent practice and attention to detail, you’ll successfully navigate the world of equations and unlock the power of algebra.
Latest Posts
Latest Posts
-
39 Fahrenheit Is What In Celsius
Apr 04, 2025
-
1 3 Cup Equals How Many Ounces
Apr 04, 2025
-
What Is 68 Cm In Inches
Apr 04, 2025
-
166 Cm To Feet And Inches
Apr 04, 2025
-
1 1 2 Cup Of Water
Apr 04, 2025
Related Post
Thank you for visiting our website which covers about Equations With Variable On Both Sides . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.
