First Derivative And Second Derivative Test
Kalali
Apr 05, 2025 · 6 min read
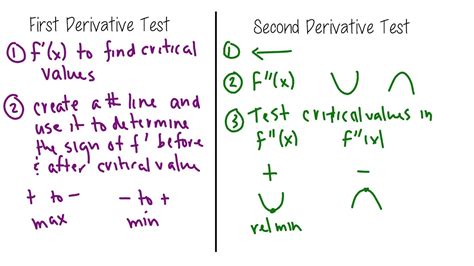
Table of Contents
First Derivative and Second Derivative Tests: A Comprehensive Guide
The first and second derivative tests are crucial tools in calculus used to analyze the behavior of functions, specifically to identify critical points, determine the intervals where a function is increasing or decreasing, and classify these critical points as local maxima, local minima, or saddle points. Understanding these tests is fundamental for sketching accurate graphs, solving optimization problems, and gaining a deeper insight into the nature of functions. This comprehensive guide will delve into both tests, providing clear explanations, examples, and practical applications.
Understanding the First Derivative Test
The first derivative test utilizes the sign of the first derivative, f'(x), to determine the function's increasing and decreasing intervals and to identify potential local extrema (maximum or minimum values). The key principle is that:
- If f'(x) > 0 on an interval, then f(x) is increasing on that interval. This means the function's value is rising as x increases.
- If f'(x) < 0 on an interval, then f(x) is decreasing on that interval. The function's value is falling as x increases.
- If f'(x) = 0 or f'(x) is undefined, then x is a critical point. These points are candidates for local maxima or minima. Note that not all critical points represent extrema.
Steps to Apply the First Derivative Test:
-
Find the first derivative, f'(x). This often involves using the power rule, product rule, quotient rule, or chain rule, depending on the complexity of the function.
-
Find the critical points. Solve the equation f'(x) = 0 and identify any values of x where f'(x) is undefined (e.g., where the denominator of a rational function is zero).
-
Analyze the sign of f'(x) in the intervals determined by the critical points. Choose a test point within each interval and evaluate the sign of f'(x) at that point.
-
Determine increasing and decreasing intervals. Based on the sign analysis, identify the intervals where f'(x) > 0 (increasing) and f'(x) < 0 (decreasing).
-
Classify critical points. If f'(x) changes from positive to negative at a critical point, it's a local maximum. If it changes from negative to positive, it's a local minimum. If the sign doesn't change, it's neither a local maximum nor minimum (it could be a saddle point or an inflection point).
Example:
Let's consider the function f(x) = x³ - 3x.
-
f'(x) = 3x² - 3
-
Critical points: Setting f'(x) = 0, we get 3x² - 3 = 0, which gives x = ±1.
-
Sign analysis:
- For x < -1, f'(x) > 0 (e.g., f'(-2) = 9 > 0)
- For -1 < x < 1, f'(x) < 0 (e.g., f'(0) = -3 < 0)
- For x > 1, f'(x) > 0 (e.g., f'(2) = 9 > 0)
-
Intervals: f(x) is increasing on (-∞, -1) and (1, ∞); f(x) is decreasing on (-1, 1).
-
Classification: At x = -1, f'(x) changes from positive to negative, indicating a local maximum. At x = 1, f'(x) changes from negative to positive, indicating a local minimum.
Understanding the Second Derivative Test
The second derivative test provides an alternative method for classifying critical points as local maxima or minima. It uses the sign of the second derivative, f''(x), evaluated at the critical point. The test relies on the concept of concavity:
- If f''(x) > 0, the function is concave up (shaped like a U).
- If f''(x) < 0, the function is concave down (shaped like an upside-down U).
Steps to Apply the Second Derivative Test:
-
Find the first derivative, f'(x), and the second derivative, f''(x).
-
Find the critical points. Solve f'(x) = 0.
-
Evaluate f''(x) at each critical point.
-
Classify critical points:
- If f''(x) > 0 at a critical point, the critical point is a local minimum. The function is concave up at this point.
- If f''(x) < 0 at a critical point, the critical point is a local maximum. The function is concave down at this point.
- If f''(x) = 0, the second derivative test is inconclusive. In this case, you must resort to the first derivative test or further analysis.
Example (using the same function as before):
f(x) = x³ - 3x
-
f'(x) = 3x² - 3; f''(x) = 6x
-
Critical points: x = ±1
-
Second derivative evaluation:
- At x = -1, f''(-1) = -6 < 0, indicating a local maximum.
- At x = 1, f''(1) = 6 > 0, indicating a local minimum.
Comparing the First and Second Derivative Tests
Both tests serve the same purpose—classifying critical points—but they have different strengths and weaknesses:
-
First Derivative Test: Always works (provided you can analyze the sign changes). It's more general and can handle cases where the second derivative test fails (f''(x) = 0 at a critical point). However, it often requires more work than the second derivative test.
-
Second Derivative Test: Simpler to apply if the second derivative is easy to compute and evaluate. However, it's inconclusive when f''(x) = 0 at a critical point, requiring a fallback to the first derivative test.
Applications of the Derivative Tests
The first and second derivative tests have numerous applications across various fields:
-
Optimization Problems: Finding maximum or minimum values in engineering, economics, physics, and other disciplines. For instance, finding the dimensions of a container that minimize material usage or maximizing profit given certain constraints.
-
Curve Sketching: Accurately sketching graphs of functions by identifying increasing/decreasing intervals, concavity, and local extrema. This improves the understanding of a function's behavior.
-
Analysis of Functions: Determining the behavior of functions, such as identifying inflection points (where concavity changes) and regions of increasing/decreasing rates of change.
-
Physics: Analyzing motion (velocity and acceleration) where the first derivative represents velocity and the second derivative represents acceleration.
Advanced Considerations and Limitations
-
Inflection Points: While the second derivative test helps classify extrema, the second derivative itself helps find inflection points, points where the concavity of a function changes. These occur when f''(x) = 0 and the sign of f''(x) changes around that point.
-
Higher-Order Derivatives: While less commonly used, higher-order derivatives can provide further insights into the function's behavior, although interpretation becomes increasingly complex.
-
Functions with Multiple Critical Points: For functions with many critical points, the application of either test might be more computationally intensive. Systematic organization and careful analysis become crucial.
Conclusion
The first and second derivative tests are indispensable tools in calculus. Mastering these tests enhances your ability to analyze functions, solve optimization problems, and gain a deeper understanding of mathematical relationships. While the second derivative test offers a quicker method in many cases, the first derivative test remains a reliable fallback when the second derivative test is inconclusive. Understanding both tests, along with their limitations, is essential for a comprehensive understanding of function analysis. Remember to always choose the most efficient and reliable test given the context of the problem. Through careful application and consistent practice, these techniques become powerful tools for solving complex mathematical problems and interpreting real-world phenomena.
Latest Posts
Latest Posts
-
How Long Does It Take For Brandy Melville To Ship
Jul 13, 2025
-
How Many Times Does 3 Go Into 30
Jul 13, 2025
-
In What Episode Of Bleach Does Ichigo Ask Orihime Out
Jul 13, 2025
-
How Much Is 4 Oz Chocolate Chips
Jul 13, 2025
-
How Many Times Does 9 Go Into 70
Jul 13, 2025
Related Post
Thank you for visiting our website which covers about First Derivative And Second Derivative Test . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.