How Do You Find The Mean On A Dot Plot
Kalali
Apr 03, 2025 · 5 min read
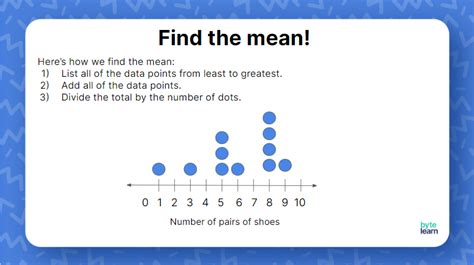
Table of Contents
How to Find the Mean on a Dot Plot: A Comprehensive Guide
Dot plots, also known as dot diagrams, are simple yet powerful statistical tools used to represent data visually. They're particularly useful for showcasing the distribution of a dataset, identifying clusters, and quickly grasping the central tendency. One key measure of central tendency is the mean, often referred to as the average. While seemingly straightforward, calculating the mean from a dot plot requires a systematic approach. This comprehensive guide will walk you through the process, providing clear explanations and examples to help you master this essential statistical skill.
Understanding Dot Plots and the Mean
Before diving into the calculation, let's solidify our understanding of the core concepts:
What is a Dot Plot?
A dot plot is a graph that displays data values as dots above a number line. Each dot represents a single data point. The frequency of a data point is shown by the number of dots stacked vertically above that point on the number line. This visual representation allows for a quick assessment of the distribution of data, showing where data points are concentrated and identifying any outliers.
What is the Mean?
The mean, or average, is a measure of central tendency that represents the typical value of a dataset. It's calculated by summing all the data values and then dividing by the total number of data points. The mean provides a single value that summarizes the overall tendency of the data.
Calculating the Mean from a Dot Plot: A Step-by-Step Approach
Calculating the mean from a dot plot involves several steps:
1. Identify the Data Values:
Begin by examining the horizontal axis of your dot plot. This axis represents the data values. Note each distinct data value present in the plot.
2. Count the Frequency of Each Data Value:
For each data value, count the number of dots stacked above it. This count represents the frequency of that specific data value in the dataset.
3. Create a Frequency Table:
Organize your findings into a frequency table. This table will list each data value and its corresponding frequency. This step simplifies the calculation significantly, especially when dealing with large datasets. For instance:
| Data Value | Frequency |
|---|---|
| 2 | 1 |
| 3 | 3 |
| 4 | 5 |
| 5 | 4 |
| 6 | 2 |
4. Calculate the Sum of All Data Values:
To find the total sum of all data values, multiply each data value by its frequency and then sum up these products. Using our example:
(2 * 1) + (3 * 3) + (4 * 5) + (5 * 4) + (6 * 2) = 2 + 9 + 20 + 20 + 12 = 63
5. Count the Total Number of Data Points:
Sum the frequencies from your frequency table to determine the total number of data points in the dataset. In our example:
1 + 3 + 5 + 4 + 2 = 15
6. Calculate the Mean:
Finally, divide the sum of all data values (step 4) by the total number of data points (step 5) to calculate the mean.
Mean = 63 / 15 = 4.2
Example: Calculating the Mean from a Dot Plot of Test Scores
Let's consider a real-world example. Suppose a teacher uses a dot plot to represent the test scores of their students:
(Imagine a dot plot here with scores ranging from 60 to 100, with varying numbers of dots above each score. For the purpose of this example, let's assume the following data):
- 60: 1 dot
- 70: 3 dots
- 80: 6 dots
- 90: 4 dots
- 100: 2 dots
1. Data Values: 60, 70, 80, 90, 100
2. Frequencies: 1, 3, 6, 4, 2
3. Frequency Table:
| Score | Frequency |
|---|---|
| 60 | 1 |
| 70 | 3 |
| 80 | 6 |
| 90 | 4 |
| 100 | 2 |
4. Sum of Data Values: (601) + (703) + (806) + (904) + (100*2) = 770
5. Total Number of Data Points: 1 + 3 + 6 + 4 + 2 = 16
6. Mean: 770 / 16 = 48.125
Therefore, the average test score is approximately 48.125.
Dealing with Larger Datasets and Outliers
While the manual method is suitable for smaller datasets, larger datasets necessitate a more efficient approach. Spreadsheets or statistical software can significantly streamline the calculation process. Simply input the data values and their frequencies, and the software will automatically compute the mean.
Outliers and their Impact:
Outliers, data points significantly different from the rest of the dataset, can significantly influence the mean. While the mean provides a useful measure of central tendency, it's crucial to be aware of the potential impact of outliers. In cases with significant outliers, consider using other measures of central tendency, such as the median (the middle value when data is ordered), which is less sensitive to extreme values. Analyzing the data visually through the dot plot helps in identifying potential outliers before calculating the mean.
Interpreting the Mean in the Context of a Dot Plot
Once you've calculated the mean, it's important to interpret it within the context of the dot plot's visual representation. Does the mean fall near the center of the data distribution, indicating a symmetrical distribution? Or does it lie significantly to one side, suggesting a skewed distribution? Observing the position of the mean relative to the data points helps understand the data's overall tendency and potential skewness.
Advanced Applications and Considerations
Understanding how to calculate the mean from a dot plot is a fundamental statistical skill. This knowledge extends to various applications:
-
Quality Control: In manufacturing, dot plots can visualize the distribution of product measurements, and the mean helps determine if the production process is consistent and meeting quality standards.
-
Environmental Science: Dot plots can represent data on pollution levels, rainfall amounts, or other environmental variables. The mean provides a summary measure for comparison across different locations or time periods.
-
Education: As demonstrated earlier, dot plots and the mean are useful in analyzing student performance on tests or assignments.
The ability to analyze and interpret data visually is crucial in various fields. Mastering the calculation of the mean from a dot plot, coupled with visual inspection of the distribution, provides a solid foundation for making informed decisions based on data. Remember to always consider the context of the data and the potential influence of outliers when interpreting the mean.
Latest Posts
Latest Posts
-
1 1 2 Cup Of Water
Apr 04, 2025
-
How Much Is 10 Fl Oz
Apr 04, 2025
-
12 Ounces Of Water Is How Many Cups
Apr 04, 2025
-
Is Food Coloring In Water A Chemical Change
Apr 04, 2025
-
What Percentage Is 40 Out Of 50
Apr 04, 2025
Related Post
Thank you for visiting our website which covers about How Do You Find The Mean On A Dot Plot . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.
