How Do You Turn A Division Problem Into A Fraction
Kalali
Mar 27, 2025 · 5 min read
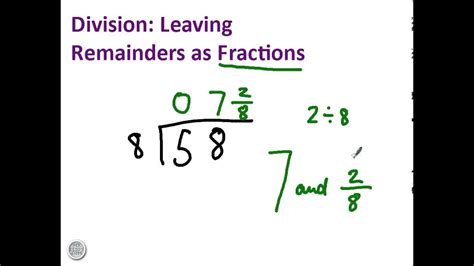
Table of Contents
Turning Division Problems into Fractions: A Comprehensive Guide
Understanding the relationship between division and fractions is fundamental to mastering arithmetic. Many find the transition from division problems to fractional representation challenging, but with a clear understanding of the underlying concepts, it becomes a straightforward process. This comprehensive guide will delve into the intricacies of this conversion, providing you with practical examples and strategies to confidently tackle any division problem and express it as a fraction.
The Inherent Connection Between Division and Fractions
At its core, division is the process of splitting a whole into equal parts. A fraction, similarly, represents a portion of a whole. This inherent similarity allows us to seamlessly translate division problems into fractional notation. The dividend (the number being divided) becomes the numerator (the top number of the fraction), and the divisor (the number we are dividing by) becomes the denominator (the bottom number of the fraction).
Example: Understanding the Basic Conversion
Let's consider a simple division problem: 6 ÷ 2. This means we're dividing 6 into 2 equal parts. To express this as a fraction, we write the dividend (6) as the numerator and the divisor (2) as the denominator: 6/2. This fraction simplifies to 3, confirming that 6 divided by 2 is 3.
Different Types of Division Problems and Their Fractional Equivalents
Division problems can appear in various forms, each requiring a slightly different approach to conversion into fractions. Let's explore these variations:
1. Whole Number Divided by a Whole Number
This is the simplest form. We directly place the dividend as the numerator and the divisor as the denominator.
Example: 15 ÷ 3 = 15/3 = 5
Example: 20 ÷ 5 = 20/5 = 4
This type highlights the fundamental relationship: division is essentially the same operation as finding the value of a fraction.
2. Decimal Divided by a Whole Number
When dealing with decimals in the dividend, the conversion to a fraction is slightly more involved, but follows the same basic principle.
Example: 2.5 ÷ 5
First, we convert the decimal to a fraction: 2.5 can be written as 25/10. Then we perform the division as we would with whole numbers: (25/10) ÷ 5. Remember that dividing by a number is the same as multiplying by its reciprocal. Therefore, we get (25/10) * (1/5) = 25/50 = 1/2. This demonstrates that 2.5 divided by 5 is equal to 1/2 or 0.5.
Example: 3.75 ÷ 2
Convert 3.75 to a fraction: 375/100. Then: (375/100) ÷ 2 = (375/100) * (1/2) = 375/200. This simplifies to 15/8 or 1 and 7/8.
3. Whole Number Divided by a Decimal
This scenario also requires a preliminary step. We must convert the decimal divisor into a whole number by multiplying both the dividend and divisor by a power of 10. This does not change the value of the division.
Example: 10 ÷ 0.2
Multiply both numbers by 10 to get rid of the decimal: (10 * 10) ÷ (0.2 * 10) = 100 ÷ 2. Now we can express this as a fraction: 100/2 = 50.
Example: 15 ÷ 0.75
Multiply both numbers by 100 to eliminate the decimal: (15 * 100) ÷ (0.75 * 100) = 1500 ÷ 75. This translates to the fraction 1500/75, which simplifies to 20.
4. Decimal Divided by a Decimal
This involves two steps: converting both the dividend and divisor into whole numbers and then expressing the result as a fraction.
Example: 1.25 ÷ 0.5
Multiply both by 10: (1.25 * 10) ÷ (0.5 * 10) = 12.5 ÷ 5. Now convert 12.5 to a fraction (125/10). Then we have (125/10) ÷ 5 = (125/10) * (1/5) = 125/50 = 5/2 = 2.5
Example: 3.75 ÷ 1.25
Multiply both numbers by 100: (3.75 * 100) ÷ (1.25 * 100) = 375 ÷ 125. As a fraction this is 375/125, which simplifies to 3.
Simplifying Fractions After Conversion
Once the division problem is converted to a fraction, the next step often involves simplifying the fraction to its lowest terms. This is achieved by finding the greatest common divisor (GCD) of the numerator and denominator and dividing both by it.
Example: 12/18
The GCD of 12 and 18 is 6. Dividing both the numerator and denominator by 6 gives us 2/3.
Example: 25/100
The GCD of 25 and 100 is 25. Dividing both by 25 gives us 1/4.
Finding the GCD can be done using various methods, including prime factorization or the Euclidean algorithm. For simpler fractions, you might be able to identify the GCD by inspection.
Practical Applications and Real-World Examples
The ability to convert division problems into fractions is invaluable in various real-world scenarios. Consider these examples:
-
Baking: A recipe calls for 2 cups of flour, and you want to make half the recipe. Dividing 2 by 2 gives you 1, which can be expressed as 1/2 of the original amount.
-
Sharing: You have 15 candies to share equally among 5 friends. Dividing 15 by 5 gives you 3 candies per friend, expressible as 15/5 = 3 candies per person.
-
Measurement: You have a 10-meter long rope and want to cut it into pieces of 2.5 meters each. Dividing 10 by 2.5 will give you 4 pieces, which can be represented as the fraction 10/2.5 = 4.
Advanced Scenarios and Problem Solving
While the core concept remains consistent, more complex division problems may necessitate a multi-step approach, but they follow the same rules:
-
Dividing Fractions by Fractions: This involves multiplying the dividend fraction by the reciprocal (inverse) of the divisor fraction. The process of conversion to a fraction helps visualize and simplify this multiplication.
-
Dividing Fractions by Whole Numbers or Decimals: Convert the whole number or decimal into a fraction before applying the reciprocal and multiplying.
-
Dividing Complex Fractions: Simplify each complex fraction into a single fraction before performing the division and converting the result into a fraction if needed.
Conclusion
Converting division problems to fractions is a fundamental skill in mathematics with numerous real-world applications. By understanding the inherent relationship between division and fractions, and by following the systematic steps outlined above, you can confidently tackle any division problem and represent it accurately as a fraction. Remember to simplify the resulting fraction whenever possible to ensure clarity and efficiency. Mastering this conversion solidifies your understanding of mathematical operations and opens up new possibilities for problem-solving across various domains. Consistent practice and attention to detail will reinforce your ability to work with both division and fractions seamlessly.
Latest Posts
Latest Posts
-
How Many Liters Is 100 Ml
Mar 30, 2025
-
1 In 25 As A Percentage
Mar 30, 2025
-
For Each Action There Is A Reaction
Mar 30, 2025
-
Why Do Flowers Contain More Stamen Than Pistils
Mar 30, 2025
-
Why Is The Entropy Change Negative For Ring Closures
Mar 30, 2025
Related Post
Thank you for visiting our website which covers about How Do You Turn A Division Problem Into A Fraction . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.
