How To Convert Rectangular To Polar Equations
Kalali
Apr 02, 2025 · 6 min read
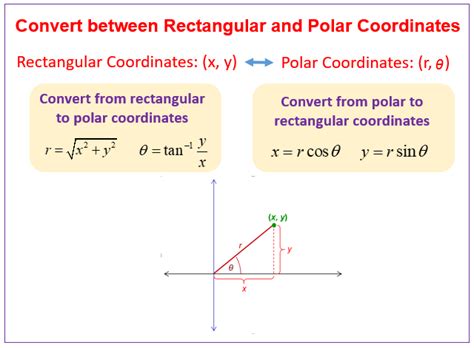
Table of Contents
How to Convert Rectangular to Polar Equations: A Comprehensive Guide
Converting between rectangular (Cartesian) and polar coordinate systems is a fundamental skill in mathematics, particularly in calculus and various branches of physics and engineering. While rectangular coordinates use x and y values to locate a point, polar coordinates use a distance (r) from the origin and an angle (θ) from the positive x-axis. Understanding this conversion is crucial for simplifying complex equations and gaining different perspectives on geometrical problems. This comprehensive guide will break down the process step-by-step, covering various scenarios and providing practical examples.
Understanding Rectangular and Polar Coordinates
Before diving into the conversion process, let's solidify our understanding of the two coordinate systems.
Rectangular Coordinates (Cartesian Coordinates)
Rectangular coordinates represent a point in a two-dimensional plane using two perpendicular axes: the x-axis and the y-axis. A point is located using its horizontal distance (x) from the y-axis and its vertical distance (y) from the x-axis. The coordinates are written as an ordered pair (x, y).
Polar Coordinates
Polar coordinates represent a point using a distance (r) from the origin (also known as the pole) and an angle (θ) measured counterclockwise from the positive x-axis. The angle θ is usually expressed in radians or degrees. The coordinates are written as an ordered pair (r, θ).
The Fundamental Conversion Formulas
The key to converting between these systems lies in the following trigonometric relationships, derived directly from the right-angled triangle formed by the point, the x-axis, and the origin:
- x = r cos θ
- y = r sin θ
- r² = x² + y²
- tan θ = y/x (Note: This formula has limitations, discussed later)
These four equations are the cornerstone of our conversion process. Let's explore how to apply them in practice.
Converting Rectangular Equations to Polar Equations
The process of converting a rectangular equation to a polar equation involves substituting the rectangular coordinates (x and y) with their polar equivalents (r and θ) using the formulas above. The goal is to express the equation solely in terms of r and θ.
Step-by-Step Guide:
-
Identify the Rectangular Equation: Begin with the rectangular equation you wish to convert. This could be a simple linear equation, a circle, a conic section, or a more complex algebraic expression.
-
Substitute x and y: Replace every instance of 'x' with 'r cos θ' and every instance of 'y' with 'r sin θ'.
-
Simplify the Equation: After substitution, simplify the equation algebraically. This often involves trigonometric identities, factoring, and solving for r or θ, depending on the equation's complexity.
-
Express in terms of r and θ: Your final goal is an equation that contains only r and θ. If possible, solve for r as a function of θ (r = f(θ)), as this form is often preferred.
Examples:
Let's illustrate this process with several examples of increasing complexity.
Example 1: Converting a Circle
Consider the equation of a circle with a radius of 5 centered at the origin: x² + y² = 25
-
Substitute: Using r² = x² + y², we can directly substitute: r² = 25
-
Simplify: This equation is already simplified and expressed solely in polar coordinates. Therefore, the polar equation of this circle is simply r = 5.
Example 2: Converting a Line
Let's convert the rectangular equation x = 3:
-
Substitute: Replace 'x' with 'r cos θ': r cos θ = 3
-
Simplify: Solving for r, we get r = 3 / cos θ = 3 sec θ. This is the polar equation of a vertical line.
Example 3: Converting a More Complex Equation
Consider the rectangular equation x² - y² = 4:
-
Substitute: Substitute x = r cos θ and y = r sin θ: (r cos θ)² - (r sin θ)² = 4
-
Simplify: Expand and simplify: r²(cos²θ - sin²θ) = 4. Using the trigonometric identity cos(2θ) = cos²θ - sin²θ, we can further simplify: r² cos(2θ) = 4. Solving for r, we get r² = 4 / cos(2θ) = 4 sec(2θ).
Example 4: Handling Asymptotes and Undefined Values:
Some equations might lead to undefined values for certain angles. Consider the equation x = 0 (the y-axis). Substituting gives r cos θ = 0. This implies either r = 0 or cos θ = 0. Cos θ = 0 when θ = π/2 and θ = 3π/2. This indicates that the equation represents the line θ = π/2 (the y-axis), where it is undefined for r.
Dealing with the Ambiguity of tan θ = y/x
Remember the formula tan θ = y/x? This formula presents a challenge because the tangent function has a period of π. This means that tan θ = tan(θ + nπ) for any integer n. Consequently, using this formula alone to determine θ can lead to multiple possible angles.
To resolve this ambiguity, you must consider the signs of x and y to determine the correct quadrant for the angle θ. For instance:
- If x > 0 and y > 0, θ is in the first quadrant.
- If x < 0 and y > 0, θ is in the second quadrant.
- If x < 0 and y < 0, θ is in the third quadrant.
- If x > 0 and y < 0, θ is in the fourth quadrant.
Converting Polar Equations to Rectangular Equations
This process involves the reverse steps, utilizing the same fundamental formulas but manipulating them to eliminate r and θ, expressing the equation solely in terms of x and y.
-
Identify the Polar Equation: Start with the polar equation you want to convert.
-
Use the Conversion Formulas: Substitute r² = x² + y², x = r cos θ, and y = r sin θ. Often, you'll need to use trigonometric identities to simplify expressions involving r and θ.
-
Simplify and Solve for y (or x): Manipulate the equation algebraically to eliminate r and θ, resulting in an equation containing only x and y.
Examples:
Example 1: Converting a Circle (Again)
Let's convert the polar equation r = 5 back to rectangular coordinates.
-
Substitute: Since r² = x² + y², we have (x² + y²) = 5²
-
Simplify: This simplifies directly to the rectangular equation x² + y² = 25, which represents a circle of radius 5 centered at the origin.
Example 2: Converting a Spiral
Consider the polar equation r = θ. This represents a spiral. Converting this to rectangular coordinates is more challenging:
-
Substitute: We have r² = x² + y². Also, since x = r cos θ, and r = θ, we can write x = θ cos θ. Similarly, y = θ sin θ.
-
Simplify: It is difficult to eliminate θ completely and obtain a concise rectangular representation. Equations like these highlight the advantage of representing certain curves using polar coordinates.
Advanced Techniques and Considerations
For more complex equations, you may need to employ advanced trigonometric identities, algebraic manipulation techniques, and a deep understanding of the relationships between different coordinate systems. Furthermore, understanding the domain and range of the functions involved is crucial for ensuring accuracy.
Some equations might require careful consideration of the specific ranges of θ to prevent inconsistencies. For instance, if an equation involves the inverse trigonometric function, you need to ensure you're working within the correct principal branch to avoid multiple possible solutions.
Conclusion
Converting between rectangular and polar equations is a powerful tool for solving mathematical and scientific problems. Mastering this skill allows you to choose the most convenient coordinate system to represent and solve equations, providing a more efficient and insightful approach to a vast array of geometric and algebraic challenges. By understanding the fundamental conversion formulas and their applications, you can confidently navigate the world of coordinate systems and enhance your mathematical proficiency. Remember to always check your work by plotting the original and converted equations to confirm the equivalence visually. The practice of conversion, particularly working through a diversity of equation types, will enhance your understanding and skills over time.
Latest Posts
Latest Posts
-
How Many Months Is A Hundred Days
Jul 18, 2025
-
Mother And I Or Mother And Me
Jul 18, 2025
-
How Many Oz In One Water Bottle
Jul 18, 2025
-
How Many Dimes In A 5 Roll
Jul 18, 2025
-
How Do You Say Basil In Spanish
Jul 18, 2025
Related Post
Thank you for visiting our website which covers about How To Convert Rectangular To Polar Equations . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.