How To Find X And Y Intercepts In Vertex Form
Kalali
Apr 01, 2025 · 5 min read
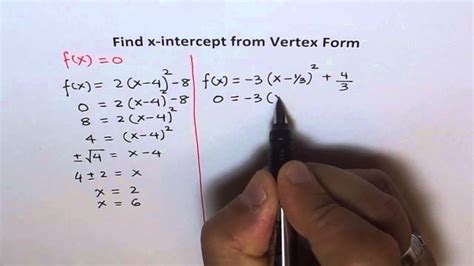
Table of Contents
How to Find X and Y Intercepts in Vertex Form
Finding the x and y intercepts of a quadratic function is a fundamental skill in algebra. These intercepts represent key points on the parabola's graph, providing valuable information about its behavior and characteristics. While various forms of quadratic equations exist, understanding how to extract these intercepts from the vertex form offers unique advantages. This comprehensive guide will delve into the methods, explain the underlying principles, and provide illustrative examples to solidify your understanding. We'll also explore how to handle different scenarios, including those with no x-intercepts.
Understanding Vertex Form
Before we dive into finding intercepts, let's refresh our understanding of the vertex form of a quadratic equation:
y = a(x - h)² + k
Where:
- a: Determines the parabola's vertical stretch or compression and its direction (opening upwards if a > 0, downwards if a < 0).
- (h, k): Represents the coordinates of the vertex (the parabola's highest or lowest point).
This form offers a straightforward way to identify the vertex, which serves as a crucial starting point for finding intercepts.
Finding the Y-Intercept
The y-intercept is the point where the parabola intersects the y-axis. This occurs when the x-coordinate is 0. Therefore, to find the y-intercept, we simply substitute x = 0 into the vertex form equation:
y = a(0 - h)² + k
Simplifying this gives us:
y = a(-h)² + k = ah² + k
Therefore, the y-intercept is the point (0, ah² + k). This is a simple calculation once you know the values of a, h, and k.
Finding the X-Intercept(s)
The x-intercept(s) are the point(s) where the parabola intersects the x-axis. At these points, the y-coordinate is 0. To find the x-intercepts, we set y = 0 in the vertex form equation and solve for x:
0 = a(x - h)² + k
Now, let's solve this equation step-by-step:
-
Isolate the squared term: Subtract k from both sides: -k = a(x - h)²
-
Divide by a: (Assuming a ≠ 0; if a = 0, the equation is not quadratic) -k/a = (x - h)²
-
Take the square root of both sides: Remember to consider both positive and negative roots: ±√(-k/a) = x - h
-
Solve for x: Add h to both sides: x = h ± √(-k/a)
This gives us two possible values for x, representing the x-coordinates of the x-intercepts. Therefore, the x-intercepts are (h + √(-k/a), 0) and (h - √(-k/a), 0).
Important Considerations for X-Intercepts:
-
The discriminant (-k/a): The expression inside the square root, -k/a, is crucial. It determines the number of x-intercepts:
- -k/a > 0: The parabola has two distinct x-intercepts (it crosses the x-axis at two points).
- -k/a = 0: The parabola has one x-intercept (it touches the x-axis at the vertex). This occurs when the vertex lies on the x-axis.
- -k/a < 0: The parabola has no x-intercepts (it does not cross the x-axis). This means the parabola lies entirely above or below the x-axis.
-
Imaginary roots: If -k/a is negative, the square root results in imaginary numbers. This indicates that there are no real x-intercepts, meaning the parabola doesn't intersect the x-axis in the real number plane.
Illustrative Examples
Let's solidify our understanding with some examples:
Example 1: Two distinct x-intercepts
Find the x and y intercepts of the quadratic function y = 2(x - 3)² - 8.
Here, a = 2, h = 3, and k = -8.
Y-intercept: y = 2(3)² - 8 = 18 - 8 = 10. The y-intercept is (0, 10).
X-intercepts: -k/a = -(-8)/2 = 4 x = 3 ± √4 = 3 ± 2 x₁ = 5; x₂ = 1 The x-intercepts are (5, 0) and (1, 0).
Example 2: One x-intercept
Find the x and y intercepts of the quadratic function y = -1(x + 1)² + 0.
Here, a = -1, h = -1, and k = 0.
Y-intercept: y = -1(-1)² + 0 = -1. The y-intercept is (0, -1).
X-intercepts: -k/a = -0/(-1) = 0 x = -1 ± √0 = -1 The x-intercept is (-1, 0).
Example 3: No x-intercepts
Find the x and y intercepts of the quadratic function y = (x - 2)² + 4.
Here, a = 1, h = 2, and k = 4.
Y-intercept: y = (2)² + 4 = 8. The y-intercept is (0, 8).
X-intercepts: -k/a = -4/1 = -4 (Negative, indicating no real x-intercepts).
There are no x-intercepts because the parabola opens upwards and its vertex is above the x-axis.
Advanced Considerations and Applications
Understanding how to find x and y intercepts in vertex form is crucial for various applications, including:
-
Graphing quadratic functions: The intercepts are key points for accurately sketching the parabola. The vertex, combined with the intercepts, provides a complete picture of the graph's shape and position.
-
Solving quadratic word problems: Many real-world problems involving projectile motion, optimization, and area calculations can be modeled using quadratic equations. Finding the intercepts helps understand the solutions within the context of the problem. For instance, in projectile motion, the x-intercepts represent the points where the object hits the ground.
-
Analyzing the behavior of quadratic functions: The intercepts reveal information about the function's range and domain. The y-intercept indicates the initial value, while the x-intercepts indicate where the function's value is zero.
-
Using technology to verify solutions: Graphing calculators and software can be used to plot the parabola and visually confirm the calculated intercepts.
Conclusion
Finding the x and y intercepts of a quadratic function in vertex form is a valuable skill with broad applications. By understanding the underlying principles and following the steps outlined above, you can accurately determine these critical points and use them to gain deeper insights into the behavior and characteristics of quadratic functions. Remember to always check the discriminant (-k/a) to determine the number of x-intercepts, and don't hesitate to use technology to verify your results and enhance your understanding. Mastering this technique will greatly improve your ability to solve quadratic equations and related problems effectively.
Latest Posts
Latest Posts
-
If Your 35 What Year Was You Born
Jul 12, 2025
-
How Many Cups Is 1 Pound Of Cheese
Jul 12, 2025
-
30 X 30 Is How Many Square Feet
Jul 12, 2025
-
How Much Does A Half Oz Weigh
Jul 12, 2025
-
Calories In An Omelette With 3 Eggs
Jul 12, 2025
Related Post
Thank you for visiting our website which covers about How To Find X And Y Intercepts In Vertex Form . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.