Integral Of 1 X 3 1
Kalali
May 19, 2025 · 3 min read
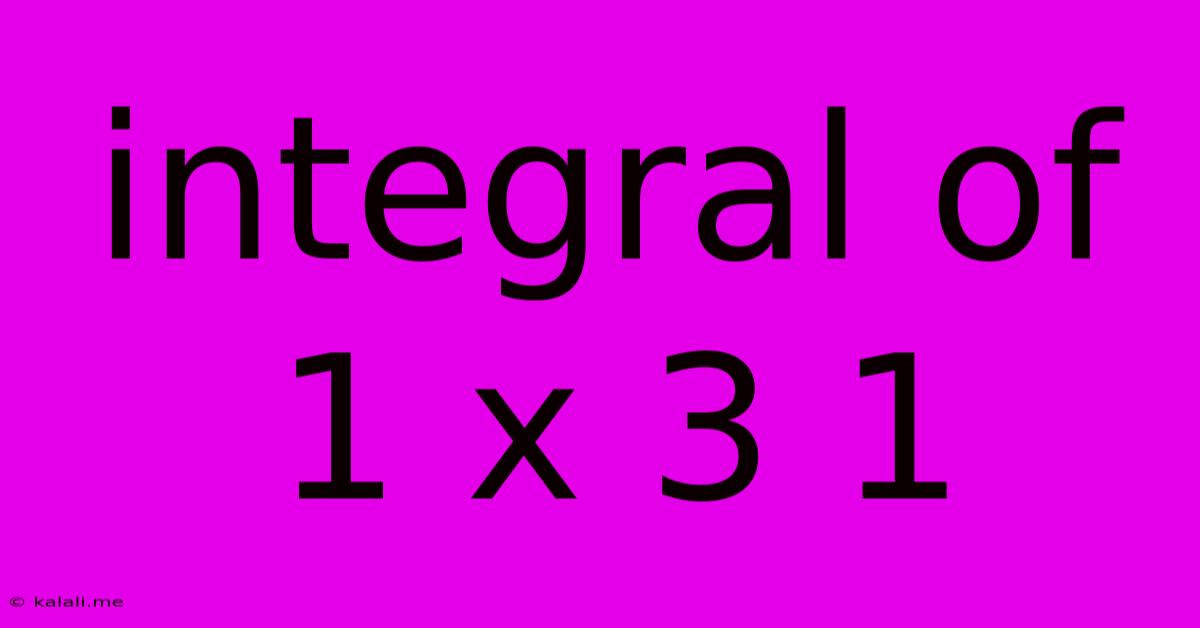
Table of Contents
Decoding the Integral: Understanding ∫1/(x³+1) dx
This article delves into the intricacies of solving the indefinite integral ∫1/(x³+1) dx. This seemingly simple integral presents a fascinating challenge, requiring a blend of algebraic manipulation and integration techniques. Understanding this process will enhance your skills in calculus and improve your ability to tackle similar complex integrals.
The integral ∫1/(x³+1) dx doesn't yield to straightforward integration rules. The key lies in factoring the denominator and applying partial fraction decomposition. Let's break down the steps:
1. Factoring the Denominator (x³ + 1)
The expression x³ + 1 is a sum of cubes, which factors neatly as follows:
x³ + 1 = (x + 1)(x² - x + 1)
This factorization is crucial for applying the partial fraction decomposition method.
2. Partial Fraction Decomposition
Now, we rewrite the integrand 1/(x³+1) as a sum of simpler fractions:
1/(x³ + 1) = A/(x + 1) + (Bx + C)/(x² - x + 1)
where A, B, and C are constants we need to determine.
To find A, B, and C, we multiply both sides by (x³ + 1):
1 = A(x² - x + 1) + (Bx + C)(x + 1)
We can solve for A, B, and C using several methods. One common approach is to substitute specific values of x:
- Let x = -1: This eliminates the (Bx + C) term, leaving 1 = 3A, so A = 1/3.
- Let x = 0: This gives 1 = A + C, and since A = 1/3, we find C = 2/3.
- Let x = 1: This gives 1 = A + 2(B + C), substituting A and C, we get 1 = 1/3 + 2(B + 2/3). Solving for B, we get B = -1/3.
Therefore, our partial fraction decomposition is:
1/(x³ + 1) = (1/3)/(x + 1) + (-x/3 + 2/3)/(x² - x + 1)
3. Integrating the Partial Fractions
Now we integrate each term separately:
∫(1/3)/(x + 1) dx = (1/3)ln|x + 1| + K₁
The second term requires a bit more work. We can rewrite it as:
∫(-x/3 + 2/3)/(x² - x + 1) dx = (-1/6)∫(2x - 2)/(x² - x + 1) dx + (1/2)∫2/(x² - x + 1) dx
The first integral in this expression is a simple u-substitution (u = x² - x + 1). The second integral requires completing the square in the denominator and using a trigonometric substitution or a formula for the integral of 1/(x²+a²).
Completing the square: x² - x + 1 = (x - 1/2)² + 3/4
Using the appropriate integral formula or a trigonometric substitution will resolve this integral into an arctan function.
4. Combining the Results
After integrating both parts and simplifying, the final result will be the sum of the two integrated parts plus a constant of integration. The precise form of this final expression will include logarithmic and arctangent terms.
In summary: Solving ∫1/(x³+1) dx involves a multi-step process combining algebraic manipulation (factoring and partial fraction decomposition), and various integration techniques (u-substitution, completing the square, and possibly trigonometric substitution or formula application for arctan). While the steps are numerous, the careful application of these techniques leads to a complete solution. The final answer will be a combination of logarithmic and arctangent functions. Remember to always check your work and consult integral tables or online calculators for verification.
Latest Posts
Latest Posts
-
My New Cat Is Hiding And Not Eating
May 20, 2025
-
Phrase Meaning Extremely Fast Crossword Clue
May 20, 2025
-
How Long Does Chia Seeds Last
May 20, 2025
-
How To Tell If Mozzarella Is Bad
May 20, 2025
-
Fallout New Vegas Power Armour Training
May 20, 2025
Related Post
Thank you for visiting our website which covers about Integral Of 1 X 3 1 . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.