Integrate 1 1 X 2 1 2
Kalali
May 19, 2025 · 2 min read
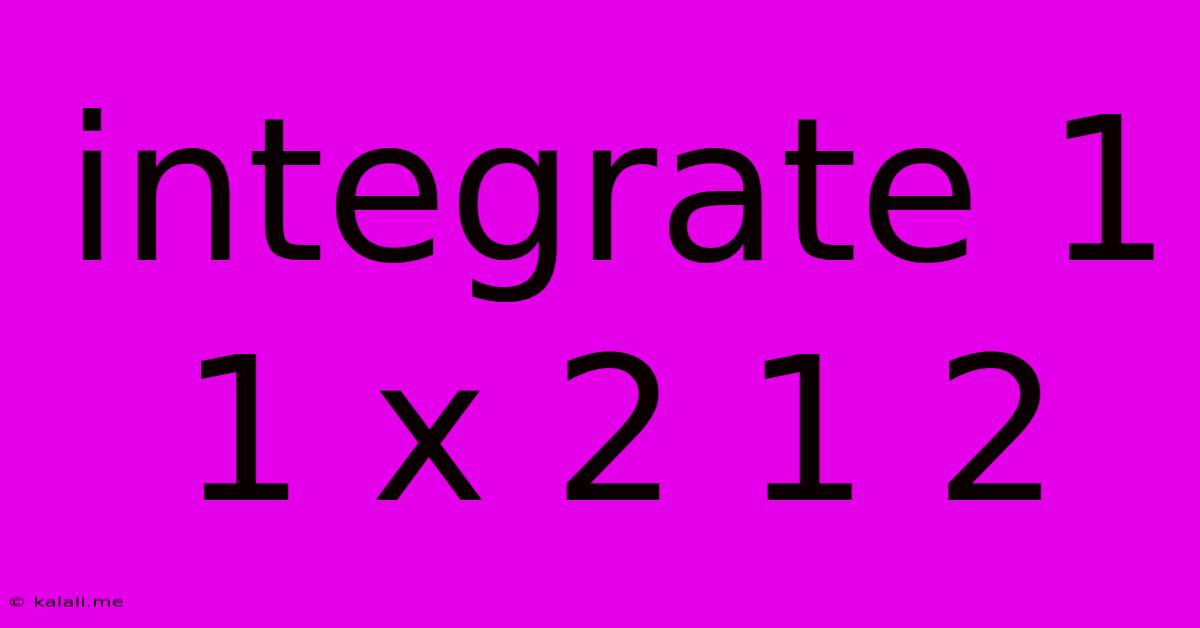
Table of Contents
Integrating 1/(1+x²) from 1 to 2: A Step-by-Step Guide
This article provides a comprehensive guide on how to integrate the function 1/(1+x²) from the limits 1 to 2. This is a common integral encountered in calculus, often related to inverse trigonometric functions. Understanding this integration process is crucial for various applications in mathematics, physics, and engineering.
What is Integration?
Before delving into the specific integration, let's briefly revisit the concept of integration. Integration is essentially the reverse process of differentiation. It finds the area under a curve, represented by a function, within specified limits. This area calculation has numerous practical interpretations, from calculating distances travelled given velocity to determining the work done by a force.
Solving the Integral ∫₁² 1/(1+x²) dx
The integral ∫₁² 1/(1+x²) dx is a standard integral, readily solvable using the knowledge of inverse trigonometric functions. Specifically, the antiderivative (or indefinite integral) of 1/(1+x²) is arctan(x) + C, where C is the constant of integration.
Here's a step-by-step breakdown:
-
Identify the Antiderivative: The crucial step is recognizing that the antiderivative of 1/(1+x²) is arctan(x). This is a fundamental result derived from the differentiation of arctan(x).
-
Apply the Limits of Integration: Once we have the antiderivative, we evaluate it at the upper and lower limits of integration (2 and 1, respectively). This process is represented as:
[arctan(x)]²₁ = arctan(2) - arctan(1)
-
Evaluate the Result: Now, we calculate the numerical values of arctan(2) and arctan(1). While these values are not easily expressed as exact fractions, they can be approximated using a calculator or mathematical software.
arctan(2) ≈ 1.107 radians arctan(1) = π/4 ≈ 0.785 radians
-
Calculate the Definite Integral: Finally, subtract the value of arctan(1) from arctan(2) to obtain the definite integral:
arctan(2) - arctan(1) ≈ 1.107 - 0.785 ≈ 0.322 radians
Therefore, the definite integral of 1/(1+x²) from 1 to 2 is approximately 0.322.
Applications and Further Exploration
This seemingly simple integral has broad applications:
- Probability and Statistics: The integral is related to the probability density function of the Cauchy distribution.
- Physics: It appears in various physics problems involving inverse square laws.
- Engineering: It finds use in signal processing and circuit analysis.
Understanding this integral lays a foundation for tackling more complex integration problems involving similar functions or techniques like substitution and integration by parts. Further exploration could involve investigating the geometric interpretation of the integral as the area under the curve 1/(1+x²) between x=1 and x=2. You can also explore numerical integration methods if analytical solutions are unavailable.
This comprehensive guide should provide a solid understanding of how to integrate 1/(1+x²) from 1 to 2. Remember, practice is key to mastering integration techniques.
Latest Posts
Latest Posts
-
Key Will Not Come Out Of Lock
May 19, 2025
-
How To Get Diesel Smell Out Of Clothes
May 19, 2025
-
How To Say With In German
May 19, 2025
-
How To Stop Creaking Floors Under Carpet
May 19, 2025
-
When Is Beauty And The Beast Set
May 19, 2025
Related Post
Thank you for visiting our website which covers about Integrate 1 1 X 2 1 2 . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.