Integration Of X 1 X 2 1 2
Kalali
May 19, 2025 · 3 min read
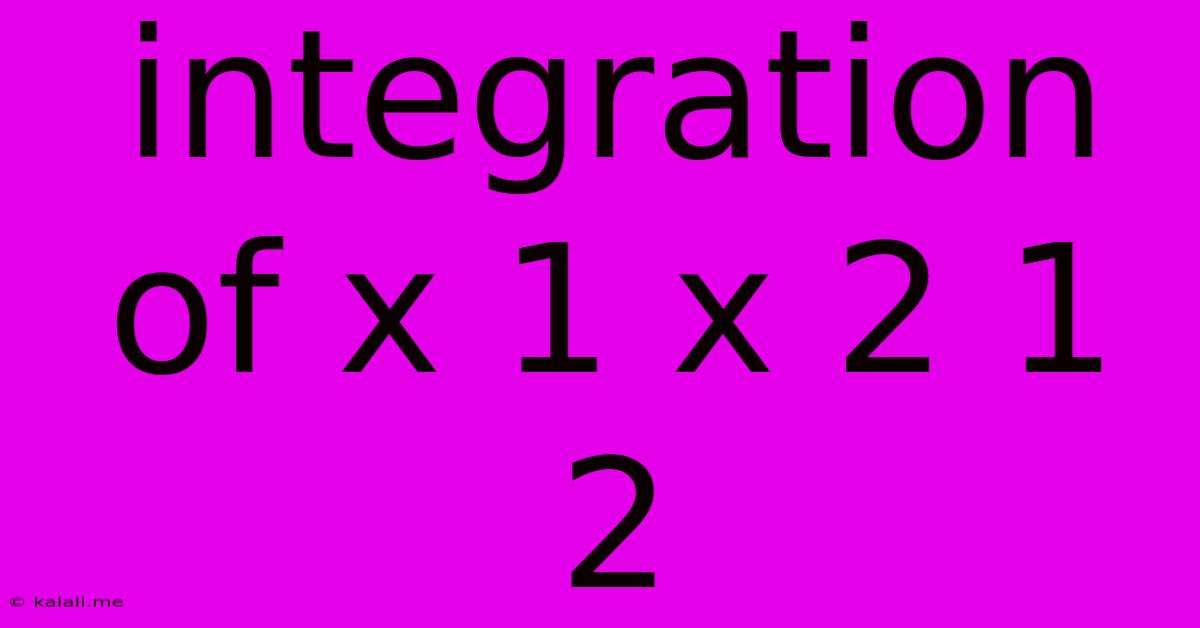
Table of Contents
Mastering the Integration of x<sup>1</sup>, x<sup>2</sup>, 1, and 2: A Comprehensive Guide
This article provides a comprehensive guide to integrating the basic functions x<sup>1</sup>, x<sup>2</sup>, 1, and 2. Understanding these fundamental integrations is crucial for mastering calculus and its applications in various fields, from physics and engineering to economics and finance. We'll cover the integration process, demonstrate examples, and highlight common pitfalls to avoid. By the end, you'll be confident in handling these integral calculations.
Understanding the Basics of Integration
Integration is the reverse process of differentiation. Where differentiation finds the rate of change of a function, integration finds the area under the curve of a function. The indefinite integral adds a constant of integration (+C) because the derivative of a constant is zero. This constant accounts for the family of functions that share the same derivative.
Integrating x<sup>1</sup> (x)
The integral of x<sup>1</sup> (or simply x) is found by applying the power rule of integration:
∫x<sup>n</sup> dx = (x<sup>n+1</sup>)/(n+1) + C
Therefore, the integral of x is:
∫x dx = (x<sup>1+1</sup>)/(1+1) + C = x<sup>2</sup>/2 + C
Example: Find the indefinite integral of 3x.
∫3x dx = 3∫x dx = 3(x<sup>2</sup>/2) + C = (3/2)x<sup>2</sup> + C
Integrating x<sup>2</sup>
Using the same power rule:
∫x<sup>2</sup> dx = (x<sup>2+1</sup>)/(2+1) + C = x<sup>3</sup>/3 + C
Example: Find the indefinite integral of 5x<sup>2</sup> + 2.
∫(5x<sup>2</sup> + 2) dx = 5∫x<sup>2</sup> dx + ∫2 dx = 5(x<sup>3</sup>/3) + 2x + C = (5/3)x<sup>3</sup> + 2x + C
Integrating 1
The integral of 1 (or x<sup>0</sup>) is:
∫1 dx = ∫x<sup>0</sup> dx = (x<sup>0+1</sup>)/(0+1) + C = x + C
This represents the area under a horizontal line at y = 1.
Integrating 2
The integral of 2 (a constant) is:
∫2 dx = 2x + C
This is a simple application of the constant rule of integration: the integral of a constant 'k' is kx + C.
Common Mistakes and How to Avoid Them
- Forgetting the Constant of Integration (+C): This is a crucial step in indefinite integration. Always remember to include the constant of integration.
- Incorrect Application of the Power Rule: Double-check your exponent calculations, especially when dealing with negative or fractional exponents.
- Not Properly Handling Constants: Remember that the integral of a constant multiplied by a function is the constant multiplied by the integral of the function.
Applications and Further Exploration
These basic integrations form the building blocks for more complex integration problems. Mastering them is essential for tackling integrals involving trigonometric functions, exponential functions, and logarithmic functions. Further exploration should involve practicing numerous examples and delving into techniques like integration by substitution and integration by parts. Understanding definite integrals and their application in calculating areas and volumes is also a crucial next step.
This comprehensive guide provides a solid foundation for understanding the integration of x<sup>1</sup>, x<sup>2</sup>, 1, and 2. By practicing these examples and understanding the underlying principles, you'll build a strong base for tackling more advanced integration problems. Remember consistent practice is key to mastering this fundamental concept in calculus.
Latest Posts
Latest Posts
-
Why Did Elliot Stabler Leave Svu
May 19, 2025
-
Do Passport Numbers Change When Renewing
May 19, 2025
-
How Do You Say With In German
May 19, 2025
-
Is It Haram To Watch Porn
May 19, 2025
-
Is Energy A Scalar Or Vector
May 19, 2025
Related Post
Thank you for visiting our website which covers about Integration Of X 1 X 2 1 2 . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.