Is 29 A Prime Number Or A Composite Number
Kalali
Apr 04, 2025 · 5 min read
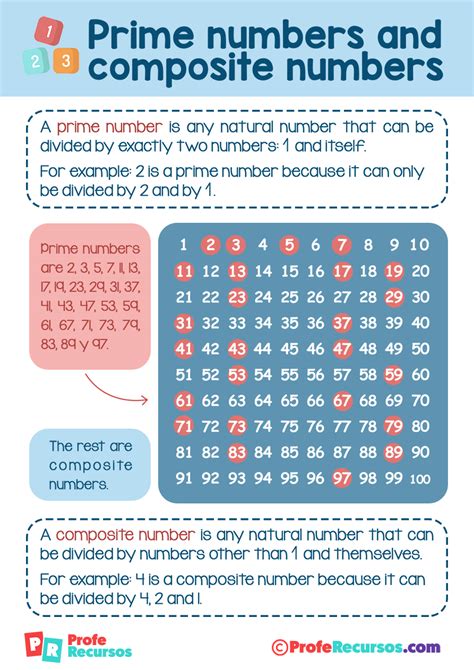
Table of Contents
Is 29 a Prime Number or a Composite Number? A Deep Dive into Prime Numbers and Divisibility
The question, "Is 29 a prime number or a composite number?" might seem simple at first glance. However, understanding the answer requires a deeper understanding of prime and composite numbers, their properties, and the methods used to determine the primality of a number. This article will not only answer the question definitively but also explore the fascinating world of prime numbers and their significance in mathematics.
Understanding Prime and Composite Numbers
Before we delve into the specific case of 29, let's establish a firm understanding of the fundamental definitions:
Prime Number: A prime number is a natural number greater than 1 that has no positive divisors other than 1 and itself. In simpler terms, it's only divisible by 1 and itself without leaving a remainder. Examples include 2, 3, 5, 7, 11, and so on. The number 1 is considered neither prime nor composite.
Composite Number: A composite number is a natural number greater than 1 that is not a prime number. This means it has at least one positive divisor other than 1 and itself. Examples include 4 (2 x 2), 6 (2 x 3), 9 (3 x 3), and so on.
The Fundamental Theorem of Arithmetic: This theorem states that every integer greater than 1 can be uniquely represented as a product of prime numbers, disregarding the order of the factors. This is a cornerstone of number theory, highlighting the fundamental importance of prime numbers in the structure of integers.
Determining if 29 is Prime or Composite
Now, let's address the question directly: Is 29 a prime number or a composite number?
To determine this, we need to check if 29 has any divisors other than 1 and itself. We can do this by systematically checking for divisibility by prime numbers less than the square root of 29. The square root of 29 is approximately 5.38. Therefore, we only need to check divisibility by the prime numbers 2, 3, and 5.
- Divisibility by 2: 29 is not divisible by 2 because it's an odd number.
- Divisibility by 3: The sum of the digits of 29 is 2 + 9 = 11, which is not divisible by 3. Therefore, 29 is not divisible by 3. (A number is divisible by 3 if the sum of its digits is divisible by 3).
- Divisibility by 5: 29 does not end in 0 or 5, so it's not divisible by 5.
Since 29 is not divisible by 2, 3, or 5 (the prime numbers less than its square root), it has no divisors other than 1 and itself. Therefore, 29 is a prime number.
Exploring the Properties of Prime Numbers
Prime numbers possess many interesting properties and have played a crucial role in various areas of mathematics and computer science. Here are some key characteristics:
-
Infinitude of Primes: There are infinitely many prime numbers. This was famously proven by Euclid in his Elements. This means that no matter how large a number you consider, there will always be larger prime numbers.
-
Distribution of Primes: While the distribution of primes seems somewhat random, there are patterns and theorems that describe their asymptotic behavior. The Prime Number Theorem, for example, provides an approximation of the number of primes less than a given number.
-
Prime Factorization: As mentioned earlier, the Fundamental Theorem of Arithmetic highlights the unique prime factorization of every integer greater than 1. This property is fundamental to many cryptographic algorithms.
-
Twin Primes: Twin primes are pairs of prime numbers that differ by 2 (e.g., 3 and 5, 11 and 13, 17 and 19). The twin prime conjecture, which postulates that there are infinitely many twin primes, remains one of the most important unsolved problems in number theory.
-
Mersenne Primes: Mersenne primes are prime numbers that are one less than a power of 2 (e.g., 3, 7, 31, 127). The search for Mersenne primes is a significant area of research, often utilizing distributed computing projects like GIMPS (Great Internet Mersenne Prime Search).
The Significance of Prime Numbers in Cryptography
Prime numbers form the bedrock of many modern cryptographic systems. The security of these systems relies on the difficulty of factoring large composite numbers into their prime factors. Algorithms like RSA (Rivest-Shamir-Adleman) utilize this principle to encrypt and decrypt information securely. The difficulty of factoring large numbers makes it computationally infeasible for attackers to break these cryptographic systems.
Methods for Primality Testing
Determining whether a large number is prime can be computationally intensive. Several algorithms have been developed to efficiently test for primality, including:
-
Trial Division: This is a straightforward method, but it becomes computationally expensive for very large numbers.
-
Probabilistic Primality Tests: These tests, such as the Miller-Rabin test, provide a probabilistic answer. They don't guarantee primality but offer a high probability of correctness.
-
Deterministic Primality Tests: These tests, like the AKS primality test, guarantee primality but can be slower than probabilistic tests for extremely large numbers.
Conclusion: 29's Prime Status and Beyond
To reiterate, 29 is indeed a prime number. Its primality is easily established by checking for divisibility by prime numbers less than its square root. This seemingly simple question opens a door to a vast and fascinating field of mathematics – the study of prime numbers. Their unique properties and significance in various applications, from fundamental theorems to modern cryptography, make them a constant source of mathematical inquiry and technological innovation. The ongoing research into prime numbers continues to push the boundaries of our understanding of numbers and their profound influence on our digital world. The seemingly simple question of whether 29 is prime serves as a compelling introduction to the rich and complex world of number theory.
Latest Posts
Latest Posts
-
Words That Have K In The Middle
Apr 04, 2025
-
How Much Is 2 1 4 Cups
Apr 04, 2025
-
How Many Neutrons Are In An Atom Of Mg 25
Apr 04, 2025
-
How Many Angles Does Triangle Have
Apr 04, 2025
-
Write A Linear Function X With The Given Values
Apr 04, 2025
Related Post
Thank you for visiting our website which covers about Is 29 A Prime Number Or A Composite Number . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.
