Maclaurin Series For 1 1 X
Kalali
May 22, 2025 · 2 min read
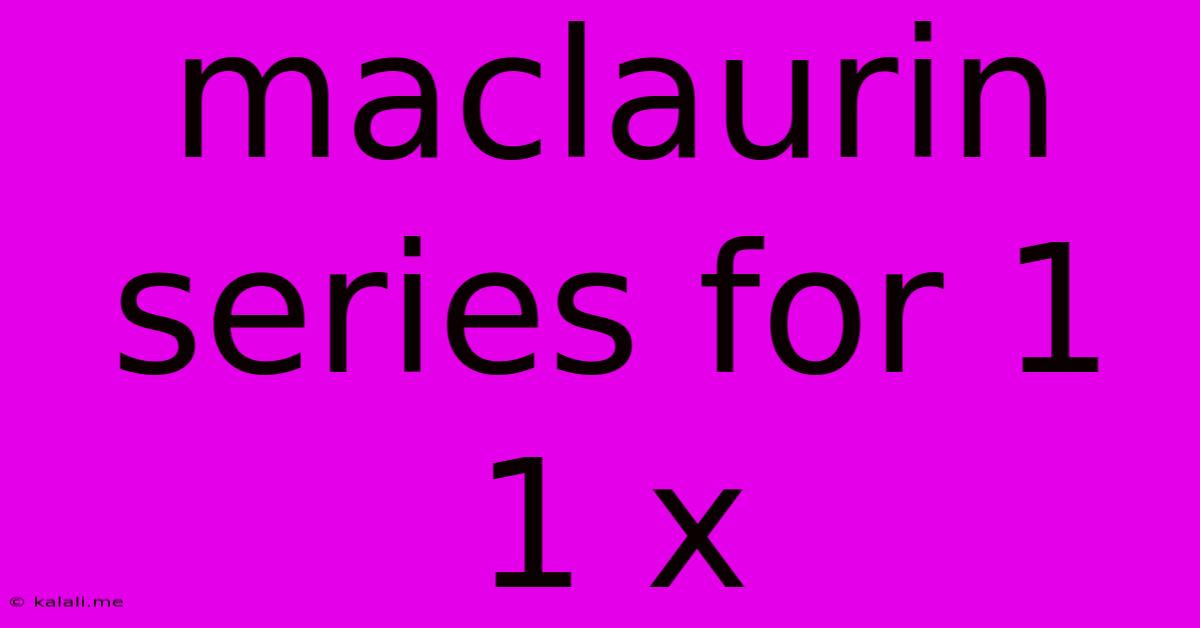
Table of Contents
Maclaurin Series for 1/(1+x)
The Maclaurin series is a powerful tool for approximating the value of a function using an infinite sum of terms. It's a special case of the Taylor series, centered at x=0. This article will delve into deriving and understanding the Maclaurin series for the function f(x) = 1/(1+x), exploring its convergence and applications. Understanding this series is fundamental to many areas of mathematics, including calculus and complex analysis.
Understanding the Maclaurin Series
The Maclaurin series of a function f(x) is given by:
f(x) = f(0) + f'(0)x + (f''(0)x²)/2! + (f'''(0)x³)/3! + ... = Σ (f⁽ⁿ⁾(0)xⁿ)/n!
where:
- f⁽ⁿ⁾(0) represents the nth derivative of f(x) evaluated at x = 0.
- n! is the factorial of n.
- The summation (Σ) runs from n = 0 to infinity.
Deriving the Maclaurin Series for 1/(1+x)
Let's find the Maclaurin series for f(x) = 1/(1+x). We need to find the derivatives of f(x) and evaluate them at x=0:
- f(x) = (1+x)⁻¹
- f(0) = 1
- f'(x) = -(1+x)⁻²
- f'(0) = -1
- f''(x) = 2(1+x)⁻³
- f''(0) = 2
- f'''(x) = -6(1+x)⁻⁴
- f'''(0) = -6
- and so on...
Notice a pattern emerging: the nth derivative evaluated at x=0 is (-1)ⁿ * n!.
Substituting these values into the Maclaurin series formula, we get:
1/(1+x) = 1 - x + x² - x³ + x⁴ - ... = Σ (-1)ⁿxⁿ
This series is valid for |x| < 1. Outside this interval, the series diverges.
Convergence and Radius of Convergence
The series converges for |x| < 1, which is its radius of convergence. At x = 1, the series becomes the alternating harmonic series, which converges conditionally. At x = -1, the series diverges. Understanding the interval of convergence is crucial for accurately applying the Maclaurin series.
Applications of the Maclaurin Series for 1/(1+x)
This seemingly simple series has surprisingly broad applications:
- Approximating values: For values of x close to 0, the series provides a good approximation of 1/(1+x). The more terms included, the more accurate the approximation becomes.
- Solving differential equations: The series can be used to find power series solutions to certain differential equations.
- Integration: Integrating the Maclaurin series term-by-term can be easier than integrating the original function, particularly for functions without elementary antiderivatives.
- Generating Functions: In combinatorics and probability, this series plays a key role as a generating function for certain sequences.
Conclusion
The Maclaurin series for 1/(1+x) is a fundamental tool in mathematics. Its derivation, convergence properties, and wide array of applications highlight its significance. Mastering this series provides a strong foundation for understanding more advanced concepts in calculus and its applications across various scientific fields. Remember to always consider the radius of convergence when utilizing this series for approximations or calculations.
Latest Posts
Latest Posts
-
Not Getting Paid For Training Is Illegal Gov Uk
May 22, 2025
-
How To Get Rid Of Ice In Freezer
May 22, 2025
-
How To Assign Workers In Fallout 4
May 22, 2025
-
How To Delete Cloud Saves On Steam
May 22, 2025
-
How To Find Out Your Facebook Email
May 22, 2025
Related Post
Thank you for visiting our website which covers about Maclaurin Series For 1 1 X . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.