Radius Is Half Of The Diameter
Kalali
Apr 05, 2025 · 5 min read
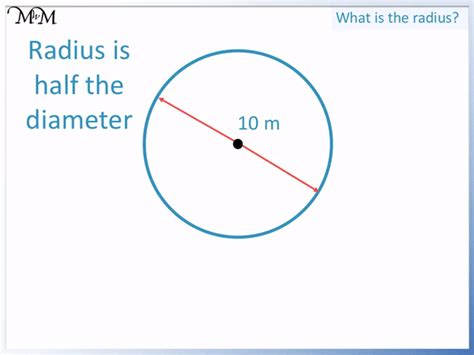
Table of Contents
Radius is Half the Diameter: A Deep Dive into Circles and Their Properties
Understanding the fundamental concepts of circles is crucial in various fields, from mathematics and engineering to design and everyday life. One of the most basic yet essential relationships within a circle is the connection between its radius and diameter. This article will explore this relationship in detail, examining its implications and applications across diverse areas. We will delve into the mathematical definition, practical examples, and even touch upon the historical significance of this simple yet powerful concept.
Defining Radius and Diameter
Before we delve into the relationship, let's clearly define each term:
Radius: The radius of a circle is the distance from the center of the circle to any point on its circumference. It's a straight line segment extending from the heart of the circle to its edge. Think of it as the "arm" of the circle. We commonly denote the radius with the lowercase letter 'r'.
Diameter: The diameter of a circle is the longest chord (a straight line segment whose endpoints lie on the circumference) that passes through the center of the circle. It essentially cuts the circle precisely in half. The diameter is twice the length of the radius. We usually represent the diameter with the lowercase letter 'd'.
The Fundamental Relationship: Radius is Half the Diameter
The core relationship between the radius and diameter is elegantly simple: the radius is exactly half the length of the diameter. Mathematically, this can be expressed as:
r = d/2 or equivalently, d = 2r
This formula is fundamental to all calculations involving circles. Knowing either the radius or the diameter allows for the immediate calculation of the other. This seemingly trivial relationship forms the bedrock for understanding more complex circular properties and calculations.
Applications in Various Fields
The relationship between radius and diameter isn't just a mathematical curiosity; it has widespread applications across numerous disciplines:
1. Engineering and Construction
In engineering and construction, understanding the radius and diameter is crucial for designing circular structures like:
-
Pipelines: The diameter of a pipeline dictates its capacity to transport fluids. The radius, in turn, influences the internal pressure and structural integrity of the pipe.
-
Wheels and Gears: The radius and diameter of wheels and gears determine their rotational speed, torque, and overall functionality in machinery. Designing these components accurately requires a precise understanding of these measurements.
-
Circular Buildings and Structures: The radius of a dome or a circular building dictates its size, structural stability, and even the amount of materials required for construction.
2. Manufacturing and Design
Manufacturing processes frequently rely on accurate measurements of radius and diameter:
-
Manufacturing Circular Parts: Precise control over radius and diameter is essential in producing cylindrical components, bearings, and other circular machine parts. Inaccurate measurements can lead to malfunctioning machinery or even safety hazards.
-
Packaging and Design: Circular containers and packaging designs often require specific radii and diameters to meet volume requirements and optimize product presentation.
3. Mapping and Navigation
Radius and diameter play a role in:
-
Cartography: Understanding the radius of the Earth is crucial for accurate map projections and geographical calculations.
-
Navigation Systems: GPS systems utilize spherical coordinates, which involve radius and latitude/longitude measurements to determine locations precisely.
4. Astronomy
Radius and diameter are fundamental concepts in astronomy:
-
Celestial Bodies: The radius and diameter of planets, stars, and other celestial bodies help astronomers understand their size, mass, and other physical properties.
-
Orbital Calculations: Orbital calculations involving planets and satellites often use radii and diameters to determine distances and trajectories.
Beyond Basic Calculations: Exploring Circumference and Area
The radius-diameter relationship is not just useful for determining each other's values; it's also an integral part of calculating other important properties of a circle, namely its circumference and area.
Calculating Circumference
The circumference of a circle is the distance around its edge. The formula for circumference (C) involves the radius (or diameter) and the mathematical constant π (pi), approximately equal to 3.14159:
- C = 2πr (using the radius)
- C = πd (using the diameter)
Knowing the relationship between radius and diameter allows you to easily switch between these two formulas depending on which measurement you are given.
Calculating Area
The area (A) of a circle, representing the space enclosed within its circumference, is also calculated using the radius:
A = πr²
Although this formula doesn't directly use the diameter, we can substitute the radius-diameter relationship (r = d/2) to express the area in terms of the diameter:
A = π(d/2)² = πd²/4
Practical Examples
Let's illustrate the applications with some examples:
Example 1: A circular garden has a diameter of 10 meters. What is its radius and area?
- Radius: r = d/2 = 10m / 2 = 5m
- Area: A = πr² = π(5m)² ≈ 78.54 square meters
Example 2: A cylindrical pipe has a radius of 2 inches. What is its diameter and circumference?
- Diameter: d = 2r = 2 * 2 inches = 4 inches
- Circumference: C = 2πr = 2π(2 inches) ≈ 12.57 inches
Example 3: A pizza has a radius of 7 inches. What is its area?
- Area: A = πr² = π(7 inches)² ≈ 153.94 square inches
The Historical Significance of π (Pi)
The relationship between the radius, diameter, and circumference of a circle is intrinsically linked to the mathematical constant π (pi). The discovery and approximation of π have been a significant endeavor throughout history, dating back to ancient civilizations. Early mathematicians, through meticulous measurements and calculations, realized that the ratio of a circle's circumference to its diameter was always a constant value, later denoted as π. This constant, approximately 3.14159, is irrational, meaning its decimal representation goes on forever without repeating. The pursuit of a more accurate value of π has driven mathematical advancements and continues to be a source of fascination.
Conclusion: A Foundation for Understanding Circles
The simple yet profound relationship between the radius and diameter of a circle – radius equals half the diameter – serves as a cornerstone for understanding circular geometry. This fundamental concept extends far beyond basic mathematical calculations, finding applications in diverse fields, from engineering and design to astronomy and cartography. Understanding this relationship is essential for solving various problems and gaining a deeper appreciation for the elegance and practicality of circular geometry. By grasping this core principle, we unlock a world of possibilities in understanding and manipulating circular forms in the world around us.
Latest Posts
Latest Posts
-
How Many Cm In 73 Inches
Apr 05, 2025
-
What Are The Factors Of 49
Apr 05, 2025
-
Convertir Metros A Pies Y Pulgadas
Apr 05, 2025
-
60 Is What Percent Of 30
Apr 05, 2025
-
3 Out Of 20 As A Percentage
Apr 05, 2025
Related Post
Thank you for visiting our website which covers about Radius Is Half Of The Diameter . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.
