What Is 1 8th Of 100
Kalali
Apr 25, 2025 · 4 min read
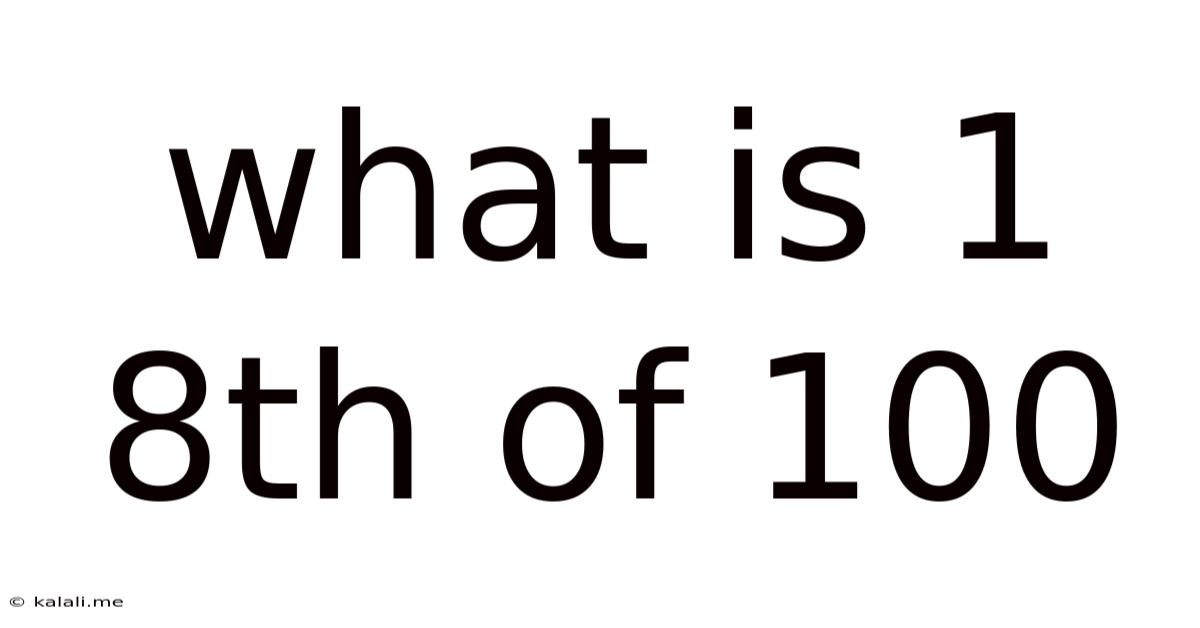
Table of Contents
What is 1/8th of 100? A Deep Dive into Fractions, Percentages, and Real-World Applications
Finding 1/8th of 100 might seem like a simple arithmetic problem, suitable only for elementary school. However, understanding this seemingly basic calculation opens doors to a broader comprehension of fractions, percentages, decimals, and their practical applications in various aspects of life. This article will not only answer the question directly but also explore the underlying mathematical concepts and demonstrate their relevance in everyday scenarios. We'll delve into different methods for solving the problem, discuss related concepts, and provide real-world examples to solidify your understanding.
Understanding Fractions: The Building Blocks
Before tackling the problem, let's solidify our understanding of fractions. A fraction represents a part of a whole. It's expressed as a ratio of two numbers: the numerator (top number) and the denominator (bottom number). The numerator indicates how many parts we have, while the denominator indicates how many equal parts the whole is divided into. In the case of 1/8, the numerator is 1, and the denominator is 8, meaning we're dealing with one out of eight equal parts.
Calculating 1/8th of 100: The Direct Approach
The most straightforward method to find 1/8th of 100 is to perform a simple multiplication:
1/8 * 100 = 100/8
This gives us an improper fraction (where the numerator is larger than the denominator). To convert this to a mixed number (a whole number and a fraction), we perform a division:
100 ÷ 8 = 12 with a remainder of 4.
Therefore, 100/8 = 12 4/8. This can be further simplified by reducing the fraction 4/8 to its lowest terms: 4/8 = 1/2.
So, the final answer is 12 1/2 or 12.5.
Alternative Methods: Exploring Different Approaches
While the direct multiplication method is efficient, other approaches can help reinforce understanding and offer alternative perspectives:
-
Using Decimals: We can convert the fraction 1/8 to its decimal equivalent. 1/8 = 0.125. Multiplying this by 100: 0.125 * 100 = 12.5. This confirms our previous answer.
-
Finding 1/4 and Halving: We know that 1/8 is half of 1/4. First, find 1/4 of 100 (100/4 = 25). Then, halve this result (25/2 = 12.5). This method showcases the relationship between different fractions.
-
Using Percentages: Since 1/8 is equivalent to 12.5%, we can calculate 12.5% of 100. This can be done by multiplying 100 by 0.125 (12.5% expressed as a decimal) resulting in 12.5. This method highlights the connection between fractions and percentages.
Real-World Applications: Where Fractions Matter
Understanding fractions and percentages isn't just an academic exercise; it's crucial for navigating various real-world situations:
-
Sharing Resources: Imagine sharing 100 cookies among eight friends. Each friend would get 1/8th, or 12.5 cookies. In reality, you'd probably cut some cookies in half to ensure fair distribution.
-
Cooking and Baking: Many recipes call for fractional amounts of ingredients. Knowing how to calculate fractions is essential for accurate measurements and successful cooking. For example, a recipe might call for 1/8th of a cup of sugar.
-
Discount Calculations: Sales often advertise discounts as a percentage or fraction. For instance, a 12.5% discount on a $100 item equates to a savings of $12.50. Understanding this calculation ensures you get the best deals.
-
Financial Management: Understanding fractions and percentages is vital for managing personal finances. Calculating interest rates, understanding loan terms, and analyzing investment returns all require proficiency in these areas. For example, if you invest $100 and get an 8% return, your return would be $8.
-
Data Analysis: In many fields like statistics, data science, and research, interpreting data often involves working with fractions and percentages. Whether it's analyzing survey results, calculating probabilities, or understanding proportions, understanding these mathematical concepts is invaluable.
Expanding Your Knowledge: Beyond the Basics
This seemingly simple problem of finding 1/8th of 100 provides a springboard for exploring more complex concepts:
-
Proportions: The problem can be viewed as a proportion: 1/8 = x/100. Solving for x would give you the same answer: 12.5.
-
Ratio and Rate: The concept of ratio and rate is closely related to fractions. A ratio expresses the relationship between two quantities, while a rate is a ratio that involves time.
-
Algebra: More complex problems involving fractions can be solved using algebraic equations.
-
Geometry: Fractions are used extensively in geometry when dealing with areas and volumes of shapes.
Conclusion: Mastering Fractions for a Brighter Future
While the answer to "What is 1/8th of 100?" is 12.5, the true value lies in the deeper understanding gained through exploring the underlying mathematical concepts. The ability to work confidently with fractions and percentages isn't just beneficial for passing math tests; it's an essential life skill that applies across various fields, from everyday tasks to complex calculations. By grasping these fundamentals, you equip yourself with the tools to tackle more complex problems and make informed decisions in various aspects of your life. Continuously practicing and applying these concepts will solidify your understanding and enhance your problem-solving capabilities. Remember, the seemingly simple often holds the key to unlocking more profound knowledge.
Latest Posts
Latest Posts
-
How Much Is 200 G In Pounds
Apr 25, 2025
-
What Is 1 125 In A Fraction
Apr 25, 2025
-
What Is 91 Inches In Feet
Apr 25, 2025
-
What Percent Of 70 Is 28
Apr 25, 2025
-
How Long Is 1k In Miles
Apr 25, 2025
Related Post
Thank you for visiting our website which covers about What Is 1 8th Of 100 . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.