What Is 2 Out Of 3 As A Percentage
Kalali
Apr 14, 2025 · 5 min read
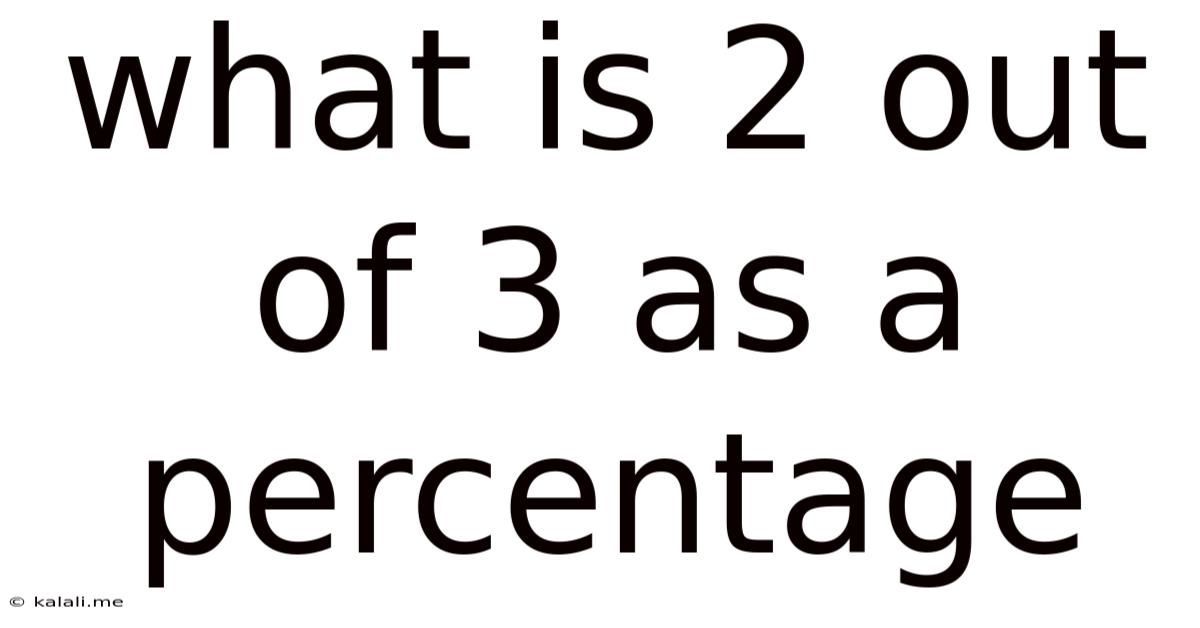
Table of Contents
What is 2 out of 3 as a Percentage? A Comprehensive Guide to Fractions, Decimals, and Percentages
Understanding fractions, decimals, and percentages is fundamental to everyday life, from calculating discounts and taxes to understanding statistics and probability. This article dives deep into the question: "What is 2 out of 3 as a percentage?" We'll not only answer this specific question but also explore the broader concepts involved, providing you with a solid foundation in these essential mathematical concepts. This guide includes practical examples and various methods to solve similar problems, making it an invaluable resource for students, professionals, and anyone looking to improve their mathematical skills.
Meta Description: Learn how to convert fractions to percentages with a detailed explanation of how to calculate 2 out of 3 as a percentage. This guide covers various methods and provides practical examples for understanding fractions, decimals, and percentages.
Understanding Fractions
A fraction represents a part of a whole. It's expressed as a ratio of two numbers: the numerator (the top number) and the denominator (the bottom number). The numerator indicates the number of parts you have, while the denominator indicates the total number of parts the whole is divided into. In our case, "2 out of 3" is represented as the fraction 2/3. The numerator is 2, representing the number of parts we're considering, and the denominator is 3, representing the total number of equal parts.
Fractions can be:
- Proper Fractions: The numerator is smaller than the denominator (e.g., 2/3, 1/4).
- Improper Fractions: The numerator is greater than or equal to the denominator (e.g., 5/4, 7/7).
- Mixed Numbers: A combination of a whole number and a proper fraction (e.g., 1 1/2).
Converting Fractions to Decimals
Before converting a fraction to a percentage, it's often helpful to express it as a decimal. To convert a fraction to a decimal, simply divide the numerator by the denominator.
In our example, 2/3:
2 ÷ 3 = 0.666666...
This is a recurring decimal, meaning the digit 6 repeats infinitely. For practical purposes, we can round this to a certain number of decimal places, such as 0.67. The level of precision required depends on the context. For most everyday calculations, rounding to two decimal places is sufficient.
Converting Decimals to Percentages
A percentage is a way of expressing a number as a fraction of 100. To convert a decimal to a percentage, multiply the decimal by 100 and add the percent symbol (%).
In our example, using the rounded decimal 0.67:
0.67 x 100 = 67%
Therefore, 2 out of 3 is approximately 67%. Remember that this is an approximation due to rounding the recurring decimal.
Calculating 2 out of 3 as a Percentage: The Direct Method
We can also calculate the percentage directly from the fraction without converting to a decimal first. The key is to understand that a percentage is a fraction with a denominator of 100.
To convert 2/3 to a percentage, we need to find an equivalent fraction with a denominator of 100. We can do this by finding a number that, when multiplied by 3, gives 100. Unfortunately, there isn't a whole number that satisfies this condition. This is why we often resort to the decimal conversion method. However, we can still solve this using proportions.
We can set up a proportion:
2/3 = x/100
To solve for x (the percentage), we cross-multiply:
3x = 200
x = 200/3
x ≈ 66.67%
This method confirms our previous result, showing that 2 out of 3 is approximately 66.67%.
Practical Applications and Examples
Understanding the conversion between fractions, decimals, and percentages is crucial in many real-world scenarios. Here are a few examples:
-
Discounts: A store offers a 2/3 discount on an item. This means you'll pay only 1/3 of the original price. Converting 1/3 to a percentage (approximately 33.33%) helps you quickly calculate the final price.
-
Test Scores: If you answered 2 out of 3 questions correctly on a quiz, your score is approximately 66.67%.
-
Surveys and Statistics: If a survey shows that 2 out of 3 people prefer a particular product, this translates to approximately 67% preference.
-
Financial Calculations: Calculating interest rates, loan repayments, and investment returns often involves working with fractions, decimals, and percentages.
-
Probability: In probability, the likelihood of an event occurring is often expressed as a fraction, which can then be converted to a percentage for easier interpretation.
Advanced Concepts and Further Exploration
While this article focused on the basic conversion of 2/3 to a percentage, several advanced concepts build upon this foundation. These include:
-
Working with more complex fractions: Understanding how to convert fractions with larger numerators and denominators, or fractions involving variables, is crucial for more advanced mathematics.
-
Understanding recurring decimals: Learning to handle recurring decimals accurately and efficiently is essential for precise calculations.
-
Percentage change: Calculating percentage increases and decreases is a common application of percentage calculations. For example, if a quantity increases from 3 to 5, the percentage increase is calculated as [(5-3)/3] * 100% = 66.67%.
-
Compound interest: This involves calculating interest on both the principal amount and accumulated interest, leading to exponential growth.
-
Statistical analysis: Percentages are fundamental to interpreting data in statistics, used in calculations of mean, median, mode and standard deviation.
Conclusion
Converting 2 out of 3 to a percentage, resulting in approximately 67%, is a straightforward yet essential skill. This article has provided a comprehensive guide, outlining different methods for conversion and highlighting the broader applications of these fundamental mathematical concepts. By mastering the relationship between fractions, decimals, and percentages, you'll equip yourself with valuable tools for tackling various mathematical problems in your personal and professional life. Remember to practice regularly, working through different examples and problems to solidify your understanding. The more you practice, the more confident and proficient you'll become in handling these essential mathematical concepts. This understanding forms a strong base for tackling more complex mathematical problems and strengthens your overall numerical literacy.
Latest Posts
Latest Posts
-
What Is 68 Centimeters In Inches
Apr 15, 2025
-
What Is 20 Percent Of 4000
Apr 15, 2025
-
Is Luster A Physical Or Chemical Change
Apr 15, 2025
-
Inch And 3 8 To Mm
Apr 15, 2025
-
How Much Is 300ml In Ounces
Apr 15, 2025
Related Post
Thank you for visiting our website which covers about What Is 2 Out Of 3 As A Percentage . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.