What Is 2 Out Of 6 As A Percentage
Kalali
Apr 15, 2025 · 5 min read
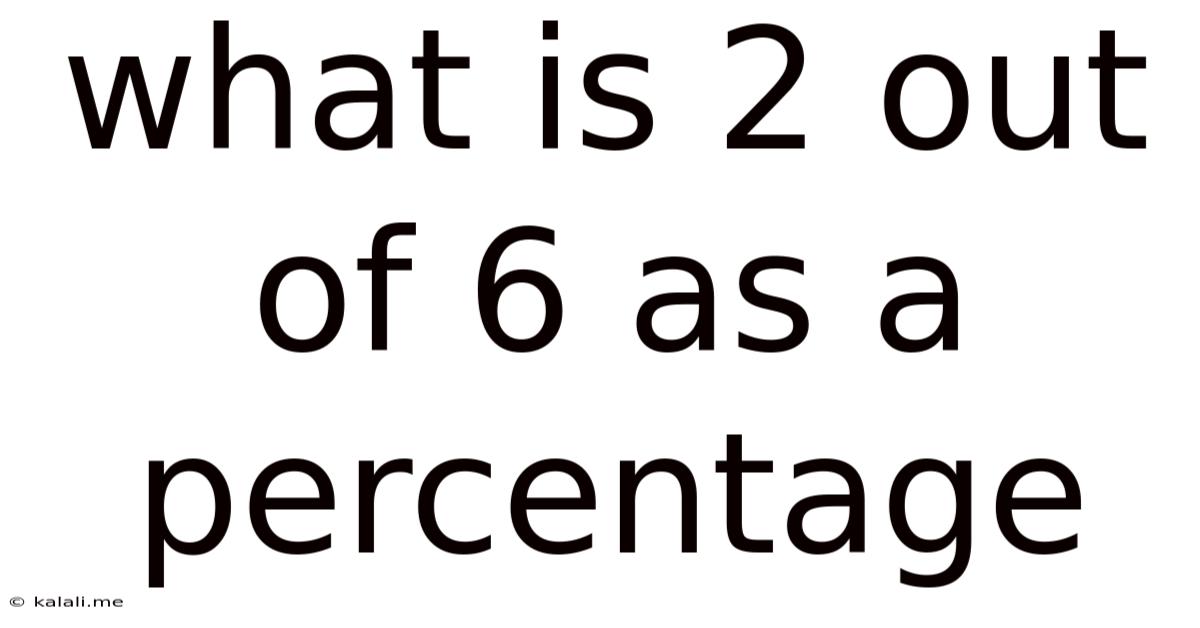
Table of Contents
What is 2 out of 6 as a Percentage? A Comprehensive Guide to Percentage Calculations
Understanding percentages is a fundamental skill applicable across numerous areas of life, from calculating discounts and tax to assessing performance metrics and understanding financial reports. This comprehensive guide will not only answer the question, "What is 2 out of 6 as a percentage?" but also equip you with the knowledge and tools to confidently tackle similar percentage calculations. We'll explore various methods, provide practical examples, and delve into the underlying concepts to solidify your understanding. This article will cover fraction to percentage conversion, practical applications, and troubleshooting common errors.
Meta Description: Learn how to calculate percentages easily! This in-depth guide explains how to determine what 2 out of 6 represents as a percentage, covering multiple methods and real-world applications. Master percentage calculations today!
Understanding Fractions and Percentages
Before diving into the calculation, let's establish the fundamental relationship between fractions and percentages. A percentage is simply a fraction expressed as a portion of 100. The term "percent" literally means "out of 100" (per cent). Therefore, to convert a fraction to a percentage, we need to express the fraction with a denominator of 100.
The fraction "2 out of 6" can be written as 2/6. This represents the ratio of 2 parts to a total of 6 parts. Our goal is to convert this fraction into an equivalent fraction with a denominator of 100, which will directly represent the percentage.
Method 1: Direct Conversion using a Calculator
The simplest approach, especially for more complex fractions, involves using a calculator.
-
Divide the numerator by the denominator: Divide 2 by 6. This gives you 0.3333... (a repeating decimal).
-
Multiply by 100: Multiply the result (0.3333...) by 100. This yields 33.333...
-
Express as a percentage: Therefore, 2 out of 6 is approximately 33.33%. You can round this to a desired level of precision (e.g., 33.3% or 33%).
Method 2: Simplifying the Fraction First
Before converting to a percentage, simplifying the fraction can make the calculation easier.
-
Find the greatest common divisor (GCD): The GCD of 2 and 6 is 2.
-
Simplify the fraction: Divide both the numerator and the denominator by the GCD: 2/6 simplifies to 1/3.
-
Convert to a percentage: Now, we need to find an equivalent fraction with a denominator of 100. To do this, we can set up a proportion:
1/3 = x/100
Solving for x: x = (1 * 100) / 3 = 33.333...
Therefore, 1/3, and consequently 2/6, is approximately 33.33%.
Method 3: Using Proportions
This method provides a more conceptual understanding of the conversion process.
-
Set up a proportion: We know that 2 out of 6 is a part of the whole. We can represent this using a proportion:
2/6 = x/100
-
Cross-multiply: Cross-multiply to solve for x:
6x = 200
-
Solve for x: Divide both sides by 6:
x = 200/6 = 33.333...
-
Express as a percentage: Therefore, 2 out of 6 is approximately 33.33%.
Practical Applications of Percentage Calculations
Understanding percentage calculations is crucial in a wide array of real-world scenarios:
-
Financial calculations: Calculating interest rates, discounts, taxes, profit margins, and investment returns all rely heavily on percentage calculations. For example, a 10% discount on a $100 item means a $10 reduction.
-
Academic performance: Grading systems often use percentages to represent a student's performance on tests, assignments, and overall course grades. A score of 85% indicates that a student answered 85 out of 100 questions correctly.
-
Data analysis: Percentages are frequently used to present data in a clear and concise manner. For instance, market research reports might show that 60% of consumers prefer a particular product.
-
Sports statistics: Batting averages, free throw percentages, and win percentages in sports are all expressed as percentages. A player with a batting average of .300 has a success rate of 30%.
-
Cooking and baking: Recipes often specify ingredients as percentages of the total weight or volume, allowing for easy scaling of recipes.
-
Scientific measurements: Percentage error, percentage change, and percentage yield are commonly used in scientific experiments to quantify results and assess accuracy.
Common Errors to Avoid
While percentage calculations are relatively straightforward, some common errors can lead to inaccurate results:
-
Incorrect order of operations: When dealing with multiple operations (addition, subtraction, multiplication, division), ensure you follow the correct order of operations (PEMDAS/BODMAS).
-
Rounding errors: Rounding numbers prematurely can introduce significant errors, especially in complex calculations. It's best to round only at the final step.
-
Confusing numerator and denominator: Ensure you correctly identify the numerator (the part) and the denominator (the whole) when setting up the fraction.
-
Misinterpreting the question: Carefully read the problem statement to accurately determine what is being asked for. Pay close attention to the wording to avoid misinterpreting the context.
Beyond the Basics: Advanced Percentage Calculations
While this article focuses on the basic calculation of "2 out of 6 as a percentage," mastering percentages involves more than just simple conversions. Further exploration might include:
-
Percentage increase and decrease: Calculating the percentage change between two values.
-
Percentage points: Understanding the difference between a percentage change and percentage points.
-
Compound interest: Calculating interest that accrues on both the principal amount and accumulated interest.
Conclusion
Calculating percentages is a valuable skill with widespread applications. Understanding the different methods for converting fractions to percentages, along with awareness of potential errors, will equip you to confidently handle various percentage-related problems. By mastering these fundamental techniques, you'll be well-prepared to tackle a broad range of quantitative challenges in your personal and professional life. Remember that 2 out of 6 is approximately 33.33%, and you now have multiple methods to arrive at this answer confidently and accurately. Practice applying these methods to different scenarios to reinforce your understanding.
Latest Posts
Latest Posts
-
How Much Is 187 Ml In Ounces
Apr 18, 2025
-
Cuanto Es 5 6 Pies En Metros
Apr 18, 2025
-
How Many Inches Are In 180 Cm
Apr 18, 2025
-
What Is 11 25 As A Percent
Apr 18, 2025
-
How Much Feet Is 172 Cm
Apr 18, 2025
Related Post
Thank you for visiting our website which covers about What Is 2 Out Of 6 As A Percentage . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.