What Is 20 Percent Of 32
Kalali
Apr 13, 2025 · 5 min read
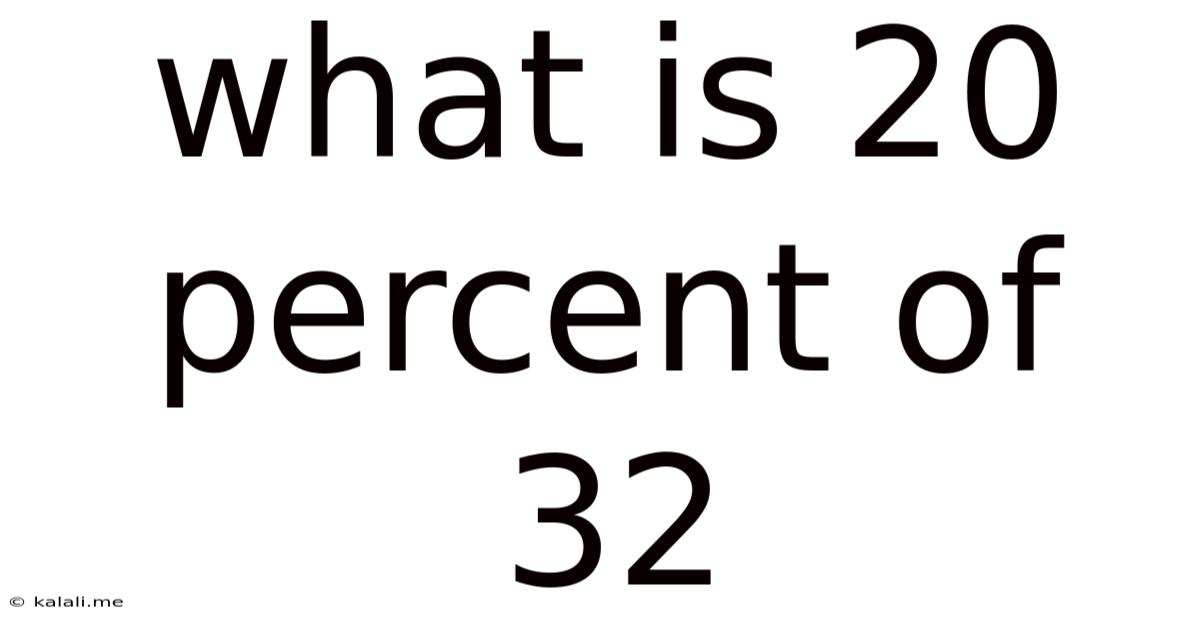
Table of Contents
What is 20 Percent of 32? A Deep Dive into Percentages and Their Applications
This seemingly simple question, "What is 20 percent of 32?", opens the door to a fascinating exploration of percentages, their practical applications, and the underlying mathematical principles. While the answer itself is readily calculable, understanding the how and why behind the calculation is far more valuable. This article will not only provide the solution but delve into the various methods for solving percentage problems, exploring their relevance in diverse fields, from everyday budgeting to complex financial modeling. We'll also touch upon the importance of percentage calculations in data analysis and interpretation, showcasing their power as a fundamental tool for understanding the world around us.
Meta Description: Learn how to calculate 20% of 32 and master percentage calculations. This comprehensive guide explores various methods, real-world applications, and the underlying mathematical principles of percentages. Discover how percentages are used in finance, data analysis, and everyday life.
Understanding Percentages: The Basics
A percentage is a fraction or ratio expressed as a number out of 100. The symbol "%" is used to denote percentages. Essentially, a percentage represents a proportion of a whole. For example, 50% means 50 out of 100, or one-half. Understanding this fundamental concept is crucial for tackling any percentage problem.
Calculating 20% of 32: Three Methods
There are several ways to calculate 20% of 32. Let's explore three common methods:
Method 1: Using the Decimal Equivalent
This is perhaps the most straightforward method. We convert the percentage to its decimal equivalent by dividing by 100. 20% becomes 0.20 (or simply 0.2). Then, we multiply this decimal by the number we're finding the percentage of:
0.20 x 32 = 6.4
Therefore, 20% of 32 is 6.4.
Method 2: Using Fractions
Percentages can also be expressed as fractions. 20% is equivalent to 20/100, which simplifies to 1/5. We can then multiply this fraction by 32:
(1/5) x 32 = 32/5 = 6.4
Again, we arrive at the answer: 6.4.
Method 3: Proportion Method
This method involves setting up a proportion. We know that 20% is to 100% as x (the unknown value) is to 32. This can be written as:
20/100 = x/32
To solve for x, we cross-multiply:
20 x 32 = 100 x x
640 = 100x
x = 640/100 = 6.4
The result, once more, is 6.4.
Real-World Applications of Percentage Calculations
The ability to calculate percentages is a crucial skill applicable across numerous contexts:
1. Finance and Budgeting:
- Calculating discounts: Determining the final price after a 20% discount on a $32 item.
- Understanding interest rates: Calculating the interest earned on savings accounts or the interest paid on loans.
- Analyzing financial statements: Interpreting profit margins, return on investment (ROI), and other key financial metrics expressed as percentages.
- Tax calculations: Determining the amount of sales tax or income tax owed.
2. Data Analysis and Interpretation:
- Presenting data visually: Representing data using pie charts, bar graphs, and other visualizations that utilize percentages.
- Analyzing survey results: Interpreting survey data where responses are often expressed as percentages.
- Understanding statistical significance: Evaluating the significance of research findings often involves percentage calculations.
- Calculating growth rates: Determining the percentage increase or decrease in sales, population, or other metrics over time.
3. Everyday Life:
- Calculating tips: Determining the appropriate tip amount in a restaurant.
- Understanding sales and promotions: Making informed decisions based on advertised discounts.
- Comparing prices: Determining the best value for money when comparing products with different prices and quantities.
- Cooking and baking: Adjusting recipes based on percentage changes in ingredient quantities.
Advanced Percentage Calculations and Concepts
While calculating 20% of 32 is relatively straightforward, more complex scenarios may involve:
- Calculating percentage change: Determining the percentage increase or decrease between two values. For example, finding the percentage increase in sales from one year to the next. The formula for percentage change is: [(New Value - Old Value) / Old Value] x 100.
- Finding the original value: If a value has been increased or decreased by a certain percentage, calculating the original value requires reversing the percentage calculation.
- Compound interest: Calculating interest earned on both the principal amount and accumulated interest. This is a more complex calculation involving exponential growth.
- Percentage points vs. percentage change: It's crucial to distinguish between these two concepts. A change from 10% to 20% is a 10 percentage point increase, but a 100% percentage change.
The Importance of Accuracy and Precision
Accuracy in percentage calculations is paramount, especially in financial and scientific contexts. Errors in calculations can have significant consequences. Using the correct methods and double-checking your work is crucial to ensure accuracy. Understanding significant figures and rounding rules also contributes to precise results.
Beyond the Basics: Using Technology for Percentage Calculations
While manual calculations are beneficial for understanding the underlying principles, technology offers tools for efficient and accurate calculations. Spreadsheets (like Microsoft Excel or Google Sheets), calculators, and specialized software can handle complex percentage calculations quickly and accurately. These tools are invaluable for handling large datasets or intricate financial models.
Conclusion: The Ubiquity of Percentages
The seemingly simple question of "What is 20 percent of 32?" has led us on a journey through the world of percentages. From basic calculations to advanced applications, we’ve seen the importance of percentages in various fields. Mastering percentage calculations is not just about finding numerical answers; it's about developing a fundamental understanding of ratios, proportions, and their relevance in interpreting data and making informed decisions in diverse aspects of life. The ability to understand and apply percentages is a valuable skill that enhances analytical thinking and problem-solving capabilities. So, next time you encounter a percentage problem, remember the methods discussed here and apply your newfound knowledge with confidence.
Latest Posts
Latest Posts
-
How Old Am I If Born In 1986
Jul 06, 2025
-
Where Is Food Coloring In The Grocery Store
Jul 06, 2025
-
How Do You Say Pickles In Spanish
Jul 06, 2025
-
How Much Is 1000 Hours In Days
Jul 06, 2025
-
Sic A Parrot On The Guild Emissary
Jul 06, 2025
Related Post
Thank you for visiting our website which covers about What Is 20 Percent Of 32 . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.