What Is 23/25 As A Percent
Kalali
Apr 15, 2025 · 5 min read
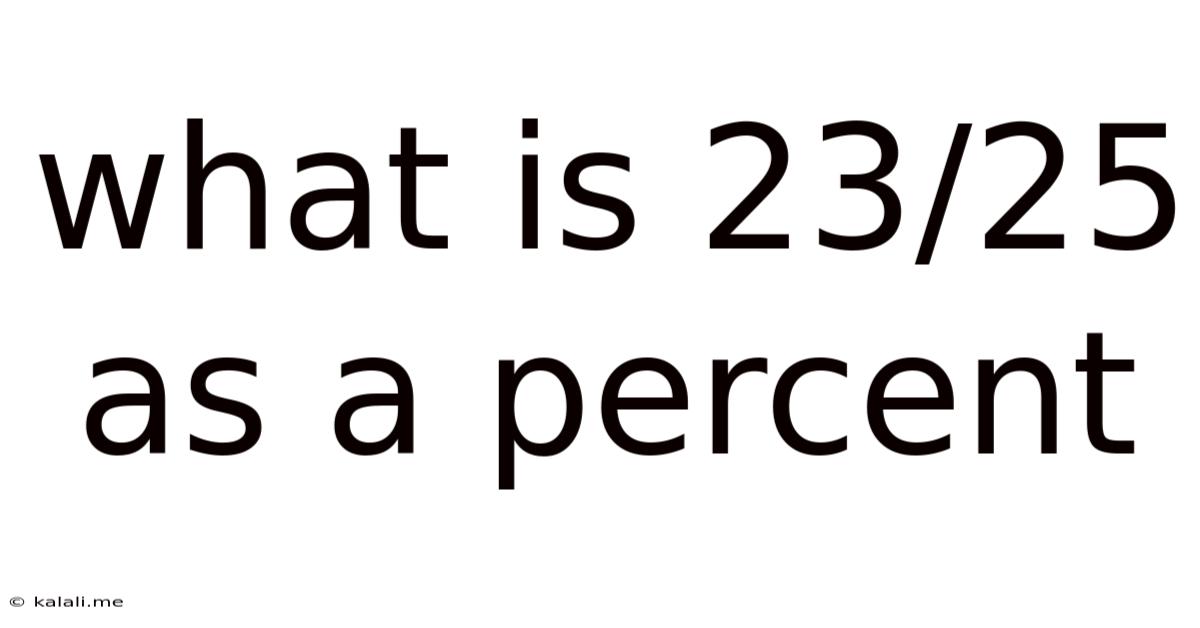
Table of Contents
What is 23/25 as a Percent? A Comprehensive Guide to Fraction-to-Percentage Conversion
This article will delve into the process of converting the fraction 23/25 into a percentage. We'll explore the fundamental concepts behind percentage calculations, providing a clear and comprehensive understanding suitable for various levels of mathematical proficiency. This isn't just about finding the answer; we'll also explore different methods, practical applications, and related percentage problems. Understanding fraction-to-percentage conversions is crucial in numerous real-world scenarios, from calculating grades and discounts to understanding financial data and statistical analyses.
Meta Description: Learn how to convert the fraction 23/25 into a percentage. This comprehensive guide explains the process step-by-step, covering multiple methods and real-world applications of percentage calculations.
Understanding Fractions and Percentages
Before diving into the specific conversion of 23/25, let's refresh our understanding of fractions and percentages.
A fraction represents a part of a whole. It's expressed as a ratio of two numbers: the numerator (top number) and the denominator (bottom number). The denominator indicates the total number of parts, while the numerator shows how many of those parts are being considered. For example, in the fraction 23/25, 23 represents the part, and 25 represents the whole.
A percentage, denoted by the symbol %, represents a fraction out of 100. It expresses a proportion relative to a whole, which is considered as 100 units. So, 50% means 50 out of 100, or 50/100, which simplifies to 1/2.
Method 1: Direct Conversion using Decimal
This is arguably the most straightforward method. We first convert the fraction to a decimal, then multiply by 100 to obtain the percentage.
-
Convert the fraction to a decimal: Divide the numerator (23) by the denominator (25). 23 ÷ 25 = 0.92
-
Multiply the decimal by 100: This converts the decimal to a percentage. 0.92 x 100 = 92
Therefore, 23/25 is equal to 92%.
Method 2: Finding an Equivalent Fraction with a Denominator of 100
This method involves finding an equivalent fraction where the denominator is 100. This directly gives us the percentage since percentages are fractions out of 100.
To achieve this, we need to find a number that, when multiplied by 25, results in 100. That number is 4 (because 25 x 4 = 100). Crucially, whatever we multiply the denominator by, we must also multiply the numerator by the same number to maintain the fraction's value.
-
Multiply both the numerator and denominator by 4: (23 x 4) / (25 x 4) = 92/100
-
Express as a percentage: Since the denominator is 100, the numerator directly represents the percentage. 92/100 = 92%
This method clearly demonstrates the relationship between fractions and percentages.
Method 3: Using Proportions
This method uses the concept of proportions to solve for the unknown percentage. We set up a proportion where one ratio represents the fraction (23/25) and the other represents the unknown percentage (x/100).
-
Set up the proportion: 23/25 = x/100
-
Cross-multiply: 23 * 100 = 25 * x 2300 = 25x
-
Solve for x: x = 2300 / 25 x = 92
Therefore, x = 92%, confirming that 23/25 is equal to 92%.
Practical Applications of Percentage Conversions
The ability to convert fractions to percentages has widespread practical applications in various fields:
-
Academic Performance: Calculating grades based on the number of correct answers out of the total questions. For example, if a student answers 23 out of 25 questions correctly, their percentage score is 92%.
-
Discounts and Sales: Determining the discount percentage offered on a product. A 23/25 discount means a 92% discount.
-
Financial Calculations: Calculating interest rates, profit margins, tax rates, and investment returns. Understanding percentage changes is crucial for financial analysis and decision-making.
-
Statistical Analysis: Representing data as percentages to make comparisons and draw conclusions. Percentages provide a standardized way to represent proportions in data analysis.
-
Everyday Life: Calculating tips in restaurants, determining the percentage of ingredients in a recipe, understanding survey results. Percentages are ubiquitous in daily life.
More Complex Percentage Problems
While the conversion of 23/25 to a percentage is relatively straightforward, let's consider some more complex scenarios that build upon this fundamental understanding:
Scenario 1: Finding the original value:
Let's say a product is on sale for $76 after a 23/25 discount. What was the original price?
-
The discount is 92%, meaning the sale price represents 8% (100% - 92%) of the original price.
-
Let 'x' be the original price. Then 0.08x = $76.
-
Solving for x, we get x = $76 / 0.08 = $950.
The original price was $950.
Scenario 2: Percentage Increase/Decrease:
Suppose a quantity increases from 25 to 46. What is the percentage increase?
-
Find the difference: 46 - 25 = 21
-
Calculate the percentage increase relative to the original value: (21/25) * 100% = 84%
The quantity increased by 84%.
Scenario 3: Combining Percentages:
Imagine a 10% discount followed by a further 20% discount. This isn't simply 30%. Let's say the original price is $100:
-
10% discount: $100 - ($100 * 0.10) = $90
-
20% discount on the reduced price: $90 - ($90 * 0.20) = $72
The final price is $72, representing a 28% discount (100-72 = 28). This demonstrates that percentages don't always add linearly.
Conclusion
Converting 23/25 to a percentage, resulting in 92%, is a fundamental skill with extensive real-world applications. Understanding the different methods – direct conversion, equivalent fractions, and proportions – allows for flexibility in approaching percentage problems. By mastering these techniques, you'll be well-equipped to handle various percentage calculations, from simple conversions to more complex scenarios involving discounts, increases, and combined percentages. The ability to confidently work with percentages is a valuable asset in numerous aspects of life, from academic pursuits to professional endeavors and personal finance. Remember to practice regularly to solidify your understanding and build confidence in tackling diverse percentage problems.
Latest Posts
Latest Posts
-
How Many Liters Is 30 Oz
Apr 18, 2025
-
How Many Cups Is In 16 9 Fl Oz Of Water
Apr 18, 2025
-
Cuanto Es Una Pulgada En Milimetros
Apr 18, 2025
-
What Is 12 16 As A Percent
Apr 18, 2025
-
What Things Are Recycled During Photosynthesis And Respiration
Apr 18, 2025
Related Post
Thank you for visiting our website which covers about What Is 23/25 As A Percent . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.